PDF (3.584 MB)

13 Pages·2012·3.58 MB·English

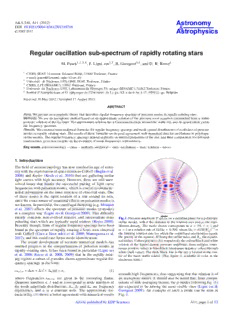
A&A546,A11(2012) Astronomy
DOI:10.1051/0004-6361/201219716 &
(cid:2)c ESO2012 Astrophysics
Regular oscillation sub-spectrum of rapidly rotating stars
M.Pasek1,2,3,4,F.Lignières1,2,B.Georgeot3,4,andD.R.Reese5
1 CNRS,IRAP,14avenueEdouardBelin,31400Toulouse,France
e-mail:[email protected]
2 UniversitédeToulouse,UPS-OMP,IRAP,Toulouse,France
3 CNRS,LPT(IRSAMC),31062Toulouse,France
4 UniversitédeToulouse,UPS,LaboratoiredePhysiqueThéorique(IRSAMC),31062Toulouse,France
5 Institutd’AstrophysiqueetGéophysiquedel’UniversitédeLiège,Alléedu6Août17,4000Liège,Belgium
Received30May2012/Accepted17August2012
ABSTRACT
Aims.Wepresentanasymptotictheorythatdescribesregularfrequencyspacingsofpressuremodesinrapidlyrotatingstars.
Methods.Weuseanasymptoticmethodbasedonanapproximatesolutionofthepressurewaveequationconstructedfromastable
periodicsolutionoftheraylimit.TheapproximatesolutionhasaGaussianenvelopearoundthestableray,anditsquantizationyields
thefrequencyspectrum.
Results.Weconstructsemi-analyticalformulasforregularfrequencyspacingsandmodespatialdistributionsofasubclassofpressure
modesinrapidlyrotatingstars.Theresultsoftheseformulasareingoodagreementwithnumericaldataforoscillationsinpolytropic
stellarmodels.Theregularfrequencyspacingsdependexplicitlyoninternalpropertiesofthestar,andtheircomputationfordifferent
rotationratesgivesnewinsightsontheevolutionofmodefrequencieswithrotation.
Keywords.asteroseismology–chaos–methods:analytical–stars:oscillations–stars:rotation–waves
1. Introduction
Thefieldofasteroseismologyhasnowreacheditsageofmatu-
ritywiththeexploitationofspacemissionsCoRoT(Baglinetal.
2006) and Kepler (Koch et al. 2010) that are gathering stellar
light curves with high accuracy. However, there are still unre-
solved issues that hinder the successful pairing of light curve
frequencieswithpulsationmodes,whichiscrucialtoobtainde-
tailedinformationontheinnerstructureofobservedstars. One
of these issues is the rapid rotation of a star around its axis,
sincetheexactnatureofrotationaleffectsonpulsationmodesis
notknown.Inparticular,thecentrifugalflattening(e.g.Monnier
et al. 2007) affects the spectrum of pressure modes (p-modes)
ina complexway (Lignières&Georgeot2009).Thisdifficulty
(cid:2)
mainly concerns non-evolved massive and intermediate-mass Fig.1.PressureamplitudeP d/ρ onameridianplaneforapolytropic
0
pulsatingstarswhich are typicallyrapidrotators(Royer2009). stellarmodel,withd thedistancetotherotationaxisandρ theequi-
0
Recentlythough,hintsofregularfrequencyspacingshavebeen librium density. The mode shown corresponds to n = 50, (cid:4) = 1 and
foundinthespectrumofrapidlyrotatingδScutistarsobserved m=1atarotationrateofΩ/Ω =0.300,whereΩ =(GM/R3 )1/2is
K K eq
with CoRoT (García Hernández et al. 2009; Mantegazza et al. thelimitingrotationrateforwhichthecentrifugal accelerationequals
2012),andthiscouldeasefuturemodeidentification. thegravityattheequator, MbeingthestellarmassandReqtheequato-
rialradius.Colors/grayness(forrespectivelythecolour/blackandwhite
The recent development of accurate numerical models has
versionofthefigure)denotepressureamplitude, fromred/gray(max-
enabled progress in the comprehension of pulsation modes in
imumpositivevalue)toblue/black(minimumnegativevalue)through
rapidlyrotatingstars.Ithasbeenfoundinparticular(Lignières white(nullvalue).Thethickblacklineistherayγlocatedinthecen-
et al. 2006; Reese et al. 2008, 2009) that in the rapidly rotat-
ter of the main stable island. (This figure is available in color in the
ingregimeasubsetofp-modesshowsapproximateregularfre- electronicform.)
quencyspacingsintheform:
ωn,(cid:4),m (cid:3)Δnn+Δ(cid:4)(cid:4)+Δm|m|+α, (1)
towardshigh-frequencies,thussuggestingthatthisrelationisof
where frequencies ωn,(cid:4),m are given in the corotating frame. anasymptoticnature.Itshouldalsobenotedthat,fromcompu-
Quantum numbers n, (cid:4) and m correspond to node numbers of tationsofdisk-averagingfactors,thep-modesfollowingEq.(1)
the modeamplitudedistributions,Δn, Δ(cid:4) and Δm are frequency are expected to be among the most visible ones (Lignières &
regularities, and α is a constant term. The approximate for- Georgeot 2009). An example of such a mode can be seen in
mulainEq.(1)showsabetteragreementwithnumericalresults Fig.1.
ArticlepublishedbyEDPSciences A11,page1of13
A&A546,A11(2012)
ThefrequencyspacingsofEq.(1)arenotablysimilartothe profiles)andenergies(frequencies)ofthequantum(wave)sys-
regularitiesdescribedbyTassoul’sasymptoticformula(Tassoul tem from the different structures that are present in the classi-
1980) for low degree p-modes in non-rotating stars. Tassoul’s calsystemphasespace (Percival1973;Berry&Robnik1984).
formulaatleadingorderis In the stellar pulsation setting, Lignières & Georgeot (2008,
(cid:3) (cid:4) 2009) found that the mixed (i.e. regular and chaotic) charac-
(cid:4) 1 ter of the acoustic ray dynamics in rapidly rotating stars re-
ωn,(cid:4) (cid:3)Δ ns+ s + +αs , (2) sults in a classification of p-modes in two broad families: reg-
2 4
ular modes either associated with stable islands or whispering
gallery zones, and chaotic modes associated with ergodic re-
withthelargefrequencyseparation
gionsinphasespace.Fortheregularmodesassociatedwithsta-
(cid:3) (cid:5) R dr (cid:4)−1 ble islands, the so-called island modes, it is known to be pos-
Δ=2π 2 , (3) sible to obtain approximate analytical solutions by solving the
c(r) waveequationinthevicinityofaperiodicstableray(Babich&
0
Buldyrev1991).Asimpleapplicationofsuchamethodisfound
where c(r) is the radially inhomogeneous sound speed, and R in modes of optical resonators, where the periodic stable light
thestellarradius.Theintegern isthenodenumberoftheradial ray is a straight line between two reflecting mirrors (Kogelnik
s
component of the mode, while (cid:4) is the degree of the associ- & Li 1966).These methodshave been previouslyemployed to
s
atedsphericalharmonics,andα dependsonsurfaceproperties. obtain modes of more complex lasing (Tureci et al. 2002) and
s
Tassoul’stheoryhasprovedto be veryusefulforinterpretating electronic (Zalipaev et al. 2008) cavities as well as quantum
solar-like oscillations in slowly rotating stars. Indeed, the for- chaossystems (Vagovet al. 2009).In this paper,we applythis
mularelatesobservablequantities,suchastheregularfrequency approachtorapidlyrotatingstars.
spacingΔ,tophysicalpropertiesofstellarinteriors.Forrapidly Inthepresentanalysis,wethusconstructanasymptoticfor-
rotatingstars,itwouldbeclearlydesirabletogaininsightsonthe mulaforregularitiesinthep-modespectrumofrapidlyrotating
underlyingphysicsofthepotentiallyobservableregularspacings stars.Partoftheresultswerealreadypresentedintheshortcom-
Δn, Δ(cid:4) and Δm by a similar asymptotic analysis. In this paper municationofPaseketal.(2011).Inthepresentpaperwegivea
wederiveaformulafortheseregularfrequencyspacingsinthe detailedderivationoftheseresults,specifytheirdomainofvalid-
asymptoticregime. ity,extendthemwithastudyofrotationalsplittings,andexplore
The generalization of the p-mode asymptotic theory to theirastrophysicalapplications.
rapidlyrotatingstarsisnottrivial.Tassoul’stheoryrequiressep- Thepaperisorganizedasfollows.InSect.2wepresentthe
arationofvariables,whichisnolongerpossiblewhenthestaris wave equation for p-modesin rotating stars and its asymptotic
flattened by rotation. For non-separable wave systems, a well- limit leading to an equation for acoustic rays. In Sect. 3 we
known technique to obtain eigenmodes is to study the short- use a stable periodic solution of the ray dynamics to obtain a
wavelength limit of the propagatingwaves. This limit gives an semi-analyticalformula for the associated p-modes, and to de-
equation for the propagationof rays that is similar to the geo- riveaformulafortheassociatedregularfrequencyspacings.We
metricalopticslimit of electromagnetism,or the classical limit thencomparetheresultsobtainedfromthederivedformulasfor
in quantum mechanics. Then, by imposing quantization condi- mode frequencies and spatial distributions with numerical re-
tionson thephaseof wavespropagatingontheserays, oneob- sults (Sect. 4). Finally, we suggest directions on how these re-
tains the eigenmodes of the wave system. This technique was sults could be used for the asteroseismic diagnosis of rapidly
first developedin the contextof quantumphysics, and is often rotating stars by discussing the phenomenologicalimplications
calledsemiclassicalquantization. ofthetheoryinSect.5.
Forsphericalstars,theraylimitofpressurewaveshasbeen
previouslyusedtorecovertheTassoulasymptoticformulafrom
theEinstein-Brillouin-Keller(EBK)quantizationofraydynam- 2. P-modesinrotatingstarsandtheirasymptotic
ics (Gough 1993). This analytical approach is possible only limit
whentheraysystemisintegrable.Adynamicalsystemissaidto
beintegrablewhenithasasmanyconservedquantities(energy, InSect.2.1weintroducethewaveequationforp-modesinrotat-
angular momentum,etc.) as degrees of freedom (Ott 2002). In ing stars. We then presentthe asymptoticlimitof thisequation
rapidlyrotatingstars,therearenotenoughconservedquantities inordertoobtainanequationforthedynamicsofacousticrays
toensureintegrabilityoftheraydynamics.Indeed,inLignières (Sect.2.2).
&Georgeot(2008,2009),ithasbeenfoundthatacousticraysin
rotatingstarshaveaverydifferentdynamicalbehaviordepend-
2.1.Pressuremodesinrotatingstars
ingontheirinitialconditionsinposition-momentumspace(the
so-called phase space). For a polytropic stellar model, the nu- We start with the equation for small adiabatic time-harmonic
mericalintegrationofthe equationsforacoustic raysdisplayed perturbationsofthepressurefieldinaself-gravitatinggas.Since
varioustypes of solutions. Indeed,one can obtain either stable weareinterestedinobtaininganasymptotictheoryforp-modes
raysstayingontorus-shapedsurfacesinphasespacewhichform in the high-frequencyregime, we use the Cowling approxima-
structures such as stable islands, or chaotic rays that are dense tion (i.e. we neglect the perturbations of the gravitational po-
andergodiconaphasespacevolume. tential),anapproximationknowntobevalidforhigh-frequency
Asimilarbehaviorhasbeenfoundinmanysystemsstudied perturbationsin non-rotatingstars (Aerts et al. 2010). We also
in the field of theoretical physics known as quantum chaos or neglecttheCoriolisforce.Indeed,inthehigh-frequencyregime,
wave chaos (Gutzwiller 1990). This field has among its objec- the time scale associated with this force is much longer than
tivestoanalyzequantum(wave)systemswhoseclassical(short- the mode period, and thus the influence of the Coriolis force
wavelength) limit is partly or fully chaotic. In this framework, on pulsation frequencies is weak. This has been numerically
one can predict the existence of some eigenfunctions (mode checked in Lignières et al. (2006), Reese et al. (2006, 2008).
A11,page2of13
M.Paseketal.:Regularoscillationsub-spectrumofrapidlyrotatingstars
In the asymptotic regime of p-modes, the oscillation frequen-
cies are far greater than the Brunt-Väisälä frequency and thus
we candiscardthetermscorrespondingtogravitywaves. With
these assumptions, the equation for pressure perturbations is a
Helmholtzequationsuchthat
ω2−ω2
ΔΨ+ cΨ=0, (4)
c2
s
where Ψ = Pˆ/f is the complex amplitude associated with the
pressure perturbation P = Re[Pˆexp(−iωt)], f is a function of
thebackgroundmodel,ω isthecut-offfrequencyofthemodel
c
andc itsinhomogeneoussoundvelocity(foradetailedderiva-
s
tionofthisequationseeLignières&Georgeot2009).Thestellar
model is not spherically symmetric due to the centrifugal dis- Fig.2.PoincaréSurfaceofSection(PSS)attherotationrateΩ/Ω =
K
tortion, but is however cylindrically symmetric with respect to 0.589forquantumnumberm = 0.Eachdotcorrespondstothecross-
the rotation axis. Therefore, we can write the pressure field as ingofanacousticraywiththeequatorialhalf-planeinthe(r/Req,kr/ω)
Ψ = Ψ exp(imφ) where m is an integer and φ is the azimuth phasespace,rbeingtheradialcoordinateandkrtheassociatedmomen-
m tum.R istheequatorial radiusandωthemodefrequency. Red/dark
angle of spherical coordinates. By inserting this expression in eq
gray denotes a chaotic ray, green/light gray a whispering gallery ray,
Eq.(4)weobtain(cf.AppendixA) blue/blackastableislandray(seetext).Upperinsetisaclose-upofthe
⎛ (cid:9) (cid:10)⎞
main stable island. (This figure is available in color in the electronic
ΔΦm+ c12 ⎜⎜⎜⎜⎜⎜⎝ω2−ω2c − c2s md22− 14 ⎟⎟⎟⎟⎟⎟⎠Φm =0, (5) form.)
s
wheredisthedistancetothe√rotationaxis.Thenewmodeampli- To probe the integrability property of a dynamical system,
tudeΦ issuchthatΦ = dΨ .Weintroducearenormalized it is convenient to use the Poincaré surface of section (PSS),
m m m
soundvelocity: a standard tool in dynamicalsystems theory (Gutzwiller 1990;
Ott2002)tovisualizethestructuresinphasespace.APSSisa
c
c˜s = (cid:14) (cid:15) s (cid:16)· (6) lowerdimensionalsliceofphasespace.Theacousticraydynam-
1− 1 ω2+ c2s(m2−14) icalsysteminthemeridionalplanehastwodegreesoffreedom,
ω2 c d2 whichgivesaphasespaceofdimensionfour(twoforpositions,
and two for momenta). There is one conserved quantity in the
We notice that besides its spatial dependence, c˜ also depends
s form of the acoustic wave frequency,so the dynamics belongs
on ω and m, and that m is taken as a parameter for the two-
to a three-dimensional manifold in phase space. By fixing an
dimensionalwaveequation(Eq.(5)).
additionalposition or momentumcoordinate,we obtain a two-
dimensional PSS which can be easily visualized. An example
2.2.Raylimitofp-modes of such a section for our system is shown in Fig. 2. Different
choices of PSS variables are possible, some of which are pre-
Innon-rotatingstars,theasymptotictheoryofhighfrequencyp- sentedinLignières&Georgeot(2009).Wehaveherechosento
modeshasfirstbeenderivedbyVandakurov(1967),andTassoul fix the colatitude θ = π/2, so that the PSS corresponds to the
(1980).Themethodwastousethesphericalsymmetryofthestar crossingofrayswiththeequatorialhalf-plane.Wethusdisplay
toreducetheproblemtoaone-dimensionalequationinorderto asectionincoordinates(r/R ,k /ω)wherek isthenormofthe
eq r r
obtainthemodefrequencies.Thismethodisnotapplicablewhen radialwavevector,ωthemodefrequency,andR theequatorial
eq
the centrifugalforce breaksthe sphericalsymmetryof the star. radius (that may be greater than the polar radius since the star
In this case though, one can study the short-wavelength limit is flattened by rotation).In such plots, each dot correspondsto
(ω→∞)ofthewaveequation(Eq.(4))(asdetailedinLignières the crossing of an acoustic ray with the PSS. Successive dots
&Georgeot2009).ThisprovidesaHamiltoniansystemdescrib- from a single ray will form lines in integrable zones, or fill
ingthepropagationofacousticrays.TheHamiltonianhasbeen surfaces densely in chaotic zones. We see in Fig. 2 that when
derivedinLignières&Georgeot(2009)as the rotation rate Ω/Ω (where Ω = (GM/R3 )1/2 is the limit-
K K eq
(cid:3) (cid:4) ingrotationrate)islarge,differentstructurescoexistinthesys-
H =−k˜2p + 1 1− ω2c − c2sm2 , (7) temphasespace:stableislandscorrespondtoconcentriccircles
2 2c2 ω2 ω2d2 aroundastableray,whisperinggalleryraystolinesnearthesur-
s
face,andchaoticzonestodenselyfilledareas(formoredetails,
where the frequency-scaledwavevector k˜p is the projection of see Lignières & Georgeot 2009). In this paper, we will focus
k˜ = k/ω onto the meridionalplane of the star. We notice that on the 2-periodic stable island which is the main stable island
thisexpressionhasbeenderivedfromtheshort-wavelengthlimit (shownin the inset of Fig. 2). The rays’ dynamicsis verysen-
ofthethreedimensionalwaveequation(Eq.(4))andthen,pro- sitive to the rotation rate, so the PSS will be differentfor each
jectedontothecorotatingmeridianplane.Analternativederiva- rotationalvelocity.Indeed,thelocusinphasespaceofthemain
tionwouldbetostartfromthetwo-dimensionalwaveequation stable island changes as rotation increases. The major change
(Eq. (5)). In this case, the ray limit yields the same expression happenswhenthecentralrayofthe2-periodicstableislandun-
withtheadditionofthe1/4factorofEq.(5)thataccountsforthe dergoesa bifurcationat Ω/Ω (cid:3) 0.26.For slow rotation rates,
K
impossibilityofacousticraystogothroughtherotationaxis(i.e. the central ray of the island is located on the polar axis, and
d =0).ThroughoutthepaperweuseEq.(7)astheHamiltonian through this bifurcation it transforms into two stable rays sur-
foracousticrays. rounding one unstable ray on the polar axis. Then, as rotation
A11,page3of13
A&A546,A11(2012)
increases,thestableislandwillcoastawayfromthepolaraxis. and
This bifurcation will be of some importance in the following, (cid:9) (cid:10)
wmhoedneswoeftwhiellwsahvoewsythstaetmonferocmanthcison2s-tpreurcitoadpicprsotaxbimleaitselaenidg.eInn- 1 = 1 + ∂ 1/c˜s(s,ξ)2 (cid:17)(cid:17)(cid:17)(cid:17) ξ
Fig.1,onecanseeanexampleofanislandmodeobtainedfrom c˜s(s,ξ)2 c˜s(s,0)2 ∂(cid:9)ξ ξ=(cid:10)0
a2-fpuellr-inoudmicesrtiacballecoismlapnudtaftoiornth,etosgaemtheerrowtiatthiotnheracteentarnadlrqauyaonftuthme + 21∂2 1/∂c˜ξs(2s,ξ)2 (cid:17)(cid:17)(cid:17)(cid:17)ξ=0ξ2+O(cid:9)ξ3(cid:10). (16)
numberm.
We then express the function Φ (s,ξ) in terms of a Wentzel-
m
Kramers-Brillouin(WKB)ansatzas
3. Semi-analyticalmethodforislandmodes
In this section we construct an asymptotic approximation of a Φm(s,ξ)=exp(iωτ)Um(s,ξ,ω), (17)
subsetofregularp-modesassociatedwithastableperiodicray.
where τ is an unknown function of position. The fundamental
ThemethodisbasedontheworksofBabichandcoworkers(see
assumptionunderlyingthetheoryofBabichisthat,asω→+∞,
Babich&Buldyrev1991andreferencesthereinforthegeneral
formalism;ande.g.Zalipaevetal.2008;Vagovetal.2009,for themodeislocali√zedontheacousticrayandthatitstransverse
extentscalesas1/ ω.Suchasolutioncanbefoundbyassuming
some applications).It consists in derivingan approximationof
thatthetransversevariableξscalesas
the wave equation in the vicinity of the ray (Sect. 3.1),finding
Gaussianwavepacketsolutionsrelatedtothestabilityproperties (cid:9) √ (cid:10)
ξ =O 1/ ω . (18)
oftheray(Sect.3.2),andthenderivingtheasymptoticformula
forthefrequenciesfromaquantizationcondition(Sect.3.3).
Then,fromanexpansionofEq.(9)inω,oneobtainsatthedom-
inantorderthattheWKBphaseinEq.(17)dependsonlyonsas
3.1.Approximatewaveequationinthevicinityofastableray dτ=ds/c˜ .Atthenextorderinωonefindsaparabolicequation
s
forthefunctionV :
For a given rotation rate and quantum number m, we start m
with the central periodic ray of the main stable island (see ∂2V 2i ∂V
Sect. 2).Thisraymustbe computedby numericallyevaluating ∂ν2m −K(s)ν2Vm+ c˜ (s) ∂sm =0, (19)
the Hamiltonianequationsderivedfrom Eq. (7). In the follow- s
ing we will call this ray γ. The first step is to write the wave with
equation (Eq. (5)) in the vicinity of γ. For this, we use a local (cid:17)
orthonormal coordinate system (s,ξ) defined as r = sT + ξN K(s)= 1 ∂2c˜s(cid:17)(cid:17)(cid:17) , (20)
wheresisthearclengthalongtheray,Ttheunittangentvector, c˜s(s)3 ∂ξ2 ξ=0
ξ the transverse coordinate and N the unit vector normal to T. √
Thetwobasisvectorsarerelatedbythecurvatureκ(s)oftheray wher√eweintroducedthescaledcoordinateν = ωξandVm =
asfollows: Um/ c˜s.
dN
κ(s)=− ·T. (8)
ds 3.2.Solutionsoftheparabolicwaveequation
Inthiscoordinatesystem,thewaveequation(Eq.(5))reads
(cid:3) (cid:3) (cid:4) (cid:3) (cid:4)(cid:4) Tofinda solutiontoEq.(19),wefirstfinda solutionata fixed
1 ∂ hξ∂Φm + ∂ hs ∂Φm + ω2 Φ =0, (9) arclengths,andthenstudyhowthissolutionmustevolvewiths.
hshξ ∂s hs ∂s ∂ξ hξ ∂ξ c˜s(s,ξ)2 m Atfixeds,thefirsttwotermsofEq.(19)correspondtotheequa-
tionforaquantumharmonicoscillatorinthedirection N trans-
wherethescalefactorshsandhξ (Arfken&Weber2005)are versetoγ.Thus,asweknowfromquantummechanics(Cohen-
h2 =(T−ξκ(s)T)2 =(1−ξκ(s))2 (10) Tannoudjietal.1973),asolutionofthisequationisaGaussian
s wavepacket,transversetotheray,thatwewriteas
and (cid:3) (cid:4)
h2ξ = N(s)2 =1. (11) V0 = A(s)exp iΓ(s)ν2 , (21)
m 2
Thisyields
(cid:3) (cid:4) (cid:3) (cid:4) withΓanunknowncomplex-valuedfunction.Tofindthevaria-
ξ∂κ(s) 1 ∂Φm + 1 ∂2Φm tionofthisGaussianwavepacketalongtherayγ,we introduce
∂s h3 ∂s h2 ∂s2 a solutionof this formin the parabolicequation(Eq.(19))and
s (cid:3) s(cid:4) (cid:3) (cid:4)
1 ∂Φ ∂2Φ 1 obtainaRiccatiequationforΓ
−κ(s) m + m +ω2 Φ =0. (12)
hs ∂ξ ∂ξ2 c˜2s m 1 dΓ +Γ2+K =0, (22)
Inthevicinityoftherayγ,thatisforsmallξ,thetermsofEq.(9) c˜ ds
s
aresimplifiedusing:
(cid:9) (cid:10) simpleequationforthefactorA
1
(1−ξκ(s)) ∼1+ξκ(s)+ξ2κ(s)2+O ξ3 , (13) 1 dA = −c˜sΓ. (23)
(cid:9) (cid:10)
1 A ds 2
∼1+2ξκ(s)+3ξ2κ(s)2+O ξ3 , (14)
(1−ξκ(s))2 Inthefollowing,weshowthattheequationforΓisrelatedtothe
1 (cid:9) (cid:10) ray propertiesin the vicinity of γ and can thus be solved from
(1−ξκ(s))3 ∼1+3ξκ(s)+6ξ2κ(s)2+O ξ3 , (15) raydynamicscomputations.First,usingthevariables(z(s),p(s))
A11,page4of13
M.Paseketal.:Regularoscillationsub-spectrumofrapidlyrotatingstars
definedasΓ(s) = 1 1 dz(s) and p(s) = 1 dz(s),Eq.(22)istrans- calledaFloquetphaseorstabilityangle.Hencethetwolinearly
formedintotheHazm(s)icl˜tsondsiansystem: c˜s ds independentsolutionsofEq.(29)canbewrittenintheform:
(cid:3) (cid:4)
dz α
dτ =c˜2sp (24) (z(τ),p(τ))± =exp ±iTγτ u±(τ)u±, (32)
dp
dτ =−c˜2sKz, (25) wherethefunctionsu±(τ)areperiodicwithperiodTγandu±are
independenteigenvectorsofthemonodromymatrixM.
wherethe(time-dependent)Hamiltonianfunctionis
Anexpressionofthemonodromymatrixintermsofsecond
p2 z2 derivativesoftheactionfunctionS canbederived(Bogomolny
H (p,z,τ)=c˜2 +c˜2K , (26) 2006).TheactionfunctionS isdefinedbyatrajectoryfromthe
0 s 2 s 2 positionq to q for a givenenergyor frequencyω (Gutzwiller
i f
τbeingthetimecoordinate.FromEqs.((24),(25)),onecande- 1990).Forourpurposes,theactioniswrittenas
riveanequationforzonlytoobtain (cid:5)
(cid:3) (cid:4) qf 1
S(q,q ,ω)= dσ, (33)
c˜12ddτ c˜12ddτz +Kz=0. (27) i f qi c˜s
s s
where σ is the arclength along a ray nearby γ. If we write the
Equations ((24), (25)) have two independent solutions (z,p)
monodromymatrixas
and(z¯,p¯)thatareconjugatetoeachother.Ofthesetwosolutions,
(cid:3) (cid:4) (cid:3) (cid:4)(cid:3) (cid:4)
only one is physically relevant, i.e. corresponds to a localized z M M z
wavepacket. According to Eq. (21) this happens if Im(Γ) > 0 pf = M11 M12 pi , (34)
for all s, and as shown in AppendixB, the sign of Im(Γ) stays f 21 22 i
constantalongγsince we can express its components from the second derivatives of
theactionfunctionS withthefollowingformulas
1 1
Im(Γ)= · (28)
2|z|2 ∂2S 1 ∂2S M ∂2S M
=− , = 11, = 22, (35)
The variation along γ of the Gaussian wavepacket can now be ∂z∂z M ∂z2 M ∂z2 M
linked to the dynamics of the acoustic rays nearby γ. Indeed, i f 12 i 12 f 12
Im(Γ)hasasimpleexpressionintermsofthecomplexvariable wherethepositionsq andq arewrittenas(s,z)and(s ,z ),z
i f i i f f i
z,asshowninEq.(28).Thisvariable,ontheotherhand,obeys andz beingrespectivelytheinitialandfinaltransversepositions
f
Eq. (27) which can be shown to be the same as the equation of the neighboringray after one period,and the derivativesare
describing the deviation from γ of a ray nearby γ (see deriva- evaluated on the periodic ray. From these expressions, and the
tioninAppendixC).TheHamiltonianinEq.(26)isthusalocal simple formulagivingthe rootsofa seconddegreepolynomial
integrableapproximation,alsoknownasanormalformapprox- (cf. AppendixD), we can obtainan expressionfor the stability
imation(Arnol’d1989),tothefullHamiltonianforacousticrays angleαas
writteninEq.(7). ⎛(cid:2) ⎞
Now, our task is to find the two linearly independentcom- α=arctan⎜⎜⎜⎜⎜⎝ −Tr(M)2+4⎟⎟⎟⎟⎟⎠, (36)
plexconjugatesolutionsofEqs.((24),(25)).Thetermsinthese
Tr(M)
equations depend only on quantities that are evaluated on the
periodic ray γ. Therefore, these equations are periodic in s, or
where
equivalentlyinτ.Equations((24),(25))canthusbewrittenas:
(cid:3) (cid:4) ⎛ ⎞
d (cid:3)z(cid:4) (cid:3)z(cid:4) Tr(M)=− ∂2S −1⎜⎜⎜⎜⎝∂2S + ∂2S⎟⎟⎟⎟⎠· (37)
dτ p =Σ(τ) p , (29) ∂zi∂zf ∂z2i ∂z2f
wherethematrixΣ Wethushaveobtainedasolutionoftheapproximatewaveequa-
(cid:3) (cid:4) tion Eq. (19) in the form of a Gaussian wavepacket(Eq. (21)),
Σ(τ)= 0 c˜2s , (30) whose evolution along the ray γ is given by Eqs. ((29), (30)).
−c˜2K 0 It is possible to find other solutions of Eq. (19) that have a fi-
s
(cid:18) nite number of nodes in the direction transverse to γ. As for
tviemriefiaelsoΣng(τγ+.TThγe)n=ifΣ((zτ(τ),),Tpγ(τ=))isγadc˜sssobleuintigonthoefaEcqo.u(s2ti9c),trsaoveisl tthheesqeusaonltuutmionhsarcmanonbiecoobsctaililnaetdorf(rComohEenq-.T(a2n1n)ouusdinjigetthael.a1n9n7ih3i)-,
(z(τ+Tγ),p(τ+ Tγ)) and the two solutions are related by the lation aˆ and creationoperatoraˆ† that are defined as (Babich &
followinglinearmap Buldyrev1991)
(cid:19) (cid:20) (cid:19) (cid:20)
pz((ττ++TTγγ)) = M pz((ττ)) , (31) aˆ =−iz∂∂ν −pνandaˆ† =−iz¯∂∂ν −p¯ν. (38)
where M is called the monodromy matrix (Cvitanovic´ et al. These operators have the commutation rule [aˆ,aˆ†] = 1, where
2010,and referencestherein). As (z,p) describe ray deviations the commutator is defined as [Aˆ,Bˆ] = AˆBˆ − BˆAˆ. Indeed, from
from γ, the matrix M characterizes the stability of γ. As γ thecommutationrelationofaˆ†withtheoperatorofEq.(19),the
is stable, we know that |Tr(M)| < 2 and that the eigenvalues functions
are of modulus one and complex conjugates of each other i.e.
Λ± = exp(±iα) with α ∈ ]0, π[ (cf. Appendix D), where α is V(cid:4) =(aˆ†)(cid:4)V0, (39)
m m
A11,page5of13
A&A546,A11(2012)
are also solutions of Eq. (19) (Babich & Buldyrev 1991). The
calculationofthesehigher-(cid:4)solutionsthenleadsto
(cid:9) (cid:10)
(cid:3) i (cid:4)(cid:4)(cid:15)z¯(cid:16)(cid:4)/2 (cid:9)(cid:2) (cid:10)exp iΓν2
Vm(cid:4)(s,ν)= √2 z H(cid:4) Im(Γ)ν √z2 , (40)
n
with H(cid:4) the Hermite polynomials of order (cid:4). Finally, we can
writethesolutionsofEq.(5)as
(cid:3) (cid:5) (cid:4)
(cid:2)
ds
Φ(cid:4)(s,ν)= c˜ V(cid:4)(s,ν)exp iω · (41)
m s m c˜
s
3.3.Quantizationconditionandregularfrequencyspacings
Fig.3. Left: schematic representation of the mode labeling/quantum
Thequantizationconditionisbasedonthesingle-valuednessof numbers used for island modes. Right: illustration of the relation be-
thesolutionpresentedinEq.(41),andthusassertsthatthephase tweenthequantumnumbersofsphericalandislandmodes.Thequan-
accumulatedbythefunctionΦ(cid:4) overoneperiodmustbeamul- tumnumber nisthenumber of nodes inthelongitudinal directionof
tiple of 2π. In the following,wme assume withoutloss of gener- theislandmode(alongtherayγ),(cid:4)isthenumberofnodesinthetrans-
verse direction of the mode (transverse to γ) and m is the number of
ality that the eigenvalue which corresponds to the wavepacket
localizationisexp(+iα).ThecontributionoffunctionV(cid:4) tothe azimuthal nodes. ns, (cid:4)s and ms are the quantum numbers of spherical
dynamicalphaseofΦ(cid:4) isobtainedfromEqs.(32)and (m40).We modes. In the right figure, the multiplets of island modes correspond
m todiagonal colored bands, whereas the multipletsof spherical modes
obtainthatthephaseaccumulatedoveroneperiodis
would have the form of vertical bands. The plotted mode on the left
(cid:21)
1 α+2πN corresponds ton = 46, (cid:4) = 1and m = 0.(Thisfigureisavailablein
ωn,l,m ds− r −(α+2πNr)(cid:4)=2πn+π, (42) colorintheelectronicform.)
γ c˜s 2
whereαistheFloquetphasethatisdefinedmodulo2π.Forour andthefunctionszandΓfromitseigenvectors.Itisimportantto
purposes,wemustalsotakeintoaccountthenumberNr ofmul- notethatformeven,onlymodessymmetricwithrespecttothe
tiplesof2πacquiredbythephase. Nr can becomputedbyfol- rotationaxisexist.Sincetheprecedingtheorydoesnottakethis
lowing the evolution of the eigenvector u over one period, and phenomenon into account, the theoretical value of δ(cid:4) is multi-
we verifiednumericallythat, alternatively, Nr is also the wind- pliedbytwowhentherayγcoincideswiththerotationaxis,i.e.
ing numberaroundγ of a ray nearbyγ duringone period.The for rotation rates less than the bifurcationpoint. Finally, it can
lastterminEq.(42)istheMaslovphase(Gutzwiller1990)that benotedthataformulasimilartoEq.(43)canalsobeobtained
comes from the reflection of the wave on the boundaries. The throughtheformalismoftheGutzwillertraceformulafollowing
formulaforthefrequencies,ωn,l,m,ofislandmodesisthus themethodofMiller(1975).
(cid:19) (cid:3) (cid:4) (cid:3) (cid:4) (cid:20)
1 1 1
ωn,l,m = (cid:18) ds 2π n+ 2 + (cid:4)+ 2 (2πNr+α) (43) 4. Comparisonwithnumericalresults
γ c˜s
Asthepresentasymptotictheoryreliesonvariousassumptions,
or,inaformthatmakestheregularitiesmorevisible,
itsrelevanceforstellarseismologyisnotguaranteedandneeds
ωn,l,m =δn(m)n+δ(cid:4)(m)(cid:4)+β(m), (44) to be assessed througha comparisonwith exactcalculationsof
realistic stellar models. In this section, the comparison is done
withthefrequencyregularities with numerically computed modes in uniformly rotating poly-
tropicmodelsofstars.Thehypothesesoftheasymptotictheory
2π 2πN +α
δn = (cid:18) ds and δ(cid:4) = (cid:18) rds (45) aisr,eftohrehfioglhloweninogu:ghfirfsrte,qituiesnvcaielisd. Sinecthoenda,sythmepitsoltaincdremgiomdee,stharaet
γ c˜s γ c˜s constructedfromastableislandofacousticrayphasespace.At
andtheconstantterm nullrotationsuchastructuredoesnotexist,soweexpectthatthe
δ +δ theoryfailstodescribesphericalmodeamplitudesandfrequen-
β= n (cid:4)· (46) cies. A stable island immediately appears at non-zerorotation,
2
but its phase space volume must be high enough for an island
The quantum numbersn and (cid:4) correspondto node numbersof mode to exist. This volume increases with frequencyand rota-
the p-mode, respectively in the longitudinal and transverse di- tion (see Lignières & Georgeot 2008, 2009, for details). Thus,
rectionsofthecentralrayγasillustratedinFig.3.Thisistobe for a givenfrequencyrange,the numberof island modesstarts
contrastedwiththecaseofasphericalmodewherethemostnat- fromzeroatsmallrotationratesandprogressivelyincreasesas
urallabeling are the quantumnumbersof spherical harmonics. therotationandthusthephasespacevolumeofthestableisland
Equation (43) is a semi-analytical formula since the quantities grows. Actually, low degree spherical modes become progres-
Tγ, Nr and α must be computed numerically from the Runge- sively island modes as rotation increases. Another assumption
KuttaintegrationoftheHamiltonianequationsforacousticrays. usedinfinding√asolutiontothewaveequationisthatthemode
Theacoustictime,Tγ,isdirectlycomputedfromγitself.From decaysas∝1/ ωinthedirectiontransversetotheperiodicray.
theintersectionsofaraynearbyγwiththePSS(ascanbeseen Finally, the theory also neglectsthe Coriolis force and the per-
inFig.2),wecomputethemonodromymatrixMthatmapsone turbationsofthegravitationalpotential.
intersectionwiththePSStothenextone.Then,bydiagonalizing In the following, the asymptotic theory is compared with
thismatrixoneobtainstheFloquetphaseαfromitseigenvalues, highlyaccuratecomputationsofhighfrequencyadiabaticmodes
A11,page6of13
M.Paseketal.:Regularoscillationsub-spectrumofrapidlyrotatingstars
Table 1. Island mode quantum numbers n, (cid:4), m of numerically com-
puted modes, corresponding to n ∈ [21,25], (cid:4) ∈ {0,1,2,3}, m ∈
s s s
[−(cid:4),(cid:4)]intermsofsphericalmodequantumnumbers.
s s
n (cid:4) m
42,44,46,48,50 0 –3,–2,...,3
42,44,46,48,50 1 –1,0,1
43,45,47,49,51 0 –2,–1,...,2
43,45,47,49,51 1 0
inuniformlyrotatingpolytropicstellarmodelswithindexN =3,
theCorioliseffectandperturbationsofthegravitationalpotential
beingtakenintoaccount.Theaccuracyofthesecalculations,de-
scribedindetailinReeseetal.(2006),isveryhigh(therelative
precisiononthefrequenciesis10−7)andthusdoesnotinterfere
withthepresentcomparison.Alargenumberofmodeswerefol-
lowedfromΩ/Ω = 0toΩ/Ω = 0.896.Atzerorotationthese
K K
modesare low degree (cid:4) ∈ {0,1,2,3},high order n ∈ [21,25]
s s
modes.Athigherrotationrates,theybecomeislandmodesand
canthusbelabeledwithnand(cid:4),thenumberofnodesalongand
transversetoγ,respectively,asillustratedinFig.3.Therelation
betweenthequantumnumbersatzeroandhighrotationratesis
(Reese2008):
n=2n +[((cid:4) +m )mod2], (47)
s s s
(cid:4) −|m |−[((cid:4) +m )mod2]
(cid:4)= s s s s , (48)
2
m=m . (49)
s
We remind the reader that rotational multiplets are defined, in Fig.4. Comparison of frequency spacings δn,(cid:4) between island modes,
thenon-rotatingcase,asasetoffrequencieswithidenticaln ,(cid:4) computed from numerical simulations and semi-analytical formulas
quantumnumbersbut differentvalues of m for m ∈ [−(cid:4) ,s(cid:4) ]s. for differen(cid:22)t values of Ω/ΩK (the frequency spacings are normalized
s s s s
For rotatingstars, we can definemultipletsas frequencieswith by ω = GM/R3 with R the polar radius). Circles: δ , triangles:
p p p n
identical n and (cid:4) but differentm ∈ Z, i.e. without any limiting δ(cid:4), orange/gray: semi-analytical results, blue/black: numerical results.
valueform.Therelationbetweenthetwosetsofquantumnum- Numericalresultscorrespondtodifferentsetsofδnum andδnum values,
n (cid:4)
bersandthetwotypesofmultipletsisillustratedinFig.3.Inthis with n ∈ [42,51], (cid:4) ∈ {0,1} for m = 0; and n ∈ [42,51], (cid:4) = 0,
figurethemultipletsofislandmodescorrespondtodiagonalcol- n ∈ {42,44,46,48,50}, (cid:4) = 1 for m ∈ {−1,1}. Upper panel: m = 0.
Lowerpanel:m=±1.(Thisfigureisavailableincolorintheelectronic
oredbands, whereasthe multiplets at zerorotation wouldhave
form.)
the form of vertical bands. We restricted ourselves to numeri-
cal modes with (cid:4)max = 3 so, in terms of island mode quantum
s
numbers, the range of numerically computedmodes is the one
given in Table 1. The associated numericalfrequencyspacings ofhigh-frequencyp-modesareingoodagreementforalmostall
aredefinedas: rotationrates.Form = 0,aroundΩ/ΩK (cid:3) 0.26,the agreement
degradessignificantly.Inthisrotationrange,therayγinthecen-
δnnum =ωnnu+m1,(cid:4),m−ωnnu,(cid:4)m,m, (50) ter of the main stable island undergoesa bifurcation from one
stable ray on the polar axis to two stable rays surroundingone
and
unstable ray. When such a bifurcation occurs, the eigenvalues
δnum =ωnum −ωnum . (51) of the monodromymatrix become Λ± = 1, correspondingto a
(cid:4) n,(cid:4)+1,m n,(cid:4),m Floquetphase α = 0 mod2π(see e.g. Brack2001).Thisis in-
The semi-analytical asymptotic theory also requires determin- deedwhathappensatΩ/ΩK (cid:3)0.26,asδ(cid:4) ∝αgoestozero.Such
ingtheαterminEq.(45)numerically.Totesttherobustnessof abehaviorconveysthenon-validityofthepresentnormalform
thiscalculation,we checkedthatthe frequencyspacingδ(cid:4) only approximationforraysundergoingabifurcation.Onepossibility
weakly depends on the choice of the ray nearby γ that is used wouldbetouseotherlocalapproximationsoftheraydynamics
to compute α. Also, the spacings δn and δ(cid:4) neither depend on calleduniformapproximations(Schomerus&Sieber1997).The
the resolution of the background model nor on the integration discrepancycomingfromthe bifurcationis notto be foundfor
parametersoftheRunge-Kuttamethod. m (cid:2) 0, since the stable ray stays away fromthe polar axisand
doesnotundergoabifurcationasrotationincreases.
Although,as mentionedbefore,thetheoreticalandnumeri-
4.1.Regularfrequencyspacings
calfrequencyspacingsarenotexpectedtomatchforslowrota-
AccordingtoEq.(44),thestructureofthespectrumischaracter- tionrates,thediscrepanciesremainsmallinthisrotationrange.
izedbythetwospacingsδn(m)andδ(cid:4)(m).InFig.4,theirsemi- Thisisduetothefactthat,asΩ/ΩK approacheszero,thestable
analyticalandnumericalvaluescomputedform=0and|m|=1 rayisalongthepolaraxisand,accordingtotheexpressionofδ ,
n
arecomparedasafunctionoftherotationrate.Onecanseethat thisimpliesthatδ = Δ/2,i.e.halfthelargeseparationdefined
n
thesemi-analyticalregularitiesδn,δ(cid:4),andthefullcomputations inEq.(3).Now,usingthefirstorderofTassoul’sformulaandthe
A11,page7of13
A&A546,A11(2012)
Startingfrom
δ ((cid:4)=0)=[δ (m)−δ (0)]n+[β(m)−β(0)], (53)
m n n
we first assume that n is large enough to neglect β(m) − β(0).
FromEq.(45),δn(m)isequalto2π/Tγ(m)where
(cid:23)(cid:24)(cid:25) ⎡ (cid:9) (cid:10)⎤
(cid:21)
Tγ(m)= γ dcss 1− ω12 ⎢⎢⎢⎢⎢⎢⎣ω2c + c2s md22− 14 ⎥⎥⎥⎥⎥⎥⎦· (54)
The dependence of Tγ on m is explicit in the integrand but is
implicitintheintegrationpathγ.Inthefollowing,thevariation
ofthelocationofγwithm/ωisassumedtobenegligible.Then,
anexpansionin1/ωoftheintegrandinEq.(54)leadsto:
Fig.5. Comparison of frequency spacing δ between island modes, (cid:21) ⎡(cid:21) (cid:21) (cid:9) (cid:10) ⎤
cEoqm.(p5u3t)edforfrdoimfferneun(cid:22)mtverailcuaelssoifmΩu/laΩtiKon(stheanfdremqsueemnic-yansaplayctiicnaglsfaorermnuolra- Tγ(m)(cid:3) γ dcss − 2ω12 ⎢⎢⎢⎢⎢⎢⎣ γω2cdcss + γ cs m2d−2 1/4 ds⎥⎥⎥⎥⎥⎥⎦. (55)
malized by ω = GM/R3 with R the polar radius). Orange/gray:
p p p
semi-analyticalresultsofEq.(53)forn = 42andm = 1, blue/black: Hence we obtain an approximate expression for δn(m) of the
numericalresults.Thenumericalresultscorrespondtodifferentsetsof form:
δ forn ∈ [42,51],(cid:4) = 0andm ∈ {−1,1}.(Thisfigureisavailablein (cid:18) (cid:18)
comlorintheelectronicform.) δn(m)(cid:3) (cid:18)2πds + ωπ2 γ ωcs2cds+(cid:15)(cid:18) γ cs(cid:16)(2m2d−21/4)ds· (56)
γ cs γ dcss
quantumnumbersconversionrulesEqs.((47)–(49)),itiseasyto
bseeectlhoaste,taothzearlfotrhoetalatirogne,sδennpuamra=tioωnnn.u+mN1,(cid:4)o,mte−alωsonnu,(cid:4)tm,hmatistheexpdeocutbedlintog Infegwleecitnβs(emrt)t−heβ(p0r)e,vwioeuhsaevxepression for δn(m) in Eq. (53) and
⎡ ⎤
iToisnfadsdtihsucoeeautneltu’ossmttthhheeaeritocsrδamy(cid:4)l.avCglaloolesunesecpsetaorornba2itsnδiegonr,nvδte(cid:4)th,dhatahittneiasFspeiΔpgm,e.ia4f-orasarntaassltlmyottwhaicellarnloroectatxaatltitcoiouonrlndaretraiarottenoesssf. δm((cid:4)=0)(cid:3)⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎢⎣mω22π(cid:15)(cid:18)(cid:18)γ dcd2ssd(cid:16)s2⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎥⎦n. (57)
Again, Tassoul’s formula applied to the n, (cid:4) quantumnumbers γ cs
shows that δnum is close to Δ. For these reasons, the frequency (cid:22)
spacings δn a(cid:4)nd δ(cid:4) converge to the results of the first order of Finally,normalizingbyωp = GM/R3p andreplacingωbynωn
Tassoul’sformula,thoughtheirderivationisformallynotpossi- yields
blefornon-rotatingstars. (cid:18)
(cid:3) (cid:4) (cid:3) (cid:4)
entImnaosrdreortatotioinnviensctirgeaatseesth,ewdercifotnbseitdwereethnethferesqpueecntrcayosfpdaciffinegr-: δm((cid:4)ωp=0) (cid:3) √mn 2π(cid:15)(cid:18)γ dcd2ssd(cid:16)s2 ω/nωp 2 ω13p
δm =ωn,(cid:4),m−ωn,(cid:4),0. (52) (cid:3) m (cid:4)2 1γ cs(cid:21) c
(cid:3) √ sds, (58)
Figure5displaysacomparisonbetweenthenumericalandsemi- n 4πωp γ d2
analyticalvaluesofδ for(cid:4) = 0and|m| = 1.Asexpected,the
m
agreementisnotgoodatsmallrotationrates.Usingthefirstor- where we have used the fact that n stays constant in the
ω/ω
p
derofTassoul’sformulatoapproximatethenumericalresultsat freq(cid:18)uency range considered here, and is known to be close to
zwehreonroΩta/tΩion,=δnm0u.mT=hisωinnsu,(cid:4)nm,mo−tcωomnnu,(cid:4)mp,0aitsibfloeuwndithtothbeeacslyomsepttoot|mic|Δth/e2- ωp γ dcss/2π.Thepreviousexpression(cid:2)willbemademoreprecise
oryoftheisKlandmodesinceitpredictsthatδ goestozerowhen byrenormalizingthevalueofcsby 1−ω2c/ω2totakeintoac-
ωgoestoinfinity.Indeed,δ (m)dependsonmm/ωbecausec˜ and countthatωc isnotnegligible,andindeedisof theorderofω,
the ray path γ, given by thne Hamiltonian Eq. (7), both despend close to the stellar surface. In Fig. 6, the numerical values of
onm/ω.Analternativeexplanationistoconsiderthespatialdis- δm((cid:4)=0)aswellasres√ultsforthelastterminEq.(58)areplot-
tribution of island modes of fixed m and (cid:4): one finds that in- ted as a function of m/ n for Ω/ΩK = 0.419, showing a good
creasingωproducesbothlargerderivativesalongthestableray agreement.This behavioris valid for rotationrates higherthan
Ω/Ω (cid:3)0.4.Itmustalsobenotedthatinthenumericalcalcula-
associated with a higher node number n, and larger√transverse K
derivativesbecause the transverseextentscales as 1/ ω. Thus, tionsbyReeseetal.(2009),usingmorerealisticstellarmodels,
theasymptotic √m dependencywasalsofoundempirically.
thecontributionoftheazimuthalderivativesbecomesnegligible n
inthewaveequation(Eq.(4)).Wealsoverifiedthatδ displayed
m
inFig.5diminisheswhennisincreased.Thus,forrotationrates
4.2.Pressureamplitudesofislandmodes
such thatthenumericalmodesare notfully island modes,they
behavemorelikesphericalmodesandδnumshowscleardiscrep- In this section, we compare the results obtained from the
m
ancieswiththeasymptoticresults.Bycontrast,athighrotation semi-analyticalformula for mode spatial distributions Eq. (41)
rates,anapproximateanalyticalformulaforδ isderivedinthe with results from full numerical computations. Equatorial cuts
m
followingandshowntocloselyreproducethenumericalresults. of the semi-analytical modes can be expressed as a function
A11,page8of13
M.Paseketal.:Regularoscillationsub-spectrumofrapidlyrotatingstars
Fig.6.Frequencyspacingsδ√m((cid:4)=0)=ωn,(cid:4)=0,m−ωn,(cid:4)=0,m=0,normalized
by ω , as a function of m/ n. The integers n, (cid:4) and m are the quan-
p
tum numbers of island modes. The rotation rate is Ω/Ω = 0.419.
K
Blue/black dots: numerical results. Orange/gray dashed line: semi-
analytical results for the last term in Eq. (58). Numerical modes in-
cluded are for quantum numbers n ∈ {42,44,46,48,50}, (cid:4) = 0 and
m∈[−3,3].(Thisfigureisavailableincolorintheelectronicform.)
√
of ν= ω(r−r ), where r is the radial position of the ray γ,
0 0
while the value of Γ is obtained from the eigenvectors of the
monodromy matrix M. In Fig. 7, the equatorial cuts of semi-
analytical and numerical modes are plotted for different rota-
tional velocities and quantum numbers (cid:4) and m. The chosen
modes are representative of the different behaviors observed.
Discrepanciesbetweensemi-analyticalandnumericalresultsare
mainlyduetoedgeeffects.Thisoccu√rswhenthetransverseex-
Fig.7. Examples of normalized amplitudes distributions (real part
tent of the mode(which scales as 1/ ω) reacheseither the po- ofΦ(cid:4))ontheequatorasafunctionofpositionr/R (whereR isthe
lar axis for small rotations, or the surface near the equator for m eq eq
equatorialradiusofthestellarmodel)forsemi-analyticalandnumeri-
highrotations.Finally,avoidedcrossingscanalsocontravenean calmodes.ModesdisplayedareforΩ/Ω = 0.300(leftcolumn),and
K
accuratepredictionformodeamplitudessincetheamplitudesof Ω/Ω = 0.707 (rightcolumn). Quantum numbers(n,(cid:4),m)areasfol-
K
crossingmodeswillbelinearcombinationsofallthemodescon- lows.Leftcolumn(50,0,0),(50,1,0),(50,0,1),(50,1,1).Rightcolumn
tributing to the crossing.Hence, modesundergoingan avoided (50,0,0),(50,1,0),(50,2,0),(50,3,0).Blue/blackcontinuousline:nu-
crossingcandiffersignificantlyfromEq.(41)(cf.thirdpanelin mericalresults;orange/graydashedline:semi-analyticalresults.(This
Fig.7).Overall,thereisneverthelessagoodagreementbetween figureisavailableincolorintheelectronicform.)
thesemi-analyticalandnumericalresultsformodespatialdistri-
butions,showingthevalidityofEq.(41).
the spectrum’s organization.This is shown in Fig. 9 where for
clarity only a few m = 0 modes have been displayed: the (cid:4) =
5. Phenomenologyandobservables 0,n∈[43,46]and(cid:4)=1,n∈[42,44]modes(orequivalentlythe
(n ,(cid:4) ) ∈ {(21,1),(21,2),(21,3),(22,0),(22,1),(22,2),(23,0)}
forasteroseismology s s
modes). Starting from the usual structure at zero rotation in-
In this section, we show that the asymptotic theory provides a volvingthe largeandsmallseparationsofTassoul’stheory,the
simpleunderstandingoftheevolutionoftheislandmodespec- spectrumreorganizationinducedbythedecreaseofδ(cid:4) canalso
trumwithrotation.Then,thephysicalcontentofthepotentially beviewedasanincreaseofthesmallseparationδ.Then,above
observablefrequencyspacingsδn,δ(cid:4)andδmisdiscussed. Ω/ΩK (cid:3)0.45,thestructureofthem=0spectrumremainsprac-
Figure 8 displays the global evolution of all the numerical ticallyunchanged.
frequencies considered in the observer’s frame, whose island Now,toillustratetheevolutionofthespectraofdifferentm,
mode quantum numbers can be found in Table 1 (or equiva- Fig.10displaysthen = 44,(cid:4) = 0,m ∈ {−2,−1,1,2}modefre-
lently n ∈ [21,25], (cid:4) ∈ [0,3], m ∈ [−(cid:4) ,(cid:4) ] in spherical quenciesasafunctionoftherotationratetogetherwiththen ∈
s s s s s
modes quantum numbers). The first phenomenon that can be [43,46],(cid:4)=0,m=0frequencies.Themainfeatureofthisevo-
noticed is a global decrease of frequencies with rotation. This lutionisthe decreaseofδ from(cid:3)Δ|m|atzerorotationtovery
m 2
effect is simply due to the increasing volume of the star when smallvaluesathighrotations.Whenmultipletsofislandmodes
itisspinningrapidly.Besidesthisglobaleffect,theevolutionof are defined as in Sect. 4 and Fig. 3, they show no regularityat
the spectrum’s organizationcan be inferred from the evolution smallrotationrates.Note,however,thatthesplittingωm −ω−m
offrequencyspacingsδn,δ(cid:4)andδm.Thespacingδnstaysalmost isalwaysverycloseto−2mΩbecausetheeffectsoftheCoriolis
constantfromnulluptohighrotations,itsvalueremainingclose force are negligible. By contrast, at high rotation rates, as δ
m
to half the large frequency separation of the spherical model. vanishes above Ω/Ω (cid:3) 0.45, the m ∈ {−2,−1,0,1,2} modes
K
If a large number of island modes are detected in an observed clearlyformaregularmultiplet,ascanbeseeninFig.√10,where
spectrum,δ shouldbe easily extractedfrom the data. By con- deviations from strict Ω spacings are due to the (m/ n)2 term.
n
trast,therapidevolutionofδ(cid:4)withrotationwillstronglymodify Sinceforsuchrotationratesthestructureofthem=0spectrum
A11,page9of13
A&A546,A11(2012)
Fig.8.Normalizedfrequenciesω/ω asafunctionofnormalizedrota- Fig.10.Normalizedfrequenciesω/ω asafunctionofnormalizedrota-
p p
tionvelocityΩ/Ω intheobserver’sframe.Differentcolorscorrespond tionvelocityΩ/Ω .Thequantumnumbersofthedisplayedmodesare:
K K
todifferentvaluesof|m|.Blue:m=0,green:|m|=1,red:|m|=2,cyan: n = 44,(cid:4) = 0,m ∈ {−2,−1,1,2}withthen ∈ [43,46],(cid:4) = 0,m = 0
|m| = 3. Modes included are for the quantum numbers n ∈ [21,23], modes. Blue: m = 0, red: |m| ∈ {1,2}. For clarity, the corresponding
s
(cid:4) ∈[0,3],m ∈[−(cid:4),(cid:4)](seeTable1).(Thisfigureisavailableincolor degrees of the spherical harmonics are also written. Continuous line:
s s s s
intheelectronicform.) frequencies in the observer frame, dashed line: in the rotating frame.
WehaveoutlinedthelargefrequencyseparationΔandtheδ spacing
m
witharrows.(Thisfigureisavailableincolorintheelectronicform.)
the m values of the modes, if m is small. This property trans-
latesitselfintoaclusteringofthefullspectrumintheobserver’s
framebecauseitturnsoutthatthisrotationrateisclosetoδ /2;
n
andδ ,thatdecreasesfromaninitialvalueofmδ tozeroathigh
m n
rotation,isaroundmδ /2atthisintermediaterotation.Thesec-
n
ondfrequencyclusteringclosetoΩ/Ω (cid:3) 0.56isrelatedtothe
K
factthatδ vanishesathighrotation.Inthisregime,thedifferent
m
mspectraareexpectedtocollapseontoasinglespectruminthe
rotatingframebutnotintheobserver’sframe.However,whenΩ
isequaltoδ ,theneardegeneracyofthemspectraproducesthe
n
frequencyclusteringobservedatΩ/Ω (cid:3)0.56.
K
Fig.9. Normalized frequencies ω/ω as a function of normalized ro- Oneoftheinterestsofasymptotictheoriesinasteroseismol-
p
tation velocity Ω/Ω . The quantum numbers of the displayed modes ogy is to gain physical insights into seismic observables such
K
are: (cid:4) = 0, n ∈ [43,46] and (cid:4) = 1, n ∈ [42,44] with m = 0. Blue: asδ , δ,andδ . Inthe following,we brieflydiscussthispoint
n l m
(cid:4)=0,green:(cid:4)=1.Forclarity,thecorrespondingdegreesofthespher- withemphasisonthedifferencesandsimilaritieswiththephys-
icalharmonics arealsowritten.Wehaveoutlined thelargefrequency ical content of the large and small separation from Tassoul’s
tesheleepcaδtrrnao,tniδoi(cid:4)cnsfpΔoar,cmtihn.e)gssmwailtlhfarerrqouwesn.c(yTsheipsafiragtuioreniδsa=vaωilnas,b(cid:4)sle−inωcnso−l1o,(cid:4)rs+i2natnhde tThγeo=ry(cid:18).γTdch˜sseaslpoancgintgheδnacdoeupsetnicdsraoynγly.oWnetheexpaeccotuTstγictotrabveedlotimmie-
natedbythetimespentinthesub-surfaceregionwherethesound
speedismuchsmallerthanintheinterior.Whilethepathofthe
remainsunchanged,the evolutionof thewholespectrumin the rayvarieswithrotation,δn remainsapproximatelyproportional
observer’sframeisdominatedbytheadvectiontermmΩ. to the mean density as shown by Reese et al. (2008). On the
The globalevolutionof mode frequenciesin the observer’s otherhand,theδ(cid:4)spacingdependsalsoonthesecondderivatives
ofthesoundspeedtransversetotherayγ, aninformationinte-
frame shown in Fig. 8 also presents some particular events: a
firstclusteringofmodefrequenciesoccursaroundΩ/Ω (cid:3)0.25 gratedallalongtheray.Aslongasthepathofthestableraygoes
K
and then a second one around Ω/Ω (cid:3) 0.56. Both phenom- throughthecentralregionofthe star, the islandmodefrequen-
K
ciesshouldbesensitivetothechemicalstratificationandthusthe
ena can be understood from the asymptotic theory. According
ageofthestar.However,afterthebifurcationatΩ/Ω (cid:3) 0.26,
totheasymptoticformulasEqs.((43)–(46)),crossingsofmode K
frequencieswillhappenwhenδ(cid:4)/δn, orequivalentlyα/π,hasa the ray path progressively avoids the central region and the is-
land modes do not contain this information anymore. Another
rationalvalue.Thoughtheasymptotictheorypredictstrueeigen-
valuecrossings,itisknownthatthesecrossingswillbeavoided interestingpropertyofδ(cid:4) (orδ(cid:4)/δn)isthatitisverysensitiveto
rotationaslongasΩ/Ω ≤0.35.Finally,forhighrotationrates
if the two modes are of the same symmetry class (Landau & K
Lifshitz 1977). As can be seen in Fig. 4, δ(cid:4) becomes equal to (Ω/ΩK ≥0.40),thevalueofδm,thatcanbedetectedthroughthe
δ at some rotation rate around Ω/Ω (cid:3) 0.25 where the spec- irregularityof multiplets, a(cid:18)lso givesan informationon rotation
trnum for a given m simplifies to ωn,mK = δn(m)n + β. The de- since it is proportionalto γ dc2sds (Eq. (58))where the distance
generacyoccurs between modes of a differentsymmetry class, of the ray to the rotation axis d strongly depends on the rota-
andtherotationrateatwhichitoccursdependsonlyweaklyon tionrate.
A11,page10of13