Notes on Vector Calculus (following Apostol, Schey, and Feynman)

50 Pages·2007·0.31 MB·English

by  Frank Benford

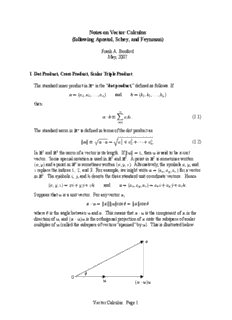
Notes on Vector Calculus
(following Apostol, Schey, and Feynman)
Frank A. Benford
May, 2007
1D. ot Product, Cross Product, Scalar Triple Product .
The standard inner product in (cid:145) is8 the “,” ddeoftin perdo adsu fcotllows.If
+ (cid:156)+(cid:223)+(cid:223)Æ(cid:223)+(cid:156),(cid:223),(cid:223)Æ(cid:223), and ,
"#8"# 8
aba b
then
8
+(cid:134)·,+, (cid:222) (1.1)
" 3 3
3(cid:156)"
The standard norm in (cid:145) is8 defined in terms of the dot product as
++·+(cid:134)(cid:156)+(cid:128)+(cid:128)(cid:226)(cid:128)+#(cid:222) # # (1.2)
l l ¨ (cid:201) " # 8
In (cid:145) an#d the(cid:145) n$orm of a vector is its length. If , then ?is sa(cid:156)id" to be a? unit
l l
vector. Some special notation is used in (cid:145) a#(cid:145)n$d . A point in(cid:145) #is sometimes written
B(cid:223)CB(cid:223) aCn(cid:223)DdB a Cpoint in (cid:145) is$ sometimes written . Alternatively, the symbols , , and
aba b
D" r(cid:223)e#p(cid:223)(cid:156)lac+e(cid:223)+ t(cid:223)h+e indices and 3. For example, we might write for a+ vector
BC D
a b
in (cid:145). $The symbols , , 3a4nd den5ote the three standard unit coordinate vectors. Hence
B(cid:223)C(cid:223)D(cid:156)B(cid:128)C(cid:128)34D5(cid:156)++34(cid:223)+(cid:223)+(cid:156)+5(cid:128)+(cid:128)an+d .
BCDBC D
aba b
Suppose that ?is a unit vector. For any vector ,B
B?(cid:134)(cid:156)B? cBos(cid:156)c)os )
lllll l
where ) is the angle between a?nBd.B ?This means that is theB c(cid:134)omponent of in the
direction of ?, aBn?d? (cid:134) is the orthogonal projeBction of onto the subspace of scalar
a b
multiples of ?(c alled the subspace of vectors “spanned” by ) ?Th(cid:222)is is illustrated below.
B

)
!
?
B?(cid:134) ?
a b
Vector Calculus. Page 1
The c orfo tsws op rvoedcutocrts and in is defi+ne(cid:156)d +a(cid:223)s+(cid:223)+(cid:156),(cid:223),(cid:223), , (cid:145)$
BCDBC D
aba b
+,(cid:130)34·+,(cid:129)+,(cid:128)+,(cid:129)+,(cid:128)5+,(cid:129)+ ,
CDDCDBBDBCC B
ababa b
(cid:226) 34 5 (cid:226) (1.3)
(cid:226) (cid:226)
(cid:156) (cid:226)+B+C +D (cid:226).
(cid:226) (cid:226)
(cid:226),, , (cid:226)
BC D
(cid:226) (cid:226)
It may be shown that e+q,u(cid:130)+als the, area of the parallelogram determined by and
l l
(i.e., w+h,e+r,e + sisin th),)e )angl1e between and , ), and that is !(cid:159)(cid:159) (cid:130)
lll l
orthogonal to the plane determined by + a,n+d . M,ore precisely, the direction of is (cid:130)
determined by the “right-hand” rule as follows: if the right hand is held with the thumb stuck
out and with the fingers curled in the direction of rotation of + into , ,then the thumb points
in the direction of +. (cid:130)In ,other words, if the index finger of the right hand is pointed
forward and shows the direction of +, and if the middle finger is bent to show the direction
of ,, and if the thumb is perpendicular to the plane determined by the index and middle
finger, then the thumb points in the direction of +. , (cid:130)B+eca,use is ortho(cid:130)gonal to both
+ and ,, it follows that
++(cid:134),,+(cid:130)(cid:156),(cid:134) (cid:130)(cid:156)!(cid:222)
aba b
Also, it's clear that +fo+(cid:130)r! any(cid:156) vector . Cro+ss products have the following
algebraic properties.
+,(cid:130),(cid:156)(cid:129)+ (cid:130)
a b
+,(cid:130)-(cid:128)+,(cid:156)+(cid:130)(cid:128)- (cid:130)
ababa b
00+,(cid:130)+(cid:156),+(cid:130)(cid:156) ,(cid:130) 0
ababa b
(where 0 is any scalar). It may be shown that
+,(cid:130)-(cid:130)+-(cid:156),—+(cid:134),(cid:209)(cid:129)-—(cid:134) (cid:209) (1.4)
a b
for any three vectors +, ,, and .-
The s ocfa laanry ttrhirpelee vpercotdorusc ,t , and is defined as th+e, scalar-
+,(cid:130)(cid:134) -(cid:222)
a b
It may be shown that is+ t,(cid:130)h-e+ vo,(cid:134)lume of the parallelepiped determined by , ,
ka b k
and -. This suggests (and itmay be shown to be true) thata cyclic permutation of the three
vectors does not affect the scalar triple product; that is,
+,(cid:130)-(cid:134)(cid:156)(cid:130),(cid:134)(cid:130)-+(cid:156)-(cid:134)+ , .(1.5)
ababa b
The commutativity of the dot product then implies that the dot and cross products in a
scalar triple product may be interchanged:
+,(cid:130)-(cid:134)(cid:156)(cid:134)(cid:130)+, (cid:222) - (1.6)
aba b
Vector Calculus. Page 2
Finally, it may be shown that the scalar triple product + m,(cid:134)ay -(cid:130)be written in terms of
a b
a determinant as follows:
(cid:226)+B+C +D (cid:226)
(cid:226) (cid:226)
+,(cid:134)(cid:130)(cid:156)- (cid:226),, ,(cid:222) (cid:226) (1.7)
BC D
a b (cid:226) (cid:226)
(cid:226)-- - (cid:226)
BC D
(cid:226) (cid:226)
2.The Gradient.
Let HbeH a subset of . :(cid:145) a8 onD H eifsi na imtioanppingsc farloamr f iienltdo ; a (cid:145)
vector field on His a mapping from inHto .(cid:145)8
Suppose that Hbe a subset of an(cid:145)d8 that is a d:ifferentiable scalar field defined on . H
For any point < in(cid:156) , Bth(cid:223)Be(cid:223) Æ-tu(cid:223)pBleH 8
"# 8
a b
‘‘:: ‘:
f·:·<(cid:223)(cid:223)(cid:226)(cid:223)grad (:2.<1)
aba b (cid:140)‘B‘"#B‘B 8 (cid:141)
(where each partial derivative is evaluated at <) is called the ogfr a. d Wiene'tll wr:ite f:
or grad :if the point where the partial derivatives are to be evaluated is clear. The
collection of vectors f co—:n(cid:209)s<titutes a vector field over . H
Example 1. Let .: —T<(cid:209)h·e·n<(cid:156)<B(cid:128)B(cid:128)(cid:226)(cid:128)B# # #
l l ¨ " # 8
‘‘:<"(cid:156)(cid:156)#BB(cid:128)B(cid:128)(cid:226)(cid:128)B ## (cid:156) # B (cid:129)"# 3.(2.2)
‘B‘B# a 3b(cid:136) "# 8 < (cid:137)
3 3
It follows that
f: <<(cid:156) < (cid:129)" , (2.3)
abl l
a unit vector in the direction of <.
As : is differentiable, the derivative of a:t wi<th respect to any vector exis?ts and is
denoted .: wI—t<(cid:224) m?a(cid:209)y be shown that
:w—<(cid:224)(cid:209)?(cid:156)<f—(cid:209)?(cid:134) : (cid:222) (2.4)
If ? is< a? unit vector, is: swa—(cid:224)id t(cid:209)o be a directional derivative; it's the rate of change of
: with respect to distance in the direction of ?. In this case,
::w—<(cid:224)(cid:209)?(cid:156)f— (cid:209)) < cos (2.5)
l l
where ) i:s :the angle between afnd—(cid:209) .f < T?—(cid:209)h<(cid:134)a?t i:s, is the component fof <
a b
in the direction of ? A(cid:222)(cid:156)s i!s mcoasx)im)al when f, it follows tha:t points in the
direction at which : in:creases fastest, and gifve—s t(cid:209)<he rat:e of change of in that
l l
direction.
Vector Calculus. Page 3
Suppose, now, that <is a differentiable vector-valued function that maps an interval of real
numbers +in(cid:223),toH .' F>o›r+ a(cid:223)n,y (cid:145) w8e write
cdc d
<—>(cid:209)(cid:156)B—>(cid:209)(cid:223)B—>(cid:209)(cid:223)Æ(cid:223)B—>(cid:209) (cid:222)
"# 8
a b
The parameter > i—s> (cid:209)commonly interpreted as .t i Tmhee vector trace<s out a curveor
“path” in (cid:145) a8s va>r+ie(cid:223)s, over . The vector of derivatives
c d
<w—>(cid:209)(cid:156)B—>(cid:209)ww(cid:223)B—>(cid:209)(cid:223)Æ(cid:223)B—w>(cid:209)
a "# 8 b
is called the v. e Tlohceit ny ovremct oorf athned is tangent to the curve at each point
velocity vector m<we—a>s(cid:209)ures the at whicshp etehdeu cnuirt vtea nisg etrnatversed. The
l l
vector Xis— d>(cid:209)efined as
<w—>(cid:209)
X· X —>(cid:209)· (cid:222) (2.6)
<w—>(cid:209)
l l
The afurnc-clteionng t his given by=
>
=—>(cid:209)(cid:156)—(cid:209) . <w 7 7 (2.7)
( l l
+
with derivative given by
=w—>(cid:209)(cid:156)—>(cid:209)<w .(2.8)
l l
Combining eqs. (2.6) and (2.8), we find that tmhea yu nbiet tianntegrepnrte vteedc taosr
the rate of change of < with respect to :=
<<w—>(cid:209).˛.>..<B.B
" 8
X ·(cid:156)(cid:156)(cid:156)(cid:223)Æ(cid:223) (cid:222) (2.9)
=w—>(cid:209).=˛.>.=.=.= (cid:140) (cid:141)
In (cid:145), w$e write , so<3—>4(cid:209)(cid:156)B—>(cid:209)(cid:128)5C—>(cid:209)(cid:128)D—>(cid:209)
.B.C.D
X34(cid:156)(cid:128)(cid:128) 5 (cid:222)
.=.=.=
Now suppose that :is a differentiable scalar field defined on . H L1e·t (cid:137) : <(cid:222) Then
1(cid:192)+(cid:223),˜>›+(cid:145)(cid:223), and for each
cdc d
1—>(cid:209)·—>:(cid:209) (cid:222)<
c d
Under these assumptions, the function 1 i1s— d>(cid:209)ifferentiable, and the derivative isw given by
the following chain rule:
8
‘:
1—w>(cid:209)(cid:156) f : <—>(cid:209) (cid:134)<w—>(cid:209)(cid:156)B—>(cid:209) w (2.10)
c d " ‘B 3
3(cid:156)" 3
where each partial derivative is evaluated at <. — >T(cid:209)he dot product
Vector Calculus. Page 4
f: <—>(cid:209)(cid:134)—>X(cid:209)
c d
is called the d i.r eScotimonea alu dtheroirvsa wtivriet eo ffa:olor nthgi sthe curve .˛:.=
directional derivative, as
8
‘.:B .:
3
f(cid:134):(cid:156)(cid:156)X (cid:222) (cid:134)
" ‘B.= .=
3(cid:156)" 3
Potential functions. The meaning of “potential function” varies from author to author.
Broadly speaking, there are two definitions, one used by mathematicians and the other used
by physicists.
Mathematicians. Let Jbe a vector field defined on a set H ' (cid:145)8. If there exists a
scalar field : d:efined o:n sHuc(cid:156)h tfhat , theJn is said to be a potential function
for J.
Physicists. .L eItf Jtbhee rae veexcitsotsr afi eslcda ldaer ffiineeldd on a set H ' (cid:145)8
YH d(cid:156)ef(cid:129)infedY on suchY that , tJhen is said to be a for .In potential function J
mechanics, the notion of a potential function is applied almost exclusively tof orce fields .
A vector field J mJ aisy < sbaeid i ntote brper ae tf eiodfr acse tfhieel d acting force
a b
on a particle at the point <. J If there Jexists a potential function for a force field , then
is said to be c (ofnosr errevaastoinves that will be explained later). In other parts of
physics, the use of “potential function” is broadened. For example, in electrostatics the
force on a charge ;i;s given by wIhere is a Ivector field called the “electric(cid:222) field” If
there exists a scalar field Y s(cid:156)uc(cid:129)h that I fY, then Y is said to be an electrostatic
potential.
The two notions of “potential function” differ principally in a sign convention; clearly
Y—(cid:209)<(cid:156)(cid:129)—(cid:209) : < . I will attempt to use the symbols : Yand consistently to denote,
respectively, the mathematician's and physicist's meaning of “potential function.”
Example 2. In ,(cid:145) l$et , wYhe(cid:156)r<e< P is< a·nP integer and . Generalizing <
abl l
Example 1, it may be shown that
(cid:129)fY(cid:156)(cid:129)P< P(cid:129)#<
Hence Y is(cid:156) a(cid:129) pPot<ential function of the force field J P(cid:129)#<. The equipotential surfaces
of Y are concentric spheres centered at the origin.
Example 3: The Newtonian potential. Newton's law of gravitation says that the force
which a particle of mass Q ex7erKts7 oQn a˛ <particle of mass is a vector of norm #
and directed from the particle of mass 7 toQwards the Kparticle of mass , where is a
proportionality constant and < is the distance between the two particles. Hence, if the
particle of mass Q is placed at the origin and the particle of mass is7 located at
<3(cid:156)4B(cid:128)C5(cid:128)D , then the force acting on the particle of m7ass is given by
Vector Calculus. Page 5
K7Q
J<(cid:156)(cid:129)<· (cid:222) whe<re
<$ l l
Using Example 2, we see that J wh(cid:156)e(cid:129)ref . Y ItY f(cid:156)ol(cid:129)loKws7 tQha<t< (cid:129)"
a b
K7Q
Y—(cid:209)<· (cid:129)
<
is a potential function for Newtonian gravity.
Example 4: Central forces. A central or “radial” force field J in is(cid:145) o$ne that can be
written in the form
J<—(cid:209)(cid:156)2—<(cid:209) /
<
where < a·nd· is<< /a <unit vector (cid:129)in" th e direction of . Tha<t is, a central force
<
l l
is directed radially, either towards the origin (if 2),— <o(cid:209)r(cid:157) aw!ay from the origin (if
2—<(cid:209)(cid:158) ! ), and the magnitude of the force at any point depends only on the distance from
the center to that point. .P rEovpeorysi tcieonntPrarlo foofrce field is conservative. .
Define
L—<(cid:209)(cid:156)2—<(cid:209).<Y—(cid:209)(cid:156)(cid:129)L—a(cid:209)n(cid:222)d < <
( l l
Then
‘Y‘< B
(cid:156)(cid:129)L—w<(cid:209)(cid:156)(cid:129)2—<(cid:209)
‘B‘B <
from eq. (2.2). Similar results holds for ‘ aYn˛d‘ .C ‘TYhe˛r‘eDfore,
2—<(cid:209)
(cid:129)fY—(cid:209)<(cid:156)<(cid:156)/J2—<<(cid:209)(cid:156)— (cid:209)
<
<
as required.
3. Divergence and Curl
The symbol f is called “del” or “nabla.” It is useful to think of afs a vector opera:tor
‘‘ ‘
f(cid:156)(cid:223)(cid:223)(cid:226)(cid:223) (cid:222)
(cid:140)‘B‘"#B‘B 8 (cid:141)
In (cid:145), w$e write
‘‘ ‘
f(cid:156)(cid:128)3(cid:128)4 (cid:222)5
‘B‘C‘D
“Multiplication” by ‘/ ‘mBeans “take the partial derivative with respect to .” TBhat is, if
3 3
Vector Calculus. Page 6
:—<(cid:209)(cid:156)—B(cid:223):B(cid:223)Æ(cid:223)B (cid:209) is a scalar field,
"# 8
‘ ‘:
: · (cid:222)
(cid:140)‘B‘3(cid:141)B 3
Playing with this operator as if it were a real vector often (but not always) yields results that
turn out to be true. For the true results, then, this device has heuristic utility.
For example, suppose that J is a vector field defined on . HFo'r an(cid:145)y$point
<3·4B(cid:128)C5(cid:128)D H in we'll write
J<—<(cid:209)(cid:156)3<J4—<(cid:209)(cid:128)5J—(cid:209)(cid:128)J— (cid:209) .
BC D
Operating in a purely formal manner, we may form both a dot product and a cross product
of f and TJhe(cid:222)se operations yielda scalar
‘J‘J ‘J
B C D
f(cid:134)·J(cid:128) (cid:128) (3.1)
‘B‘C‘D
and a vector
(cid:226) 34 5 (cid:226)
(cid:226) (cid:226)
‘‘ ‘
f(cid:130) J· (cid:226) (cid:226)
(cid:226) ‘B‘C‘D (cid:226)
(cid:226) (cid:226)
(cid:226) JJ J (cid:226) (3.2)
BC D
(cid:226) (cid:226)
‘J‘J‘J‘‘JJ‘J
DBD C B C
(cid:156)(cid:129)(cid:128)(cid:129)(cid:128) (cid:129)34 5 ,
(cid:140)(cid:141)‘(cid:140)C‘(cid:141)D(cid:140)‘D‘B‘B‘C (cid:141)
where all partial derivatives are to be evaluated at the point <. Amazingly, both these
objects are meaningful and useful. The scalarf is(cid:134) cJalled the of anddiv iesrgence J
also written “div J TJ.”he vector is cfalle(cid:130)d theJ of and is aclusor lwritten
“curl J.”
We will give geometric interpretations of f(cid:134)fJ(cid:130) and afterJ our discussion of line and
surface integrals. However, two simple examples at this stage will start to give the reader
some idea of the meaning of the divergence and the curl.
Example 1. Suppose .J <T—<(cid:209)h·3a4t(cid:156) isB, (cid:128)th5Cis(cid:128) vDector field is radially
directed, and , Jth<e— <(cid:209)dis(cid:156)tance from the origin to . Henc<e,
lll l
‘B‘C‘D
divJ—<(cid:209)(cid:156)(cid:128)(cid:128)(cid:156)$(cid:222)
‘B‘C‘D
Example 2. Consider a rigid circular disk rotating around an axis through its center and
perpendicular to the plane of the disk. Without loss of generality, we may set up the
coordinate system so that the disk rotates in the B-pClane, and the axis of rotation coincides
with the D coordinate. Let d=enote the angular of stpheee ddisk (in radians per second).
Physicists find it convenient to let =de(cid:156)no=te5 t he angular of the divsekl:o cthitayt is,
Vector Calculus. Page 7
angular velocity is a vector with magnitude = directed along the aDx(cid:156)isB. (cid:128) LCet <3 4
denote a point on the disk. The speed of that point depends on = and on a<cc·ordi<ng
l l
to the equation .@ (cid:156)In m=<ore detail, the of that vpeolioncti t(ya @vector) is given by
(cid:226)34 5 (cid:226)
(cid:226) (cid:226)
@<(cid:156)3(cid:130)=(cid:156)(cid:156)4(cid:129)C(cid:128)(cid:226)B! ! =(cid:226) =
(cid:226) (cid:226) a b
(cid:226)BC ! (cid:226)
(cid:226) (cid:226)
where @N·ote t@h@a(cid:222)t is a vector field. We now ask: what is the curl o@f ? From
l l
eq. (3.2),
(cid:226) 34 5 (cid:226)
(cid:226) (cid:226)
‘‘ ‘
curl@@(cid:156)f(cid:130) (cid:156) (cid:226) 5(cid:226) (cid:156)#(cid:156)=# (cid:222) =
(cid:226) ‘B‘C‘D (cid:226)
(cid:226) (cid:226)
(cid:226)(cid:129)C=B =! (cid:226)
(cid:226) (cid:226)
In words, the curl of linear velocity is just twice the angular velocity of the disk.
So far in our play with f we've only considered first derivatives. When we consider second
derivatives, four of the possible combinations turn out to be meaningful and useful.
(1) dfiv(cid:134)fgrad: .(cid:156) Working form:ally, we find
aba b
‘‘‘‘‘ ‘ :: :
f(cid:134)f(cid:156)(cid:128):(cid:128)(cid:134)(cid:128) 3453(cid:128)4 5
a b (cid:140)(cid:141)‘(cid:140)B‘C‘D‘B‘C‘D (cid:141)
‘#: ‘#: ‘#:
(cid:156)(cid:128)(cid:128) (cid:222)
‘B‘##C‘D #
It turns out that this scalar field is very useful in physics. The operationf i(cid:134)s f:
a b
called the L oafp laancdi ains wri:tt:en . If fo:r all finf# so(cid:156)m!e vo#lum<e <
a b
H, the scalar function : is said to be . hTahrem Lonapiclacian of a vector field is J
defined “component-wise”: if J t4h(cid:156)enJ(cid:128)3J5(cid:128)J
BC D
f(cid:156)##J#f4J(cid:128)fJ(cid:128)#35fJ (cid:222)
BC D
—#(cid:209)f(cid:130)f(cid:156)(cid:130)::0 c(cid:156)urlgrad . For any v0ector and@ s@calar@, we know that
ababa b
@@(cid:130)!(cid:156)f0(cid:130) f. This suggests that , the curl: of a gradient, should equal .!
aba b
This turns out to be true under some weak conditions: if : is a scalar field with
continuous second-order mixed partial derivatives, then curl(grad :)Co(cid:156)nv!e(cid:222)rsely, it
may be shown that if curl J f!o(cid:156)r all points in Ban open convex set , then thHere
exists a scalar field : defined on sHuc(cid:156)hf tha(cid:222)t J :
(3) (fdi(cid:134)vfc(cid:130)ur(cid:209)l(cid:156) F(cid:222)J(cid:134)orJ a+n(cid:130),y+ v+ec(cid:156)to,rs and , we know that 0.
aba b
This suggests that f(d(cid:134)ifvc(cid:130)ur(cid:209)l(cid:156) . (cid:156) JTh!is is in fact tJhe case: if all the
a b
mixed partial derivatives of a vector fieldJ are continuous, then ( f(cid:134)f(cid:130)(cid:209) (cid:156)J
divcurl . J Co(cid:156)nv!eHrsfel(cid:134)y(cid:156), if! is an open interval in , and thro(cid:145)ug$hout K
a b
Vector Calculus. Page 8
H, then KcuJr(cid:156)l for some vector field J.[An “open interval” in is the Cart(cid:145)es$ian
product of open intervals. That is, an open interval in (cid:145) h$as the form
+(cid:223),(cid:130)—+(cid:223),(cid:209)(cid:130)—+(cid:223),(cid:209)+(cid:157),(cid:223)+(cid:157),(cid:223)+ (cid:157)wh,ere and .]
BBCCDDBBCCD D
a b
(4) cfu(cid:130)rlcfu(cid:130)rl(cid:156)(cid:130)J(cid:130)J+,(cid:156) - . Equation (1.4) may be writt en
ababa b
,+—(cid:134)-(cid:209)(cid:129)+,—-(cid:134)(cid:209)+,J -. If we sufbstitute for and , and for , we obtain
f(cid:130)f(cid:130)(cid:156)fJfJ(cid:134)(cid:129)JfJ(cid:134)f(cid:156)ffJ(cid:134)(cid:129)f # (3.3)
abababa b
which holds if all mixed partial derivatives are continuous. In other words,
curlcurl gJraJdd(cid:156)iv(cid:129) f J . #
aba b
(There are other ways the right-hand side of eq. (1.4) may be written, but these lead to
meaningless formulae whenf is substituted for a+nd .) ,
4. Line Integrals.
Let <be a vector-valued function that maps an interval of real numbers i+nt(cid:223)o,H ' . (cid:145)8
c d
If < is continuous on , t+h(cid:223)e,n is said< to be a in -spacceo.n tTinhueo puas tpha itsh 8
c d
said to be s imf o eoxtihsptsi ea<cnwedw iiss econtinuous in The path +is(cid:223), sa(cid:222)id to be
a b
smooth if +ca(cid:223)n, be partitioned into a finite number of subintervals in each of which the
c d
path is smooth.
Let <be a piecewise smooth path in -s8p+a(cid:223)c,e defined on an interval , and let be a J
c d
vector field defined and bounded on the graph of <. J The line< integral of along is
denoted by the symbol aJnd (cid:134)i.s <defined by the equation
’
,
J<(cid:134)J.·<—>(cid:209)(cid:134)—><(cid:209).>(cid:223) w (4.1)
( ( c d
+
whenever the integral on the right exists, either as a proper or improper integral.
Other notations for line integrals. If G d(cid:134)en.otes the graph of , th<eJ line <integral
’
is also written as anJd <is(cid:134)J .c+alled< If andthe integral of along G(cid:222)(cid:156)—+(cid:209)
’G
, ,
,<(cid:156)J—<,(cid:209) , thJen the line integral is sometimes written as or a(cid:134)n.d is called the
’+ ’+
,
line integral off r J o +m ., t <oWalhoenng the notation is useJd it should be kept in
’+
mind that the integral depends not only on the end points + and b,ut also (in general) on
the path <jo+inin,g them. When the(cid:156) path is said to be . The scylmosbeodl is often
)
used to indicate integration along a closed path. When J and ar<e expressed in terms of
their components, say
J<—<(cid:209)(cid:156)<<J—(cid:209)(cid:223)J—(cid:209)(cid:223)Æ(cid:223)J—(cid:209)—><(cid:209)(cid:156)B—>(cid:209)(cid:223)B—>(cid:209)(cid:223)Æ(cid:223)Ban—>d(cid:209)
"#8"# 8
aba b
then the integral on the right in eq. (4.1) becomes the integral of a sum (and a sum of
integrals):
Vector Calculus. Page 9
, 8 8 ,
J<(cid:134)<. (cid:156)J—>(cid:209)B—>(cid:209).>(cid:156)J—>(cid:209)Bw—><(cid:209).>(cid:222) w
( ( " 3cdc d3 "( 3 3
+ 3(cid:156)"3(cid:156)" +
In this case, the line integral is also written as . J In. B t(cid:128)heJ.B(cid:128)(cid:226)(cid:128)J.B (cid:145)#
’ ""##8 8
path <is usually written as a pair of parametric equations , aBn—d> (cid:209)t(cid:223)hCe— >l(cid:209)ine integral
a b
J<(cid:134). is written . SJim.iBla(cid:128)rlJy,. iCn the path is usuall(cid:145)y $written< as a
’G ’G B C
triple of parametric equations aBn—d>(cid:209) t(cid:223)hCe—> l(cid:209)i(cid:223)nDe— >i(cid:209)ntegral is written J (cid:134).<
a b ’G
J.B(cid:128)J.C(cid:128)J.D .
’G BC D
Basic properties of line integrals. Line integrals share many of the fundamental properties
of ordinary integrals. For example, they have al iwneiathr irteys ppreocpt etort ythe
integrand:
!"J!K(cid:128)<(cid:134)J.(cid:156)<K(cid:134).(cid:128)(cid:134).<"
((a b(
and an additive property with respect to the path of integration:
J<(cid:134)J.(cid:156)<J(cid:134).(cid:128)(cid:134)<.
(( (
GG "G #
where the two curves GanGd make up the curve G.
" #
Change of parameter. As evaluation of the integral maJke(cid:134)s. u<se of the parametric
’G
representation <, —it> (cid:209)might seem that an alternative parameterization of the curve woGuld
yield a different value of . IJn <f(cid:134)aJ.c(cid:134)t,. th<e value of is invariant with respect
’G ’G
to the parameterization of G up to a change of sign. Let be< a continuous path in -spa8ce
defined on an interval ,+ a(cid:223),nd let be a1 differentiable real-valued function defined on an
c d
interval -s(cid:223)u.c1h- t(cid:223)h.a1t- (cid:223)(.1+) (cid:223) i,s nevwer zero on , and (2) maps onto . Then
cdcdcdc d
the function º<d(cid:192)e-f(cid:223)i.ned˜ by (cid:145)8
c d
º<—?(cid:209)·1<?
c a bd
is a continuous path having the same graph G as .T<w<o path<s and so reºlated are said to
be e. q Iufi veavleernytswahmeer1e(cid:158)w o!n- (cid:223),. we say that and trace out in theG < º<
c d
direction,o panpdo siift e1 e(cid:157)wv!er-y(cid:223).where on , we say that and traceG o<ut in º<
c d
directions.o r Iine ntthaet ifoirns-t case, the change of parameter function 1 is said to be
preserving,o arinedn tiant itohne -sreecvoenrsdi ncgase it is said to be .
Theorem 4.1. Let <and bº<e equivalent piecewise smooth paths. Then we have
J<(cid:134)J.(cid:156)(cid:134).< º
( (
G G
if < and tº<race out in Gthe same direction, and
Vector Calculus. Page 10