Experimental study of heat transfer enhancement in a liquid piston compressor/expander using porous media inserts

11 Pages·2015·2.658 MB·English

by  Bo Yan, Jacob Wieberdink, Farzad Shirazi, Perry Y. Li, Terrence W. Simon, James D. Van de Ven

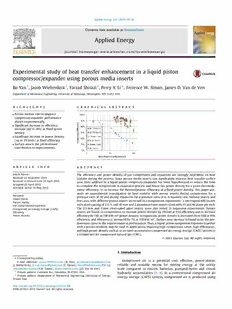
AppliedEnergy154(2015)40–50
ContentslistsavailableatScienceDirect
Applied Energy
journal homepage: www.elsevier.com/locate/apenergy
Experimental study of heat transfer enhancement in a liquid piston
compressor/expander using porous media inserts
Bo Yan1, Jacob Wieberdink1, Farzad Shirazi2, Perry Y. Li⇑, Terrence W. Simon, James D. Van de Ven
DepartmentofMechanicalEngineering,UniversityofMinnesota,Minneapolis,MN55455,USA
h i g h l i g h t s g r a p h i c a l a b s t r a c t
(cid:2)Porousmediasroletoimprove
compressor/expanderperformance
shownexperimentally.
(cid:2)Significantincreaseinefficiency
increase(upto18%)atfixedpower
density.
(cid:2)Significantincreaseinpowerdensity
(upto39folds)atfixedefficiency.
(cid:2)Surfaceareaisthepredominant
contributiontoimprovements.
a r t i c l e i n f o a b s t r a c t
Articlehistory: The efficiency and power density of gas compression and expansion are strongly dependent on heat
Received25November2014 transferduringtheprocess.Sinceporousmediainsertscansignificantlyincreaseheattransfersurface
Receivedinrevisedform24April2015 area,theiradditiontoaliquidpistoncompressor/expanderhasbeenhypothesizedtoreducethetime
Accepted25April2015
tocompletethecompressionorexpansionprocessandhencethepowerdensityforagiventhermody-
Availableonline14May2015
namicefficiency;ortoincreasethethermodynamicefficiencyatafixedpowerdensity.Thispaperpre-
sents an experimental investigation on heat transfer with porous inserts during compression for a
Keywords:
pressureratioof10andduringexpansionforapressureratioof6.Abaselinecasewithoutinsertsand
Liquidpiston
fivecaseswithdifferentporousinsertsaretestedinacompressionexperiment:3interruptedABSinserts
Porousmedia
withplatespacingof2.5,5,and10mmand2aluminumfoaminsertssizedwith10and40poresperinch.
Gascompression/expansion
Compressedairenergystorage(CAES) The 2.5mm and 5mm interrupted plate inserts were also tested in expansion experiments. Porous
Efficiency insertsarefound,incompression,toincreasepower-densityby39-foldat95%efficiencyandtoincrease
Powerdensity efficiencyby18%at100kW=m3powerdensity;inexpansion,powerdensityisincreasedthreefoldat89%
efficiency,andefficiencyisincreasedby7%at150kW=m3.Surfaceareaincreaseisfoundtobethepre-
dominantcauseintheimprovementinperformance.Thus,aliquidpistoncompressor/expandertogether
withaporousmediummaybeusedinapplicationsrequiringhighcompressionratios,highefficiencies,
andhighpowerdensitysuchasinanopen-accumulatorcompressedairenergystorage(CAES)systemor
acompressorforcompressednaturalgas(CNG).
(cid:2)2015ElsevierLtd.Allrightsreserved.
1.Introduction
⇑
Correspondingauthor.
Compressed air is a potential cost effective, power-dense,
E-mail addresses: [email protected] (B. Yan), [email protected]
(J.Wieberdink),[email protected](F.Shirazi),[email protected](P.Y.Li), reliable and scalable means for storing energy at the utility
[email protected](T.W.Simon),[email protected](J.D.VandeVen). scale compared to electric batteries, pumped-hydro and closed
1 Presentaddress:CumminsInc.,Columbus,IN47201,USA. hydraulic accumulators [1–4]. In a conventional compressed air
2 Presentaddress:DepartmentofMechanicalEngineering,UniversityofTehran, energy storage (CAES) system, compressed air is produced using
Tehran,Iran.
http://dx.doi.org/10.1016/j.apenergy.2015.04.106
0306-2619/(cid:2)2015ElsevierLtd.Allrightsreserved.
B.Yanetal./AppliedEnergy154(2015)40–50 41
excess electricity and stored in underground caverns. It is then 2.5mm,5mmand10mmspacingbetweenplatesandaluminum
combined with natural gas in a gas turbine to boost combustion foamswith10and40poresperinch(PPI).Thesewerechosento
efficiency. As a storage device, its efficiency is typically less than span a range of surface area augmentation types, to utilize com-
50%[5].Inrecentyears,isothermalCAEShavebeenproposedboth merciallyavailablematerials(foams),andbasedondemonstrated
byindustryandacademiaasanenergystorageapproachthatdoes advantages from previous computational studies (interrupted
not require fuel [6,7]. Here, mechanical work is consumed in the plates) [24]. The input/output work, heat transfer and change in
compressortocompressairduringthestoragephase,andrecuper- internalenergyoftheair,andcompression/expansionefficiencies
ated in the expander as the compressed air expands during the arecalculatedduringcompression/expansionforcaseswithporous
regenerationphase. inserts and compared with a benchmark case without porous
For isothermal CAES to be viable, a high pressure (e.g. 200– inserts. This is the first experimental study of a compressor/ex-
300bar), powerful and efficient compressor/expander is critical. panderwithheattransferinsertsandaliquidpistonthathasbeen
Such a compressoris also needed for compressing natural gas as conducted with precise documentation of the volume, pressure
fuel for vehicles. Conventionally a high pressure gas compressor and bulk temperature of the air during compression and expan-
consists of multiple stages with inter-cooling. This creates a sion.Theresultsshow,indeed,thatporousmediainsertscansig-
zig-zag pressure–volume curve consisting of successive adiabatic nificantly improve the trade off between efficiency and power
andconstantvolumecoolingsegments.Acompressorwithmulti- densityinaliquidpistoncompressor/expander.
pleadiabaticstagesandidealinter-coolingisthemostefficientif The remainderofthe paperis structuredas follows. Section2
allstageshavethesamecompressionratio[8].Asthenumberof provides an overview of the liquid piston compressor/expander
stages increases, compression approaches the most efficient and the governing equations that describe it. Section 3 presents
isothermalcompression.Whereasefficiencyofthecompressor/ex- thetestfacilitiesanddescribestheporousmaterialsthatareused.
panderisgovernedbythepressure–volume(P–V)curve,thetimeit Section4describesthetesting,dataanalysisanduncertaintyanal-
takestotracethecurveandhencethepower(work/time)depends ysis.Section5presentstheresultsforthecompressionandexpan-
on the heat transfer rate in the compressor/expander or in the siontests.Sections6and7containdiscussionandconclusions.
inter-cooling.Iflesstimeisallowedforheattransfer,theP–Vcurve
deviates more and more from isothermal and becomes less and 2.Liquidpistoncompression/expansionprocesses
less efficient. Hence, there is an inherent trade-off between effi-
ciencyandpowerofacompressor/expander. Inaliquidpistonaircompressor/expander,airentersandexits
This trade-off can be mitigated by optimizing the compression a compression/expansion chamber from the top, and liquid
andexpansiontrajectories,orbyimprovingtheheattransfercapabil- (water in our case) from the bottom. The volume of the liquid
ity.In[9–11],trajectoriesareoptimizedforthreecases:whenh(cid:3)A inthechamberiscontrolledbyahydraulicpump/motor.Incom-
(the product between the heat transfer coefficient, h, and the heat pression mode, the compression/expansion chamber is initially
transfersurfacearea,A)isaconstant, whenh(cid:3)A isvolumedepen- filled with air at ambient pressure. As liquid is pumped into the
dent, and when h(cid:3)A is an arbitrary function. By matching power chamber,thevolumeofthe airaboveitis reducedand thepres-
andavailabilityofheattransfercapability,several-foldimprovement sure is increased. In expansion mode, the chamber is initially
inpowerdensitiesoveradhoc(sinusoidalorlinearcompression/ex- filled with mostly liquid and a small volume of compressed air
pansion) profiles at the same efficiency can be achieved. Optimal abovetheliquid.Asliquidisallowedtoexitthechamber,thevol-
volume-timetrajectoriesatlowpressureratios(1barto7bar)have ume of the compressed air increases and pressure is reduced. As
beenvalidatedexperimentallyin[12,13].Theeffectivenessofusing such, the liquid column acts as a piston and mechanical work is
tunedcompressiontrajectoriesisalsoshowninsimulationin[14]. applied and extracted via the hydraulic pump/motor for the liq-
Heat transfer capability can be enhanced by actively spraying uid piston. The compression/expansion cycles can be repeated
verysmallliquiddropletswithhighheatcapacityandlargetotal (frequently, if high power is desired) with the intake of ambient
heat transfer surface area [15–18]. The approach pursued in this air or the exhaust of expanded air. With the liquid piston, the
paper is the use of a liquid piston in a compression/expansion chamber can be filled with a porous medium since both liquid
chamberfilledwithporousmaterial,asproposedin[19].Theliq- and air can flow through it. This can significantly increase the
uidpistonfreelyflowsthroughtheporousmaterialwhichgreatly surface area for heat transfer for a given amount of initial/ex-
increases the heat transfer area. Compared with a rigid piston, a panded air volume.
liquid piston also forms an effective low friction seal for the air In this paper,airis treated as an ideal gas since pressures are
beingcompressed.InadditiontotheCAESapplication,liquidpis- relatively low (1–12bar under compression) and temperatures
ton is also being pursued as a cost-effective and efficient means are modest (200–450K). This assumption allows a mass-average
tocompressnaturalgasforvehicleuse[20],aswellasforhydro- bulktemperaturetobederivedfromameasuredpressureandvol-
gen[21]andsupercriticalCO [22].Numericalsimulationsoffluid ume.Anadditionalassumptionisthatthechamberandwaterlines
2
flowandheattransferwereconductedforvariousgeometries(tiny arerigidandthewaterisincompressible.Thispermitsthevolume
tubes, metal foams and interrupted-plate heat exchangers) in ofairinthechambertobedeterminedbythedifferencebetween
[19,23–25]. Preliminary experimental studies of enhanced heat theinitialairvolumeandtheamountofliquidintroduced.
transferwiththeuseofinsertsarereportedin[26].However,since Let P and T be the ambient pressure and temperature. We
0 0
the instrumentation was limited, only limited qualitative infer- assumethatthecompressedairistobestoredinatankatthecon-
encescouldbemade.Recently,aliquidpistoncompressorwasalso stantpressureofrP (risthecompressionratio)andthatthereis
0
demonstratedinabenchtopoceancompressedairenergystorage sufficientdwelltimeforthecompressed airin thestoragevessel
(OCAES)system[27]. tocooldowntoT beforereusing.Theworkinputforthecompres-
0
Theobjectiveofthispaperistotestexperimentallytheeffec- sionprocessandworkoutputfromtheexpansionprocessareillus-
tiveness of various porous media to increase the efficiency or trated as the horizontally-shaded areas under the P–V curves in
power density of a liquid piston compression/expansion process. Fig. 1. The work input to compress V volume of ambient air at
0
Aseriesofcompressionandexpansionexperimentsusingdifferent ðP ; T Þtobestoredinthetankconsistsofthecompressionwork
0 0
typesofporousinsertsinalow-pressure(12barmaximumpres- to rP along a certain compression trajectory (f) defined by the
0 c
sure) compression/expansion chamber are presented. The porous P–V (or T–V) profile, and the isobaric ejection of the compressed
inserts under study are ABS plastic interrupted-plates with airintothetank:
42 B.Yanetal./AppliedEnergy154(2015)40–50
Inadditiontoefficiency,powerdensityisalsoanimportantfigure
of merit for a compressor/expander. The storage power of a com-
pressorisdefinedastheratioofthestorageenergyðE Þtothecom-
P
pressiontimet .Theoutputpowerofanexpanderisdefinedasthe
c
ratiooftheoutputwork(W )totheexpansiontimet .Thecom-
out e
pression/expansionpowerdensitiesaretherespectivepowersnor-
malizedbythecompressor/expandervolume,whichconsistsofthe
expandedairvolumeV andthesolidvolumeoftheporousinsert
0
V :
ins
E
q :¼ p ð6Þ
c t ðV þV Þ
c 0 ins
W
q :¼ out ð7Þ
e t ðV þV Þ
e 0 ins
A compressor/expander with a larger power density is advanta-
geous as smaller or fewer units are needed for a given power
requirement,so thatsystemismorecompactandthecapitalcost
islower.
Thetemperaturedynamicsofafixedmass(m)ofairisgivenby
thefirstlawofthermodynamics:
mcvdTðtÞ¼(cid:4)PðtÞV_ðtÞ(cid:4)Q_ðtÞ ð8Þ
dt
wherecv istheconstantvolumespecificheatcapacity,VðtÞisthe
instantaneousairvolume,andQ_ðtÞistheheattransferrateoutof
the air (to the environment assumed to be at T ). To achieve the
0
isothermal condition, it is necessary that the heat transfer equals
inputpower:
Q_ðtÞ¼(cid:4)PðtÞV_ðtÞ¼Power ðtÞ ð9Þ
input
AssumingNewton’slawofcooling:
Q_ ¼hAðT(cid:4)T Þ ð10Þ
0
Fig.1. Pressure–volumecurvesforisothermal,adiabatic,andrealprocesses.Top:
wherehAistheproductoftheheattransfercoefficientandsurface
compression;bottom:Expansion.
area,thenwehave
W ðf Þ¼ðrP (cid:4)P ÞV (cid:4)Z VcðP(cid:4)P ÞdV ð1Þ mcvdT¼(cid:4)PdV(cid:4)hAðT(cid:4)T0Þdt ð11Þ
in c 0 0 c 0
V0 Rearranging,wehave
wherethesecondtermandV aredependentonf.
c c mcvdTþPdV
Similarly,theworkoutputfromexpandingavolumeV ofcom- (cid:4) ¼hA(cid:3)dt ð12Þ
c ðT(cid:4)T Þ
pressedairfromðrP ; T ÞtoP consistsoftheisobaricinjectionof 0
0 0 0
the compressed air to the expansion chamber and the expansion Eq.(12)showsthatifhAcanbeincreaseduniformlyateachairvol-
work to P0 along an expansion trajectory (fe) defined by the P–V umeVsuchthathA!ahA,thenthesolutionto(12)willbescaled
(orT–V)profile: asPðtÞ!PðatÞ;TðtÞ!TðatÞandVðtÞ!VðatÞ.Hence,thesameP–V
Z Ve and T–V profiles can be accomplished faster by a factor of a, i.e.
W ðf Þ¼ðrP (cid:4)P ÞV þ ðP(cid:4)P ÞdV ð2Þ t !t =a.Thisisachievedbyscalingtheliquidpistonflowrate
out e 0 0 c 0 c=e c=e
Vc bya((cid:4)V_ðtÞ!(cid:4)aV_ðatÞ).Withthedecreaseincycletimes,t ort ,
c e
whereVeisthevolumeatwhichtheexpandingairreachesambient from(6),(7),thepowerdensitieswillalsobeincreasedbyafactor
pressure,andVe andthesecondtermaredependentonfe. ofa.
For a compressed air volume of Vc¼V0=r at pressure rP0, we Thisisthebasisforourhypothesisthatbyintroducingporous
definethestoredenergyasthemaximumworkthatcanbeextracted media which increase the heat transfer surface area A, the com-
given an ambient environmental temperature of T0. This can be pression/expansiontimeswillbereducedandthepowerdensities
obtainedeitherfromWin orWout whenthecompression/expansion willbeincreasedwhilemaintainingthesameefficiency.Similarly,
processisisothermalatT0.ThestoredenergyEP isgivenby: at the same power-density, it is expected that increasing A will
Z V0 increase efficiency. The objective of this paper is to test this
E ¼ðrP (cid:4)P ÞV þ ðP(cid:4)P ÞdV ¼P V lnðrÞ ð3Þ hypothesisexperimentally.
P 0 0 c 0 0 0
Vc
|fflfflfflfflfflfflfflfflfflfflfflffl{zfflfflfflfflfflfflfflfflfflfflfflffl}
isothermal 3.Experimentalsetup
Thus, we can define the compression and expansion efficiencies
respectivelyas: Figs.2and3showaschematicandpictureoftheexperimental
setup.Thesetupisdesignedtoallowtheliquidflowinandoutof
E
g :¼ P ð4Þ the compression/expansion chamber to be controlled and mea-
c W
in sured. Flow rate control permits the compression/expansion tra-
W
g :¼ out ð5Þ jectoriestobecontrolledsothatoperationoverarangeofpower
e EP densitiescan be explored. Instantaneousair volume is calculated
B.Yanetal./AppliedEnergy154(2015)40–50 43
compressor/expander so that the flow rate is determined by the
pressure drop across the valve and the orifice area. By adjusting
the orifice area from run to run, the average flow rate during
expansionisadjusted,buttheflowrateisnotconstant.
An advantage of conducting experiments with constant flow
rates (as in compression) is that between any two experiments,
the air volume trajectories V ðtÞ and V ðtÞ are related by a time
1 2
scaling,i.e.thereexistsascalarasuchthat
V ðtÞ¼V ðatÞ; V_ ðtÞ¼aV_ ðatÞ
1 2 1 2
Aswasnotedearlier,thesolutiontoEq.(12)hasthistimescaling
property if the scaling hA!ahA is applied uniformly. The orifice
determinedflowprofiles(asinexpansion),however,donotenjoy
thispropertysothatthescalinganalysisisnotasexact.Forexam-
ple, the air volume trajectories V ðtÞ and V ðtÞ for a 6s and 3s
1 2
expansiontimesarenotrelatedbyV_ ðtÞ¼2V_ ð2tÞ.
1 2
An Omega FTB-1412 turbine flowmeter (7), with a measure-
ment range of 46.7–466.7cc/s, and a load cell (10), which mea-
sures the weight of water that has left the expansion chamber,
areusedtomeasuretheflowenteringorexitingthecompression/
expansionchamber.Athighflowrates,theturbinemeterisused
(theloadcellmeasurementisinaccurateinthiscaseduetofluid
Fig.2. Schematicoftheliquidpistoncompression/expansionexperimentalsetup.
bysubtracting/addingtheamountofwaterthathasentered/exited
thechamberfromtheinitialairvolume.Airpressureismeasured
directlywithapressuretransducer.Withairvolumeandpressure,
bulkairtemperaturecanbecalculatedfromtheidealgaslaw.This
avoidstheuseofthermocouplesthatcanmeasureonlylocaltem-
perature and because of theporous media, are restricted in their
placements.Compression/expansionworks(W andW )canalso
in out
becalculatedfromairvolumeandpressuretrajectories.
The compression/expansion chamber (1) is a 353mm long,
50.8mm internaldiametertransparentpolycarbonatecylinder.It
can accommodate various porous media inserts. The empty
volumeofthechamberis715cc.Theaspectratioandvolumewere
determinedbasedoncommerciallyavailablesizesandflowcapa-
bilities of the pump/valve to reach reasonable power densities
(relatedtocompression/expansiontimes).Graduationsonthetube
permitinitialandfinalwaterlevelstoberead.Aluminumendcaps
at the top and bottom of the compression/expansion chamber
serve as manifolds for making various connections. The top cap
holds a Kulite ETM-375 pressure transducer (11) for air pressure
measurement and a port (12) for charging the chamber with
compressed air prior to expansion experiments. The bottom cap
provides ports for liquid to enter and exit the chamber.
Elastomeric O-rings are used at the end caps for sealing and tie
rodsareusedtoholdthecylinderagainstthetwoendcaps.
When operating in compression mode, a Wanner Hydra-cell
positivedisplacementdiaphragmpump(3)supplieswatertothe
circuit. The pump is capable of supplying a maximum flow rate
of275cc/s.Areliefvalve(5)isusedtodivertaportionofthisflow
back to tank. This fraction is set by controlling the pressure
upstreamofthesolenoidcontrolvalve(6).Whenthatpressureis
held constant, the flow through the relief valve to the tank and
theflowintothecircuitareconstant.Aproportional–integralcon-
trollerisusedtoregulatethisupstreampressure,measuredwith
pressure sensor (4), to a specified level. When operating in com-
pressionmode,theflowrateisadjustableandheldconstantfora
givenexperiment.
When operating in expansion mode, flow control is more
limited. A needle valve (9) is placed downstream of the Fig.3. Pictureofthecompression/expansionchamber.
44 B.Yanetal./AppliedEnergy154(2015)40–50
areatT (cid:5)300K.Forcompression,constantflowratecompression
0
experiments from 1bar pressure and T temperature to 10bar
0
10 mm ABS 5 mm ABS 2.5 mm ABS 10 PPI 40 PPI were conducted at various compression times and with different
Interrupted Interrupted Interrupted Aluminum Aluminum
Plate Plate Plate Foam Foam inserts.Forexpansion,expansionexperimentsfrom12barandT0
to2barareconductedforarangeofexpansiontimesandwithdif-
ferentinserts.Sinceexpansionflowratescannotbeactivelycon-
trolled, expansion times are varied by adjusting the downstream
orifice for each run. The orifice opening area is constant during
eachrun,however.
Baseline tests with no porous media inserts were conducted
first.Forcompression,16experimentalrunsatdifferentflowrates,
andhencepowerdensities,wereperformed.Ambientpressureand
Fig.4. Fivetypesofporousinsertsusedincompression/expansionexperiments.
temperaturewereusedasinitialconditions.Theinitialairvolume
was typically 715cc. Exceptions were the shortest time, highest
inertialforce);atlowflowrates,theloadcellisused(theflowrates power density cases when a smaller initial volume (410cc) was
areoutofrangeoftheturbinemeter). used.Thisisduetolimitedpumpflowcapability.Effectsofdiffer-
Thecompression/expansionchambercanbesafelypressurized encesininitialvolumearefactoredoutbyconsideringpowerden-
upto12bar.Forcompressiontests,airiscompressedfromambi- sityinsteadofpower.Thedatatakenwhenthepressureisabove
ent pressure to a pressure ratio of 10. For expansion tests, com- 10bar werediscarded. Powerdensities ranging from 3kW/m3 to
pressed air at approximately 12bar is expanded to a final 180kW/m3weretested.Forexpansion,6experimentalrunsatdif-
pressure of approximately 2bar. A pressureratio of 6 is used for ferent expansion times were performed by adjusting the down-
all expansion tests. Thus, P ¼1 bar for compression, and stream orifice for each run. Initial pressure of 12bar, ambient
0
P ¼2barforexpansion.Thissetupiscapableofcompressionrates temperature and initial air volume of (cid:5)100cc were used in all
0
from 46.7cc/s to 275cc/s and (peak) expansion rates up to cases.Thedatatakenwhenthepressureisbelow2barweredis-
275cc/s. carded. Power densities ranging from 29kW/m3 to 223kW/m3
Thecompression/expansionchamber(1)canaccommodatevar- weretested.
iousporousmediainserts.Fivedifferentporousinsertsareconsid- Testing with porous media inserts proceeded similarly as for
ered in these tests: two metal foams and three interrupted-plate the baseline cases and over a similar range of power densities.
styles, as shown in Fig. 4. Table 1 compares some of the insert For compression, all five porous media inserts shown in Fig. 4
properties. The metal foams used in these studies are open-cell andTable1weretested.Theentirechamberwasfilledwithporous
aluminumfoamswith40poresperinch(PPI)and10PPI(Duocel media. Accounting for the insert volumes, the initial air volumes
foamsfromERGAerospaceCorp.Oakland,CA).Thesefoamshave were 600–670cc., except for the highest power density cases,
anominalporosity(i.e.theproportionofvoidvolumetototalvol- which were 350–460cc. For expansion, only the interrupted-
ume)of93%.Theinterrupted-platesaremodeledafterthedesign plateinsertswith2.5mmand5.0mmplatespacingweretested.
proposedin[24]andarefabricatedinABSplasticusinga3Dprint- Also, a small gap was left at the top of the chamber so that the
ingprocess.Theinterruptedplatesdisruptandrestartthebound-
arylayerssothatheattransferisenhanced.Theiropenstructures
enablelow liquidflowdragand low tendencytotrap water.The
threeinterrupted-platedesignshaveanominalplatethicknessof
0.8mm and a nominal plate length of 7.5mm. The inter-plate
spacings of the three inserts are 2.5mm, 5.0mm and 10.0mm.
Fig.5comparesthetotalsurfaceareafoundinthecompressor/ex-
panderwhenthepistonisatbottomdeadcenter.Sincetheinserts
aretobeplacedinthechamberuniformly,exceptforminoreffects
oftheendcapareas,thedifferentinsertswouldhavetheeffectof
scalingtheheattransfersurfaceareasnearlyuniformly.
4.Methods
4.1.Testingmethods
Totesttheeffectofporousmediainsertsontheefficiencyand Fig.5. Totalsurfaceareaavailableattheonsetofcompression.Thisareainclude
powerdensityinliquidpistoncompressionandexpansion,thefol- thetopcapandliquidpistonsurface.
lowingtestswereperformed.Theambientandinitialtemperature
Table1
Detailedpropertiesofporousinserts.Cellsizecorrespondstohydraulicdiameter.Specificareaistheratioofsurfaceareatovolume.Dataforthemetalfoamsareprovidedbythe
manufacturer(ERGAerospace,OaklandCA)anddatafortheinterruptedplatesarecomputedfromCADmodels.
Media Inserttype Material Cellsize(mm) Specificarea(m(cid:4)1) Porosity(%)
40PPI Metalfoam Aluminum 0.5 1732 92
10PPI Metalfoam Aluminum 2.0 709 93
2.5mm Interrupted-plate ABS 2.5 655 81
5mm Interrupted-plate ABS 5.0 377 88
10mm Interrupted-plate ABS 10.0 203 86
Baseline No-insert Polycarbonate 50.8 78.7 100
B.Yanetal./AppliedEnergy154(2015)40–50 45
Table2
Uncertaintyinexperimentalmeasurements.
Measurement Instrument Measuringrange Measurementuncertainty
Pressure ETM-375 0–17bar ±0.1%ofFullscale
Flow FTB-1412 140–467cc/s ±0.6%ofReading
Flow FTB-1412 47–140cc/s ±1.0%ofReading
Flow Loadcell 0–100g/s ±0.5%ofReadingatsteady-state
Initialairvolume Graduatedcylinder N/A ±2cm3(baseline)
±2.8cc(withInserts)
Table3
Uncertaintyinefficiencyfortheoreticalcompressionandexpansiontrajectories.
Baseline 2.5mm 5.0mm 10.0mm 10PPI 40PPI
Errorinisothermalcompressionefficiency ±1.5% ±1.7% ±1.6% ±1.6% ±1.6% ±1.6%
Errorinadiabaticcompressionefficiency ±0.9% ±1.0% ±0.9% ±0.9% ±0.9% ±0.9%
Errorinisothermalexpansionefficiency ±1.2% ±1.2% ±1.2% N/A N/A N/A
Errorinadiabaticexpansionefficiency ±0.4% ±0.4% ±0.4% N/A N/A N/A
initialairvolumecouldbereadfromthegraduationsonthecham- 5.Results
ber.Initialairvolumeswere(cid:5)100cc.Onlydatatakenatpressure
below 10bar (for compression) and above 2bar (for expansion) 5.1.Typicalflowprofiles
areusedfordataprocessing.
Further details of the experimental setup and data processing The typical flow profiles for compression and expansion are
canbefoundin[28]. shown in Fig. 6. As expected, the flow rates are nearly constant
for the compression tests, as maintained by active control. For
4.2.Datareductionmethods expansion,becauseafixedorificeisusedforeachtest,theflowrate
decreasesaspressuredecreases.Ideally,themaximumflowshould
Liquid flow rates and pressures are obtained using the occur at the beginning. However, because the on/off valve takes
Matlab/xPC Target Real-Time System (Mathworks, MA) and a sometimetofullyopen,theflowrateincreasescontinuouslyfrom
16-bitdataacquisitioncardatasamplingrateof1kHz.Airvolume zerotoamaximumflowinstead.
iscalculatedfrom:
Z t
VðtÞ¼V0(cid:4) FlowðsÞds ð13Þ 5.2.Pressure–volumeandtemperature-volumeprofiles
0
whereV0istheinitialairvolumeandFlowðsÞistheliquidflowrate. 5.2.1.CompressionP–V/T–V
Bulkairtemperatureisobtainedfromtheidealgaslaw: Thepressure–volumeandtemperature–volumeprofilesforthe
baseline compression tests with different compression times are
TðtÞ PðtÞVðtÞ
¼ ð14Þ shown in Figs. 7 and 8. As compression time decreases, the P–V
T P V
0 0 0 and T–V profiles tend from being close to the isothermal profiles
Theworkinput,Win,workoutput,Wout,efficiencies,gc andge and towardstheadiabaticprofiles.
power-densities,q andq ,areobtainedfromnumericalintegration Typicaleffectsofdifferentinsertsonthepressure–volumeand
c e
oftheP–Vtrajectory,andfromEqs.(1)–(7). temperature–volumeprofilesforthecompressiontestsoversimi-
lartimesareshowninFigs.9and10.Curveswithothercompres-
4.3.Uncertaintyanalysis siontimeshavesimilartrends.Theseshowthattheintroductionof
inserts shifts the profiles towards the isothermal profiles. It is
Theuncertaintiesofthemeasuredvariablesaresummarizedin apparent that the 10mm and 5mm interrupted-plates are the
Table2.Pressure,volumeandtemperatureareessentialinthecal- leasteffectiveinserts.
culationofefficiencyandpower.Ofthese,pressureisknownwith Atcompressiontimeof2s,insertsreducepeaktemperatureby
themostcertainty,asitismeasureddirectly.Standarderrorprop- 90–120K (57–75% of the temperature rise in the baseline empty
agation analysis shows that air volume and temperature are cylindercase). ComparisonbetweenFigs.8and 10showsthatto
knownwithsomewhatlesscertainty.Forexample,theworstcase limittemperaturerisetoT =10withoutanyinserts,acompression
0
pressure error is at the initial (ambient) pressure (1.7%, time of 60s is needed. However, with the use of inserts (40PPI,
(cid:6)0:017bar)andtheworstcasevolumeerrorisattheendofcom- 10PPIfoams,and2.5mminterrupted-plates),compressiontimes
pression(6.1%,(cid:6)4:3cc). canbereduced30timesto2s,hencesignificantlyimprovingcom-
To estimate the impact of measurement uncertainties on the pactness.WhilethegeneraltrendoftheT–Vprofilesisthattem-
estimate of efficiencies, adiabatic and isothermal compression perature increases monotonically as volume decreases, at small
andexpansionprocessesareconsidered.Theserepresenttheslow- volume there are some small deviations. These deviations are
estandfastestcaseswithefficienciesthatcanbecalculatedanalyt- likelyduetotheslightdropinflowattheendofthecompression
ically,andthuspresentgoodboundsonthepossibleuncertainties. cycle (for the no-insert 2s case in Fig. 8). The increase in heat
By perturbing these trajectories by the estimated errors in pres- transfersurfaceareatovolumerationeartheendofcompression,
sure, volume and temperature, uncertainties in efficiency esti- sincethecapandliquidpistonsurfaceareasareunchangedwhen
mates are obtained. Results are summarized in Table 3. thevolumesaresmall,andtheeffectofwaterevaporatingastem-
Uncertainty values in efficiency are highest when the process is peratureincreasesarealsopossiblereasons.Theseeffectsbecome
closetoisothermal(whenflowrateislow),butuncertaintyvalues moreapparentwheninputworkisrelativelysmall(lowcompres-
areroughlythesameforthedifferentinserts. sionratesorgoodheattransfer).
46 B.Yanetal./AppliedEnergy154(2015)40–50
300 1.5
no insert 2s
no insert 6s
no insert 14s
250 2s compression time 1.4 no insert 23s
s) no insert 60s
3/
m200 2s
c 1.3
e ( 6s compression time 6s
at
n R150 T0 23s
ssio 14s compression time T(t)/1.2 14s
e
mpr100
o 1.1
C
23s compression time
50
1 60s

0 5 10 15 20 25
Time(s) 0.9
0 0.2 0.4 0.6 0.8 1
V(t)/V0
300
Fig. 8. Temperature–volume profiles for baseline compression cases without
2s expansion time insertsatvariouscompressiontimes.
250
3m/s)200 4s expansion time 10
c
e (
at 5s expansion time
R150
on 5
Expansi100 7s expansion time e ratio I4s0opthpei r2msal
ur 10ppi 2s
s
50 Pres 25.m5mm mp laptlaet e2 s2s
2
10mm plate 2s
0 no inserts 2s
0 1 2 3 4 5 6 7 8
Time(s) Adiabatic
Fig.6. Typicalcompression(top)andexpansion(bottom)flowrateprofiles. 1
0.1 0.2 0.5 1
V(t)/V0
10
Fig. 9. Pressure–volume profiles for compression cases with different inserts at
Adiabatic similarcompressiontimesof2s.
no insert 2s
Increasing time no insert 6s
no insert 14s 1.6
5 no insert 23s
no insert 60s
1.5 No insert
Isothermal
o 2 sec compression
e rati 1.4
ur
ess 0 1.3
Pr 2 T(t)/T 1.2 10mm5 mm
1.1
12.5 mm
10ppi 40ppi
10 .1 0.2 0.5 1 0.90. 1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1
V(t)/V0 V(t)/V0
Fig.7. Pressure–volumeprofilesforbaselinecompressioncaseswithoutinsertsat Fig.10. Temperature–volumeprofilesforcompressioncaseswithdifferentinserts
various compression times. Isothermal and adiabatic profiles are added for atsimilarcompressiontimesof2s.
comparison.
processes with constant flow rates follow, nearly, a polytropic
ItisinterestingtonotefromFigs.7and9thattheP–Vcurvesfor process:
all compression times, with and without inserts, are close to
straight lines on a log–log scale. This indicates that compression PðtÞVkðtÞ¼constant
B.Yanetal./AppliedEnergy154(2015)40–50 47
wherethe(cid:4)kistheslope.Forisothermalandadiabaticprocesses,k 1
is1andtheratioofthespecificheats.SinceinputworkW andeffi-
in 5/6 Isothermal
ciencyg becomefunctionsonlyofthepolytropicindex,thissimpli-
c 2.5 mm 7s
fication allows us to compare, according to (12), different 2/3 Adiabatic 5.0 mm 7s
compressionprocesseswithdifferentcompressiontimesanddiffer- No Insert 8s
entinsertscasesthathavethesameefficiencies. 2.5 mm Adiabatic
1/2
P0 5 mm
5.2.2.ExpansionP–V/T–V P(t)/
Thepressure–volumeandtemperature–volumeprofilesforthe
1/3 Isothermal
expansiontestsareshowninFigs.11–14.Inallcases,asevidenced
inboththeP–VandT–Vcurves,theinitialportionofexpansionis
close to the adiabatic process, whereas the latter portion tends No insert
towardtheisothermalprocess.Thisshapeisaconsequenceofflow
ratesdecreasingaspressuredecreases(Fig.6-bottom).Forsuchflow
profiles,expansionworkislargeinthebeginningandmuchsmaller 1/61 2 3 4 5 6
V(t)/V
towardsthelatterportionoftheexpansion.From(8)and(9),high 0
heattransferratesarenecessarytomaintaintheairtemperature,ini-
Fig. 13. Pressure–volume profiles for expansion cases with different inserts at
tially.Itiseasiertodosotowardstheendofexpansionduetothe
similarexpansiontimesof(cid:5)7s.
lowerpowerandlargerexposedsurfaceareaforheattransfer.
Figs.11–14alsoshowthatforslowerexpansions(longerexpan-
siontimes)andwiththeintroductionofinserts,transitiontothe 1
isothermal process occurs at smaller volumes. It is also apparent 2.5mm 2.4s
0.95 5.0mm 2.4s
that the 2.5mm interrupted-plate insert is more effective than
No Insert 2.1s
the5mminterrupted-plateinserts.ComparisonbetweenFigs.12
0.9
and14showsthattolimittheminimumtemperatureto0:85(cid:3)T
0
T0
T(t)/ 0.85
1
Isothermal 0.8
5/6 Adiabatic
no insert 3s 0.75
2/3 no insert 8s
no insert 16s
0.7
1 2 3 4 5 6
1/2 V(t)/V0
P
P(t)/ Fsiimg.il1a4r.eTxepmanpseiroantutirme–evsoolufm(cid:5)e2p:2rosfi.lesforexpansioncaseswithdifferentinsertsat
1/3
I6s
3s withoutanyinserts,anexpansiontimeof8sisneeded.Withthe
useofinserts,expansiontimescanbereducedmorethan3times
8s
tolessthan2.5s.
Notethatfortheexpansionprocesswiththeorificedetermined
1/61 2 3 4 5 6 flowprofiles,theP–VandT–Vdatadonotfitapolytropicmodel,as
V(t)/V0 intheexpansioncasewithconstantflowprofiles.
Fig.11. Pressure–volumeprofilesforbaselineexpansioncaseswithoutinsertsat
variousexpansiontimes.
5.3.Efficienciesandpowerdensities
1.05 Efficiencies and power densities are calculated according to
no insert 3s
Eqs.(4)and(5)andareplottedagainsteachotherinFigs.15and
no insert 6s 6s
16 for the compression and expansion tests, respectively. Error
1 no insert 8s
no insert 16s bars(estimatedfromSection4.3)indicatelevelsofuncertainties.
Figs. 15 and 16, show that the insert cases are more power
denseatthesameefficiency,andmoreefficientatthesamepower
0.95 16s
T0 density,thanthebaseline(noinsert)cases.Specifically,foracom-
T(t)/ pressionefficiencyof95%,powerdensityisincreasedbyafactorof
0.9 10–37(with10mmplatesand40PPIfoam)atthesameefficiency.
Similarly,forafixedexpansionefficiencyof89%,expansionpower
density is increased by a factor of 3 with the use of inserts. The
0.85 8s 3s inserts can alsobe seen as improving efficiency for a given power
density.Atthepowerdensityof100kW/m3,thecompressioneffi-
ciencywasincreasedfrom78%to96%withtheadditionofporous
0.8
1 2 3 4 5 6 media (40PPI Al foam). At a power density of 150kW/m3, the
V(t)/V0
expansionefficiencywasincreasedfrom83%to90%withtheaddi-
tion of porous media (2.5mm interrupted-plates). These improve-
Fig.12. Temperature–volumeprofilesforbaselineexpansioncaseswithoutinserts
atvariousexpansiontimes. ments areexpected becausethe inserts provide moresurface area
48 B.Yanetal./AppliedEnergy154(2015)40–50
casesinFigs.17and18,respectively.Thisnormalizedpowerden-
sityisalsothemeanpowerpersurfacearea.Byusingthetotalvol-
umetodefinethespecificsurfacearea, thenegativeeffectofthe
inserts occupying more volume (which can affect power density
by 8–20%, depending on porosity)is also factored out. While the
areasofthetopcapandtheliquidpistonsurfaceareincluded,their
contributions are relatively small (except when the volume has
become small). The difference in specific surface areas between
compression and expansion cases is due to the gap between the
topcapandtheinsertsintheexpansioncases.
Inthecaseofcompression,Fig.17showsthataftersurfacearea
isaccountedfor,thecaseswithporousinsertscollapsetoapprox-
imatelythesamecurve.Particularly,at95%efficiency,thenormal-
ized power densities lie between 0.1kW=m2 and 0.16kW=m2,
within the range of data scatter. On the other hand, the baseline
no-insertcaseisnoticeablylowerat0:045kW=m2.Thedifference
isevenmorepronouncedatlowerefficiencies.
Intheexpansioncase,Fig.18showsthattheefficiencyvs.nor-
malizedpowerdensitycurvesforallcases(includingtheno-insert
cases)alsocollapsetoapproximatelythesamecurve.However,the
differencebetweeninsertandno-insertcasesdoesnotseemtobe
significant.
Because the compression processes (with the constant flow
rates) are nearly polytropic, each experiment with the same effi-
ciencywillhavesimilarP–V/T–Vcurves.From(12),thisnormaliza-
tion allows other factors affecting heat transfer beyond surface
area,suchasheattransfercoefficient,h,andheatexchangertem-
perature, T , to be compared. Because the expansion processes
0
(with orifice flow control) are not polytropic, the comparison is
more approximate, as the same efficiency does not necessarily
implythesameP–V/T–Vcurves,andhence,thesameLHSof(12).
Fig.15. Compressionefficienciesvscompressionpowerdensityforvariousinserts
(pressureratio=10).Top:Allcases.Bottom:Expandedviewoftheinsertcases.
Fig.16. Expansionefficiencyvs.expansionpowerdensityforbaselineandinsert
cases(pressureratio=6).
forheattransfer.Theorderofdecreasingeffectivenessoftheinserts
to improve efficiency/power density in compression are: (1)
40PPI-foam, (2=) 10PPI-foam or 2.5mm plates, (4) 5mm plates,
(5) 10mm plates, (6) no-inserts. For expansion, the order is: (1)
2.5mmplates,(2)5mmplates,(3)no-inserts.Theseorderscorrelate
exactlywiththesurfaceareasprovidedbytheseinsertsinFig.5.
5.4.Efficienciesandpowerdensitiesnormalizedbyspecificsurface
area
To further investigate the role of surface area increase in
performanceimprovement,efficienciesare plottedagainstpower
Fig.17. Compressionefficiencyvs.powerdensitynormalizedbyspecificsurface
densitiesnormalizedbythevolumespecificsurfacearea(i.e.ratio
areaforvariousinsertsandcompressiontimes(pressureratio=10).Top:Allcases.
ofsurfaceareatototalvolume)forthecompressionandexpansion Bottom:Expandedviewofhighefficiencyregion.
B.Yanetal./AppliedEnergy154(2015)40–50 49
trajectory optimization, however, since the experiments in
[12,13]wereperformedwithoutinserts.
Whiletheexperimentsinthispaperhighlighttheheattransfer
benefits,especiallytheincreasedsurfaceareaprovidedbythepor-
ous media inserts, other aspects of the porous media need to be
consideredwhenchoosingaporousmedium.In[19],longnarrow
cylinderswereanalyzedforliquidpistonapplications.Preliminary
experiments in [26] have shown that while these provide good
heattransfer,differencesindraginsideandbetweenthecylinders
induce unwelcome water jets. The open cell foams and
interrupted-platesinthisstudyavoidthisissue.Themetalfoams
wereselectedinthisstudyfortheirisotropicpropertiesandhigh
porosity. The interrupted-plates were selected because of their
interruptedboundarylayerfeatures,whichofferedpromisingflow
featuresforheatexchangersandlowerdrag.
Fig.18. Expansionefficiencyvs.powerdensitynormalizedbyspecificsurfacearea Porousmediamaterialsalsohavedifferentthermalconductivities
forvariousinsertsandcompressiontimes(pressureratio=6). and thermal capacitances. The thermal conductivity of the
Aluminium foam (167W/mK) is 1000 times higher than that of
Theseresultssuggestthatsurfaceareaisindeedthemostimpor-
theABSplasticinterruptedplates(0.19W/mK).Resultsinthispaper
tantfactorforincreasingefficiencyandpowerdensity.Thefactthat
suggestthatattherelativelylowpressureratiosof6–10,thesedif-
thenormalizedcurvescollapsetoasinglecurveimpliesthatallthe
ferencesinconductivitiesand capacitances donothavesignificant
inserts have similar effects, if any, on factors other than area that
effects.Forexample,theABS2.5mminterruptedplatesandtheAl
mayinfluenceheattransfer.Astheno-insertcasehasalowernor-
10PPIfoamhave similarsurfaceareas(Table1)butverydifferent
malizedpowerdensitythantheinsertcasesatthesameefficiency,
thermalconductivities.Takingporosityintoaccount,theheatcapac-
theinsertcasesshowgreaterpositiveinfluenceontheseotherfactors
itanceperunitairvolumeoftheABSplatesis2.3timesthatoftheAl
thanno-insertcaseincompression.Apossibleexplanationisthatthe
foam. Yet, theirperformances interms ofefficiency/power density
porousmediaprovidemanysmallfeaturestointerrupttheflowand
trade-off are not discernable from the data scatter in Figs. 15 and
encourage new boundary layers to develop. The small hydraulic
17.Tounderstand whyperformanceis insensitive tothermalcon-
diameters in the porous media also induce higher local velocities.
ductivity, consider the (worst) cases with the fastest flow rates
Bothcancauseheattransfercoefficients,h,toincrease.Anotherpos-
(2s).Usingtheheattransfercorrelationsformetalfoaminvestigated
sibilityisthattheheattransfersurfacesmayhaveheatedmoreinthe
in[25],andfortheinterruptedplatesin[24],itisfoundthattheBiot
no-insertcasethanintheinsertcase,thusincreasingtheT onthe
0 numberisoftheorderof10(cid:4)4fortheAlfoamandrangesfrom0.03
LHSof(12)anddecreasingthetemperaturegradient.
to0.2fortheABSinterruptedplates.Thus,theAlfoamcanreason-
ablybeconsideredasalumpedthermalmassbutthevalidityofthis
assumption is not as clear for the interrupted plates. To further
6.Discussion investigatethisissue,zero-dimensional,two-energy-equations(both
the air and the porous medium exposed to air are considered as
Theresultsdescribedaboveshowclearlythatporousmediacan lumped masses) simulations are performed for the 2.5mm inter-
significantly improve the trade-off between efficiency and ruptedplatecaseusingaslightlymodifiedheattransfercorrelation
power-densityofaliquidpistoncompressor/expander.Theexper- proposedin[24]3andthevolumeprofilesfromtheexperiment.The
imentsalsoshowthattheimprovementcanbeexplainedpredom- predictedtemperatureprofilematchestheexperimentwellwiththe
inantlybytheincreaseinheattransfersurfaceareaintroducedby predicted efficiency (92.98%) matches that of the experiment
theporousmedia.However,thepressureratiosinthesetestsare ((cid:5)93%). The predicted rise in porous medium temperature is only
relatively low compared to the applications of compressed air 0.45K.Whentheplatesareassumedtobe1/4oftheiroriginalthick-
energy storage or compressed natural gas for which pressures of ness of 0.8mm so that the Biot number would be comfortably less
200–300barareexpected.Thus,futureexperimentsmustconsider than0.1,thetemperaturerisebecomes1.8K.Thischangeintemper-
higherpressureratios. ature rise decreases efficiency negligibly (from 92.98% to 92.89%).
Theuseoffamiliesofconstantflowrates(forcompression)and Thesesmallmediatemperatureincreasesareconsistentwiththe2D
orifice flows (for expansion) in the experiments simplifies the CFD simulations for metal foams and ABS interrupted plates in
experimentalconditionstobetterisolatetheeffectsoftheporous [25,29] respectively. This analysis shows that because the thermal
media within the flow control constraint of the experimental capacitanceissufficientlylargecomparedtothethermalenergyand
setup.Asshownin[9–11]analytically,and[12,13]experimentally, theheattransfercoefficientsintheselowpressureratioexperiments,
an optimized flow trajectory can significantly improve perfor- theeffectofthermalconductivitiesisinsignificant.Forlargerpressure
mance of compression/expansion processes. Judicious choice of ratios,forexampleforour2ndstagesetup(7–210bar),theeffectof
trajectories can also mitigate the effect of liquid pressure drop conductivitybothintheaxialandtheradialdirectionswouldbemuch
whilemaximizingoverallperformance[11].Anoptimizedprofile more significant as the thermal energy and the heat transfer coeffi-
typicallyconsistsofsegmentsofhighflowsatthebeginningand cientswillbeatleast1orderofmagnitudehigher.
at the end of the process, and a slow (nearlyisothermal)portion Anotheraspecttobeconsideredisthelikelihoodfortheporous
inthemiddle.Inthisregard,theorificeflowprofilesinexpansion mediatotrapwaterorair.Thisreducesthevolumetricefficiencyof
resemble optimizedprofiles moreso than theconstant-flowpro- the compressor/expander as it prevents the ejection of the com-
files in compression (see Fig. 6). This may explain partially why pressed air and the intake of fresh ambient air. In this respect,
the baseline efficiency in expansion is higher than the efficiency despite their thermal characteristics, the 40PPI and 10PPI metal
incompressionatsimilarpowerdensities,andthatthesubsequent
improvement due to adding porous media is less dramatic in
expansion than in compression. Further experiments are needed 3 ThecorrelationusedisNu¼2þ0:0876(cid:3)Re0:792(cid:3)Pr1=3.Thebiastermin[24]is9.7
to clarify the combined benefits of porous media inserts and insteadof2andtheoreticallyshouldbe1.Themodificationfitsthedatamuchbetter.