Distance Dependence of the Energy Transfer Rate From a Single Semiconductor Nanostructure to Graphene PDF
Preview Distance Dependence of the Energy Transfer Rate From a Single Semiconductor Nanostructure to Graphene
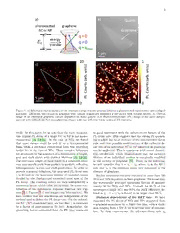
Distance dependence of the energy transfer rate from a single semiconductor nanostructure to graphene Franc¸ois Federspiel,1 Guillaume Froehlicher,1 Michel Nasilowski,2 Silvia Pedetti,2 Ather Mahmood,1 Bernard Doudin,1 Serin Park,3 Jeong-O Lee,3 David Halley,1 Benoˆıt Dubertret,2 Pierre Gilliot,1 and St´ephane Berciaud1,∗ 1Institut de Physique et Chimie des Mat´eriaux de Strasbourg and NIE, UMR 7504, Universit´e de Strasbourg and CNRS, 23 rue du Lœss, BP43, 67034 Strasbourg Cedex 2, France 2Laboratoire de Physique et d’Etude des Mat´eriaux, ESPCI-ParisTech, PSL Research University, Sorbonne Universit´e UPMC Univ Paris 06, CNRS, 10 rue Vauquelin 75005 Paris, France 5 3Advanced Materials Division, Korea Research Institute of Chemical Technology, Daejeon 305-343, Korea 1 The near-field Coulomb interaction between a nano-emitter and a graphene monolayer results 0 in strong Fo¨rster-type resonant energy transfer and subsequent fluorescence quenching. Here, we 2 investigatethedistancedependenceoftheenergytransferratefromindividual,i)zero-dimensional n CdSe/CdSnanocrystalsandii)two-dimensionalCdSe/CdS/ZnSnanoplateletstoagraphenemono- a layer. Forincreasingdistancesd,theenergytransferratefromindividualnanocrystalstographene J decays as 1/d4. In contrast, the distance dependence of the energy transfer rate from a two- 4 dimensional nanoplatelet to graphene deviates from a simple power law, but is well described by 1 a theoretical model, which considers a thermal distribution of free excitons in a two-dimensional quantum well. Our results show that accurate distance measurements can be performed at the ] single particle level using graphene-based molecular rulers and that energy transfer allows probing l l dimensionality effects at the nanoscale. a h Keywords: graphene; semiconductor nanocrystals; quantum dots; semiconductor - nanoplatelets; quantum wells; resonant energy transfer; FRET; single molecule lumi- s nescence; heterostructures; dimensionality e m . Introduction Graphene and colloidal semiconduc- to graphene, resulting in a quenching of the lumines- t a tor nanostructures are model low-dimensional systems, cence signal by more than one order of magnitude, has m which hold promise for opto-electronic applications [1– recently been reported [13]. Related effects have been - 3]. On the one hand, graphene, as a quasi-transparent observed using other types of semiconductor nanostruc- d semi-metal [4, 5] with excellent transport properties [6], tures[14,15,25–27],fluorescentmolecules[28–30]orNV n o can be seen as an ultimate transparent electrode [7, 8]. centers [31, 32]. The observation of robust and efficient c Ontheotherhand,CSNs,intheformofzero-dimensional RET to graphene has stimulated numerous applications [ nanocrystals[9](NCsorquantumdots),one-dimensional in biosensing [33] and holds promise for distance sens- 1 quantumrods[10]andtwodimensionalnanoplatelets[4, ing [34] and photodetection. The case of a single col- v 12](NPs,orquantumwells),areveryefficientbroadband loidal semiconductor nanostructure near a single layer of 1 light harvesting systems and size tunable nano-emitters, grapheneisofparticularinterest,sinceitprovidesawell- 0 which are intensively used in a new generation of light definedandtechnologicallyrelevantsystem,inwhichthe 4 emitting diodes, solar cells and photovoltaic devices [3]. sensitivity of the RET rate to the local environment and 3 0 Thereisagrowinginterestincombininggrapheneand itsdistancedependencecanbeassessedwithaccuracy. In . colloidalsemiconductornanostructuresintheformofhy- addition, RET is known to be strongly affected by exci- 1 0 brid systems [13–15] and devices [16–19] with new func- tondimensionalityandexcitonlocalization[35,36]. Col- 5 tionalitiesandpotentiallyenhancedopto-electronicprop- loidal semiconductor nanostructures offer natural ways 1 erties. The photoresponse of the graphene NC-hybrid to explore such effects. : v system is governed by interface and short-range phe- Here, weinvestigateRETfromi)individualcore/shell i nomena, such as charge transfer and F¨orster-type res- CdSe/CdS NCs and ii) core/shell CdSe/CdS/ZnS NPs X onant energy transfer [20] (RET) (see Figure 1). While to a graphene monolayer. Using molecular beam epi- r photo-induced charge transfer may result in a photogat- taxy, we are able to deposit ultrasmooth dielectric spac- a ing effect and improved photogain [16, 17], energy trans- ers of magnesium oxide (MgO), with variable thickness, fer from a photoexcited colloidal semiconductor nanos- between graphene and the nanoemitters [6]. The scaling tructure (donor) to a graphene layer (acceptor) may effi- oftheRETratewiththedistancedseparatinggraphene cientlygenerateelectron-holepairsingraphene, whichis from the nanoemitters is then quantitatively determined of interest for optoelectronics [2]. Importantly, graphene from the luminescence decays recorded on a collection stands out as a uniquely tunable acceptor system, in of individual emitters. In the case of zero-dimensional which distinct regimes of RET can be observed by vary- NCs, the RET rate scales as 1/d4, as expected theo- ing its Fermi level [21–24]. retically [21, 22, 30, 38–40]. Interestingly, although the Highly efficient RET from individual CdSe/ZnS NCs RET rate of individual two-dimensional NPs adsorbed 2 on bare graphene is similar to that observed with zero- multiplyingchargecoupleddevicecamera(emCCD).PL dimensional NCs, we find that the RET rate decays less time traces and PL decays of individual colloidal semi- rapidly with increasing distance. Such a behavior is dis- conductor nanostructures were measured in a confocal cussedwithintheframeworkofenergytransferfromfree arrangement, using an avalanche photodiode coupled to two-dimensional excitons [41, 42] to a two-dimensional a time-tagged, time-correlated single photon counting acceptor. board. PL spectra were recorded using a monochroma- Methods We have investigated individual colloidal tor coupled to a CCD matrix. A pulse fluence lower semiconductor nanostructures near a graphene mono- than 1 × 1013 photons/pulse/cm2 at λ = 480 nm was layer at room temperature, using a home-built micro- used for all measurements. Considering similar absorp- photoluminescence (PL) setup equipped with a 100× tion cross sections of a few 10−14 cm2 for our individual (NA = 0.90) air objective. Core/shell CdSe/CdS NCs NCsandNPsat480nm[50–52],wecanestimatethaton ((9.5±1.5) nm in diameter, with peak emission at λ = average, significantly less than one exciton per incoming 0 580 nm, i.e., photon energy of 2.14 eV), coated with laser pulse is formed in an individual NC or NP. We fur- oleylamine and oleic acid ligands, and core/shell/shell ther verified that the PL decays of individual NCs and CdSe/CdS/ZnS NPs (4 monolayer thick core, ∼ 1.3 nm NPs are independent on the pulse fluence, in the range thickshell,∼9nmwidth,∼22nmlengthandpeakemis- (≈1012−3×1013 photons/pulse/cm2). sion at λ = 645 nm, i.e., photon energy of 1.92 eV), Energy transfer on bare graphene Figure 1 0 coated with oleate ligands, were synthesized following showswide-fieldPLimagesofindividualCdSe/CdS/ZnS previous works [2, 3, 12, 45, 46] (see also Support- NPs deposited on a bare, mechanically exfoliated ing Information). Using core/shell structures dramati- graphene monolayer. The PL intensity is strongly cally reduces the possibility of irreversible photoinduced quenched, by more than one order of magnitude for NPs NC or NP ionization and subsequent charge transfer to deposited on graphene. Very similar results were ob- graphene [46, 47]. NCs and NPs, dispersed at very low tainedusingCdSe/CdSNCs. Aspreviouslydiscussedfor concentration into a 90%/10% hexane/octane mixture individual core/shell CdSe/ZnS NCs [13], we attribute were then dropcast onto graphene samples. PL quenching to F¨orster-type RET. Measurementsonbaregraphene(seeFigures1–3)were We then compare the typical PL spectrum, PL time performedusingmechanicallyexfoliatedgraphenemono- trace and PL decay of individual NCs (see Figure 2) and layers deposited on transparent fused quartz substrates. NPs(seeFigure3),measuredonafusedquartzsubstrate The distance dependence of the energy transfer rate was andonabaregraphenemonolayer. Foreachnanoemitter investigatedusinglargeareagraphenemonolayers,grown investigated, we introduce the average number of emit- by low-pressure chemical vapor deposition (LPCVD) on ted photons per incoming laser pulse N (see PL time em a copper foil, then transfered onto a fused quartz sub- traces in Figures 2b,e and 3b,e). First, we note that strate using standard methods [5] (see Supporting Infor- although the peak energy of the PL spectra exhibits a mation). Inordertovarythedistancebetweengraphene slightdispersionoveracollectionofnanoemitters,wedid and the nano emitters, thin films of MgO were grown not observe systematic spectral shifts for NCs or NPs on on top of graphene in a staircase fashion in a molecu- graphene with respect to a reference on fused quartz. In lar beam epitaxy (MBE) chamber [6] before deposition both cases, the PL count rates on graphene and fused of NCs or NPs. We checked by atomic force microscopy quartz are similar, but the PL signals are obtained us- that the roughness of the MgO layer is on the order of ing very different repetition rates (compare Figures 2b 0.5 nm [49]. and 2e, and Figure 3b and in Figure 3e, for NCs and The bare graphene and graphene/MgO samples were NPs, respectively). On these selected examples, N is em characterizedusingahome-builtmicro-Ramansetup(see quenched by a factor of approximately 50 (60) when the Supporting Information). Although residual doping on NC (NP) is adsorbed on graphene, as compared to a ref- the order of a few 1012 cm−2 could be observed, the re- erence recorded on fused quartz. Over time scales larger sulting Fermi level shifts relative to the Dirac point are than100ms,wealsoobserve,inagreementwithprevious aboutoneorderofmagnitudesmallerthantheenergyof observations [13, 14] that the blinking behavior, charac- theemittedphotons. Therefore,graphenewillbeconsid- teristic of NCs and NPs deposited on fused quartz, is ered as quasi-neutral in the following. seemingly reduced when the nanoemitters are adsorbed Individual NCs and NPs were excited using a pulsed on graphene. This observation is presumably due to the supercontinuum laser, with a repetition rate tunable acceleration of the excited state decay, which occurs be- from 1.95 MHz up to 78 MHz. The unpolarized out- fore charge carriers may be trapped and allow the obser- put of the supercontinuum laser at a wavelength of vation of dark and/or grey states [46, 53–56]. 480 nm (photon energy of 2.53 eV) was selected us- WenowcomparethePLdecaysofindividualNCsand ing an acousto-optic tunable filter. The full width at NPs measured on fused quartz and on graphene. Exam- half maximum of the filtered pulses was ≈ 50 ps. ples are shown in Figures 2c and 2f for individual NCs Wide field PL images were recorded using an electron- and in Figures 3c and 3f, for individual NPs, respec- 3 a) b) Quartz photoexcited graphene NC or NP Graphene qq=00 energy E q0 0 transfer qq==qq max 5 µm 20 1 c) d) ) ) s s t t i i n n uu uu . . b b r r a a ( ( ss ss t t n n u u o o C C L L L L 5 µm P P 0 0 Figure1. a)Schematicrepresentationoftheresonantenergytransferprocessbetweenaphotoexcitednanoemitterandundoped graphene. Electronic excitations in graphene with various transferred momenta q are shown with colored arrows. b) Optical image of an exfoliated graphene sample deposited on fused quartz. c-d) Photoluminescence (PL) image of the same sample, covered with CdSe/CdS/ZnS nanoplatelets, shown with two different linear scales of PL intensity. tively. At this point, let us note that the room tempera- in good agreement with the enhancement factors of the ture typical PL decay of a single NC or NP is not mono- PLdecayrate. ThissuggeststhatthestrongPLquench- exponential [46, 54–56]. In the case of NCs, we found ingissolelyduetoanincreaseofthenon-radiativedecay that most decays could be well fit to a bi-exponential rate, and that possible modifications of the radiative de- form, while a stretched exponential form was providing cayrateofanindividualNCorNPadsorbedongraphene better fits in the case of NPs. These complex behaviors can be neglected. This is consistent with recent theoret- areattributedtotheexistenceofadistributionofbright, ical calculations, which demonstrated that the radiative grey and dark states with distinct lifetimes [46, 53–56]. lifetime of an individual emitter is marginally modified Thefractionalweightofthesestatesinameasureddecay in the vicinity of graphene [22]. Thus, in the following, mayvarysignificantlyfromparticletoparticle,reflecting we will consider that γ ≈ γ +γ , where γ is the RET t 0 t heterogeneities in core and shell passivation. In order to rate and γ is the reference decay rate measured in the 0 provideageneraldefinition, themeasuredPLdecayrate absence of graphene. γ is defined as the maximum number of recorded counts Similarmeasurementswererepeatedonmorethan100 divided by the (background corrected) total area of the NCsandNPsdepositedonbaregraphene. Wefoundsim- PL decay. The resulting values are then multiplied by a ilar statistically averaged quenching factors of approxi- correctionfactor,whichtakesintoaccounttheminorcon- mately 50 for NCs and NPs. Overall, for 95 % of the tribution of the instrument response function (see Fig- investigatedsingleNCsandNPs,theRETefficiency,de- ures 2c,f,Figures3c,f,andSupportingInformation). We fined as η =1−γ/γ is found to be larger than 95 %. 0 have verified that ourconclusions are independent ofthe Distance dependence of the RET rate We have methodusedtodefinethePLdecayrate. Fortheindivid- measured the PL decay of NCs and NPs separated from ualNC(NP)consideredhere, wefindthatγ isenhanced a graphene monolayer by a MgO thin film, with a thick- by a factor of approximately 50 (80). Remarkably, the ness ranging from a few ˚A up to several tens of nanome- quenching factors estimated from the PL time traces are ters. In these experiments, the reference decay rate γ 0 4 s)units 11 a)) z)0 Hz10 b)) LL=1.9 MHz s)units Quartz c)) b. 10 b. Graphene ar x ar10 (nts s (nts 5 (nts u u u o o o L c L c L c = 0.012 ns-1 PP PP PP 0 0 1 2.0 2.2 0 50 100 0 200 0 200 400 Photon energy (eV) Time (s) Occurrence Time delay (ns) nits) 1 d) Hz)10 e) L=78 MHz nits) f) u 0 u 10 Quartz b. arb 0x1 b. arb10 Graphene nts ( nts ( 5 nts ( ouo ou ouo =00..6600 nnss-1 L c cL c L c 1 P P P 0 5 10 0 0 1 2.0 2.2 0 50 100 0 500 0 200 400 Photon energy (eV) Time (s) Occurrence Time delay (ns) Figure2. a-c)Photoluminescence(PL)spectrum,PLtimetraceandPLdecayofindividualCdSe/CdSnanocrystaldeposited on fused quartz. d-f) Same measurements recorded on an another individual CdSe/CdS nanocrystal deposited on a graphene monolayer. Alldatawerecollectedusingapulsedlaserexcitation,witharepetitionrateΓ indicatedinpanelsb)ande). The L redlinesinc)andf)arefitsbasedonbi-exponentialfunctionsconvolutedwiththeinstrumentresponsefunction(displayedas a gray line in f). is the statistically averaged decay rate of NCs and NPs one would expect the measured decay rate to scale as measuredonabare>100nmthickfilmofMgO.There- (cid:34) (cid:32) (cid:33)p(cid:35) sults for NCs and NPs are summarized in Figures 4 and γ =γ 1+ z0 , (1) 5, respectively. Each point corresponds to a statistical 0 d0+dMgO average over 10 to 30 single NCs, and over more than wherethedistancedseparatingthenanoemitterfromthe 25 single NPs, respectively. The vertical error bars cor- graphenelayerisd=d +d ,withd ,thethickness respond to the standard deviations, while the horizontal 0 MgO MgO of the MgO film and d , the minimal distance between errorbarsaccountfortheroughnessoftheMgOfilm. For 0 the center of the nanoemitter and the graphene surface both NC and NPs, we observe that the measured decay in the absence of a MgO spacer, where z characterizes ratedecreasessignificantly,whenincreasingthethickness 0 theRETefficiency,andpisrelatedtothedimensionality of the MgO film. However, as shown in figures 4c and of the donor and acceptor. A straightforward extension 5c, the product N γ varies by less than a factor of 2 em of F¨orster’s theory would then give p = 4 for a zero- and, consideringthestandarddeviationsassociatedwith dimensional emitter interacting with a two-dimensional each distribution, can be considered as constant. This assemblyofindependentdipoles[38,39]. Indeed,forNCs generalizes the conclusions drawn from the analysis of (see Fig. 4b, using p = 4, we obtain a good fit with Figures 2 and 3. z =(11.5± 1.5) nm and d =(5.5± 1) nm. The latter 0 0 AkeyobservationisthatthedecreaseofthePLdecay valueisslightlylargerthantheaveragephysicalradiusof rateasfunctionoftheMgOthicknessisseeminglysteeper theNCs,whichisconsistentwithapossiblecontribution forNCsthanforNPs(seealsoFigure7intheSupporting from the surrounding ligands and residual adsorbates to Information for a comparison of the normalized decay d . 0 rates). This points towards the effect of dimensionality AlthoughafitbasedonEq.1isingoodagreementwith on RET, which we now discuss. Let us first focus on our measurements, one has to recall that Eq.1 overlooks the case of NCs interacting with graphene. Based on the fact that graphene is a two-dimensional system with the well known 1/d6 distance dependence of the F¨orster extended,delocalizedelectronicwavefunctionsandawell energy transfer rate between two point-like dipoles [20], defined electronic dispersion. The RET rate γ0D from a t 5 s)nits 11 a)) )Hz) b)) LL=33..99 MMHHzz s)nit c)) (arb. unts (x100 nts 25 (arb. unts 10100 Quartz Graphene u u u o o o L c L c L c =0.070 ns-1 PP 0 PP 0 PP 1 1.8 2.0 0 20 40 0 100 0 100 200 Photon energy (eV) Time (s) Occurence Time delay (ns) nits) 1 d) Hz) e) L=78 MHz nits) f) b. ub 00 0 b. ub100 100 Quartz GGraphhene nts (ar nts (x110 nts (ar 10 10 = 5.5 ns-1 u u u o oo o cPL c 0 PL c 0 cPL c 1 0 5 1.8 2.0 0 20 40 0 100 0 100 200 PPhhotton energy ((eVV)) Time (s) Occurence Time delay (ns) Figure 3. a-c) Photoluminescence (PL) spectrum, PL time trace and PL decay of an individual CdSe/CdS/ZnS nanoplatelet depositedonfusedquartz. d-f)SamemeasurementsonanotherindividualCdSe/CdS/ZnSnanoplateletdepositedonagraphene monolayer. All data were collected using a pulsed laser excitation, with a repetition rate Γ indicated in panels b) and e). L The red lines in c) and f) are fits based on stretched exponential functions convoluted with the instrument response function (displayed as a gray line in f). single point-like dipole to graphene has been extensively point-like donor, while in our case, the NCs have a fi- studiedtheoreticallyinrecentyears[21,22,30,40,57,58] nite radius, larger than the thinnest MgO films (0.6 nm) and can be written as [22, 40] deposited here. However, the surrounding ligands and the finite thickness of the CdS shell of our CdSe/CdS NCs warrant that there is a minimal distance of a few (cid:90) qmax dq q3e−2qd nanometers between the graphene layer and the emit- γ0D ∝ , (2) t (cid:112)q2 −q2 ting CdSe core. We will therefore consider that the long 0 max distance approximation is valid and neglect the finite where q is the transferred momentum, q = 2πc size of the NCs and NPs. Considering random relative max λ0vF dipole orientation, we obtain [40] z4 = 3α λ4, is the largest transferable momentum, λ0 is the wave- 0 2048π3F2ε5/2 0 where α ≈ 1/137 is the fine structure constant, ε is the lengthoftheemittedphotons, cisthespeedoflightand v ≈ 1.1×106 m/s is the Fermi velocity in graphene. effective dielectric constant (at λ0) of the surrounding F medium and F = 3ε is a screening factor [59]. Us- Contrary to far-field emission and absorption processes, ε +2ε CdS the RET process described by Eq.2 involves a finite mo- ing ε≈(εMgO+1)/2≈2 results in z0 ≈11 nm, in good mentum transfer (see Figure 1a), which is on the or- agreement with our measurements. der of 1/d. For neutral graphene, and distances such We now address the distance dependence of the RET that d (cid:29) 1/q ≈ 0.3 nm, the denominator of the rate from single NPs to graphene. The NPs cores are max integrand in Eq.2, which originates from the (momen- atomically smooth and have recently been shown to be- tum dependent) optical conductivity of graphene [22], have as genuine quantum wells [12]. As a result, a differ- only gives significant contributions for q (cid:28) q (quasi- entregimeofRETisanticipatedwhenatwo-dimensional max vertical transitions), and can thus be approximated as a donor is involved. Electronic states in NPs are described constant. In these conditions, graphene can be treated by extended wave functions that are coherent over large as a two-dimensional assembly of incoherent point-like distances in the NP plane. However, at finite tempera- dipoles, and Eq.2 simplifies as γt0D ≈ γ0(cid:0)zd0(cid:1)4. Never- ture, one has to consider, in a photoexcited NP, a ther- theless, it must be emphasized that Eq. 2 applies to a mal distribution of excitons with different in-plane cen- 6 11 a) CVD graphene 17.0 nm MgO b) 1 6.0 nm MgO MgO 3.4 nm MgO s) Quartz 2.6 nm MgO 1) nitn 00.66 nnmm MMggOO -sns u ( b. 0.1 r a s ( ntn00.11 u o c ) c) L u. P a. 2 ( m e N 0 0 20 40 60 80 0.1 1 10 100 TTiimmee ddeellaayy ((nnss)) MMggOO tthhiicckknneessss ((nnmm)) Figure 4. a) Selected luminescence decays of individual CdSe/CdS nanocrystals separated from graphene by a MgO spacer graphenewithincreasingthicknesses. Thethinblacklinesarefitsbasedonbi-exponentialdecaysconvolutedwiththeinstrument response function. b) Statistically averaged measured decay rate γ as a function of the thicknesses of the MgO spacer. The red solid line is a fit based on Eq. 1, with p=4, d =5.5 nm, z =11.5 nm. c) Statistically averaged product of the number 0 0 of emitted photons per exciting laser pulse N and the decay rate γ. em a) CVD graphene MgO (no graphene) b) MgO 8.0 nm MgO 1 Quartz 4.0 nm MgO s) 1.5 nm MgO 1) nitn 0.4 nm MggO -sns 11 u ( b. r a s ( 0.1 ntn u 0.1 PL co (a.u.) 2 c) 1 0.01 mm e N 0 0 5 10 15 20 25 0.1 1 10 100 Time delayy ((ns)) MggO thickness ((nm)) Figure 5. a) Selected luminescence decays of individual CdSe/CdS/ZnS nanoplatelets separated from graphene by a MgO spacer graphene with increasing thicknesses. The thin black lines are fits based on stretched exponential decays convoluted with the instrument response function. b) Statistically averaged measured decay rate γ as a function of the thicknesses of the MgO spacer. The red solid and dashed lines are fits based on Eq. 3, with d =3.5 nm, Λ=7.5 nm, and on Eq. 1 with p=4, 0 d =3.5 nm, z =8.0 nm, respectively. c) Statistically averaged product of the number of emitted photons per exciting laser 0 0 pulse N and the decay rate γ. em ter of mass momentum. Besides, since the thickness of systems composed of quantum wells transferring energy the NP shell is on the order of 2 nm, there is a mini- to an assembly (thick film or monolayer) of point-like mal separation between the NP core and graphene, such dipoles. The calculated RET rate writes thattheapproximationd(cid:29)1/qmax holds(i.e.,graphene (cid:90) ∞ can also be treated as an incoherent plane of point-like- γt2D(d)∝ dq q3e−2qde−(Λ2πq)2, (3) dipoles). Similar situations have been previously mod- 0 eled by Basko et al. [41] and Kos et al. [42] for hybrid where Λ = √ h is the de Broglie thermal length, 2m∗k T B 7 with h the Planck constant, k the Boltzmann constant tor nanostructures, which is of interest for photodetec- B and m∗ the mass of the lowest energy heavy hole exci- tion. Amajorchallengeisnowtodissociatetheelectron- ton (m∗ ≈ m , where m is the free electron mass) in hole excitations generated in graphene before their fast e e CdSe [12, 60]. relaxation into heat [61, 62]. This expression applies to free excitons in an infinite Acknowledgement We are grateful to D.M. Basko quantumwell,withthickness(Lz (cid:28)d). Theassumption and G. Weick for fruitful discussions, to R. Bernard, of free excitons is consistent with the reported low den- S. Siegwald and H. Majjad for help with sample fabri- sity of trapping sites of CdSe-based core/shell NPs [46]. cation and characterization in the STNano clean room In addition, since the typical lateral dimensions of our facility, and to M. Romeo for technical support. The NPs (Lx =(22 ±2) nm and Ly =(9 ±1.5) nm) exceed authors at IPCMS acknowledge financial support from Λ (at room temperature, Λ ≈ 7.5 nm), finite size effects the CNRS, Universit´e de Strasbourg, C’Nano GE and canbeneglected. Wehavethusattemptedtofitthedata the Agence Nationale de Recherche (ANR) under grants inFigure5busingtheexpressionγ(d)=γ0+γt2D(d). A QuandDoGra ANR-12-JS10-0001 and Fungraph ANR- goodagreementwithourexperimentalresultsisobtained 11-IS10-0003. B.D. (at ESPCI) thanks the ANR for using d0 = (3.5±1) nm. Again, the latter value is con- funding under grants SNAP and QDOTICS. J-O. L. (at sistentwiththethicknessofthecoreandshelloftheNPs KRICT)acknowledgessupportfromNRF-ANRprogram and includes a minor contribution from the surrounding throughtheNationalRearchFoundationofKoreafunded ligands and residual adsorbates. by the Ministry of education, Science and Technology An analysis of the limiting cases of Eq. 3 provides a (NRF-2011-K2A1A5-2011-0031552). rationale for the observed scaling. In the short distance Supporting Information Synthesis and character- limit, d (cid:28) Λ, γt2D becomes independent on the distance ization of CdSe/CdS nanocrystals and CdSe/CdS/ZnS and is determined by the thermal cutoff. In contrast, in nanoplatelets, graphene growth by chemical vapor de- the large distance (or high temperature) limit, d (cid:29) Λ position, sample characterization by micro-Raman spec- and γt2D follows a 1/d4 scaling, as expected in the case troscopy, definition of the energy transfer rate, compari- of a two-dimensional assembly of incoherent point-like son of the normalized distance dependent decay rates. dipoles. Since, at room temperature, Λ falls exactly in ourmeasurementrange,weexpecttheRETratetodecay more smoothly than 1/d4 for d < Λ. This is indeed ob- served experimentally (see Fig 5b), since a scaling based on Eq. 2, with p = 4 and d = 3.5 nm, having asymp- ∗ [email protected] 0 toticbehaviorforlargedasourfitbasedonEq.3,would [1] F. Bonaccorso, Z. Sun, T. Hasan, and A. C. Ferrari, “Graphene photonics and optoelectronics,” Nature Pho- predict a higher γ in the short distance limit. tonics 4, 611–622 (2010). The results shown in Figure 5 suggest that even at [2] F. H. L. Koppens, T. Mueller, Ph Avouris, A. C. Fer- room temperature, the distance scaling of the RET rate rari,M.S.Vitiello, andM.Polini,“Photodetectorsbased from an extended donor (such as a two-dimensional NP) on graphene, other two-dimensional materials and hybrid to graphene exhibits, as expected theoretically, a slight systems,” Nature Nanotechnology 9, 780–793 (2014). deviation from a simple power law. Following Eq.3, this [3] Dmitri V. Talapin, Jong-Soo Lee, Maksym V. Ko- valenko, and Elena V. Shevchenko, “Prospects of col- deviationisexpectedtobemoreprominentatlowertem- loidal nanocrystals for electronic and optoelectronic ap- peratures. Thus, further investigations of the distance plications,” Chemical Reviews 110, 389–458 (2010). andtemperaturedependenceoftheRETrateforvarious [4] R. R. Nair, P. Blake, A. N. Grigorenko, K. S. Novoselov, NP architectures could provide insights into the dimen- T.J.Booth,T.Stauber,N.M.R.Peres, andA.K.Geim, sionality of excitons in these novel systems [36]. “Fine Structure Constant Defines Visual Transparency of In conclusion, F¨orster-type resonant energy transfer Graphene,” Science 320, 1308 (2008). dramatically affects the photophysics of semiconductor [5] Kin Fai Mak, Matthew Y. Sfeir, Yang Wu, Chun Hung Lui, James A. Misewich, and Tony F. Heinz, “Measure- nanostructures absorbed on graphene. In spite of its ment of the optical conductivity of graphene,” Physical atomic thinness, a single layer of graphene typically Review Letters 101, 196405 (2008). quenches more than 95% of the luminescence of indi- [6] S. Das Sarma, Shaffique Adam, E. H. Hwang, and vidual nanocrystals or nanoplatelets. The observation Enrico Rossi, “Electronic transport in two-dimensional of well-defined distance scalings of the resonant energy graphene,”ReviewofModernPhysics83,407–470(2011). transferratesuggeststhatnovelgraphene-basedmolecu- [7] Xuan Wang, Linjie Zhi, and Klaus Mllen, “Transpar- lar rulers can be engineered using semiconductor nanos- ent, conductive graphene electrodes for dye-sensitized so- lar cells,” Nano Letters 8, 323 (2008). tructures with different size, shape, and dimensionality. [8] Keun Soo Kim, Yue Zhao, Houk Jang, Sang Yoon Lee, Thisisapromisingdevelopment,especiallyforbiological Jong Min Kim, Kwang S. Kim, Jong-Hyun Ahn, Philip research. Finally, with the prospect of designing hybrid Kim,Jae-YoungChoi, andByungHeeHong,“Large-scale opto-electronic devices, we show that graphene can very patterngrowthofgraphenefilmsforstretchabletranspar- efficientlyharvestenergyfromphotoexcitedsemiconduc- ent electrodes,” Nature 457, 706–710 (2009). 8 [9] V.I.EditedbyKlimov,Nanocrystal Quantum Dots(CRC P. Jarillo-Herrero, and F. H. L. Koppens, “Electrical Press, UK, 2010). control of optical emitter relaxation pathways enabled by [10] XiaogangPeng,LiberatoManna,WeidongYang,Juanita graphene,” arXiv:1410.1361 (2014). Wickham, Erik Scher, Andreas Kadavanich, and A. P. [24] Jiye Lee, Wei Bao, Long Ju, P. James Schuck, Feng Alivisatos, “Shape control of CdSe nanocrystals,” Nature Wang, and Alexander Weber-Bargioni, “Switching indi- 404, 59–61 (2000). vidualquantumdotemissionthroughelectricallycontrol- [4] Sandrine Ithurria and Benoit Dubertret, “Quasi 2D col- ling resonant energy transfer to graphene,” Nano Letters loidal CdSe platelets with thicknesses controlled at the 14, 7115–7119 (2014). atomic level,” Journal of the American Chemical Society [25] Eyal Shafran, Benjamin D. Mangum, and Jordan M. 130, 16504–16505 (2008). Gerton, “Energy transfer from an individual quantum [12] S. Ithurria, M. D. Tessier, B. Mahler, R. P. S. M. Lobo, dot to a carbon nanotube,” Nano Letters 10, 4049–4054 B. Dubertret, and Al L. Efros, “Colloidal nanoplatelets (2010). withtwo-dimensionalelectronicstructure,”NatureMate- [26] SebastianJander,AndreasKornowski, andHorstWeller, rials 10, 936–941 (2011). “EnergytransferfromCdSe/CdSnanorodstoamorphous [13] Zheyuan Chen, Ste?phane Berciaud, Colin Nuckolls, carbon,” Nano Letters 11, 5179–5183 (2011). TonyF.Heinz, andLouisE.Brus,“Energytransferfrom [27] T. N. Lin, L. T. Huang, G. W. Shu, C. T. Yuan, J. L. individualsemiconductornanocrystalstographene,”ACS Shen, C. A. J. Lin, W. H. Chang, C. H. Chiu, D. W. Nano 4, 2964–2968 (2010). Lin, C. C. Lin, and H. C. Kuo, “Distance dependence [14] O. A. Ajayi, N. C. Anderson, M. Cotlet, N. Petrone, of energy transfer from ingan quantum wells to graphene T.Gu,A.Wolcott,F.Gesuele,J.Hone,J.S.Owen, and oxide,” Opt. Lett. 38, 2897–2899 (2013). C. W. Wong, “Time-resolved energy transfer from single [28] EmanueleTreossi,ManuelaMelucci,AndreaLiscio,Mas- chloride-terminated nanocrystals to graphene,” Applied simo Gazzano, Paolo Samori, and Vincenzo Palermo, Physics Letters 104, 171101 (2014). “High-contrast visualization of graphene oxide on dye- [15] Beno¨ıt Rogez, Heejun Yang, Eric Le Moal, Sandrine sensitized glass, quartz, and silicon by fluorescence L´evˆeque-Fort,ElizabethBoer-Duchemin,FeiYao,Young- quenching,” Journal of the American Chemical Society Hee Lee, Yang Zhang, K. David Wegner, Niko Hilde- 131, 15576–15577 (2009). brandt, Andrew Mayne, and G´erald Dujardin, “Fluo- [29] Jaemyung Kim, Laura J. Cote, Franklin Kim, and Ji- rescence lifetime and blinking of individual semiconduc- axingHuang,“Visualizinggraphenebasedsheetsbyfluo- tor nanocrystals on graphene,” The Journal of Physical rescencequenchingmicroscopy,”JournaloftheAmerican Chemistry C 118, 18445–18452 (2014). Chemical Society 132, 260267 (2009). [16] Gerasimos Konstantatos, Michela Badioli, Louis Gau- [30] L. Gaudreau, K. J. Tielrooij, G. E. D. K. Prawiroat- dreau,JohannOsmond,MariaBernechea,F.PelayoGar- modjo, J. Osmond, F. J. Garcia de Abajo, and F. H. L. cia de Arquer, Fabio Gatti, and Frank H. L. Koppens, Koppens, “Universal distance-scaling of nonradiative en- “Hybrid graphene-quantum dot phototransistors with ul- ergy transfer to graphene,” Nano Letters 13, 2030–2035 trahigh gain,” Nature Nanotechnology 7, 363 (2012). (2013). [17] Zhenhua Sun, Zhike Liu, Jinhua Li, Guo-an Tai, Shu- [31] Rainer J. Sto¨hr, Roman Kolesov, Kangwei Xia, Rolf PingLau, andFengYan,“Infraredphotodetectorsbased Reuter, Jan Meijer, Gennady Logvenov, and Jo¨rg oncvd-growngrapheneandpbsquantumdotswithultra- Wrachtrup, “Super-resolution fluorescence quenching mi- high responsivity,” Advanced Materials 24, 5878 (2012). croscopy of graphene,” ACS Nano 6, 9175–9181 (2012). [18] Alexander V. Klekachev, Inge Asselberghs, Sergey N. [32] Julia Tisler, Thomas Oeckinghaus, Rainer J. Sto¨hr, Ro- Kuznetsov, Mirco Cantoro, Jeong Hun Mun, Byung-Jin man Kolesov, Rolf Reuter, Friedemann Reinhard, and Cho, Jun-ichi Hotta, Johan Hofkens, Marleen van der Jo¨rg Wrachtrup, “Single defect center scanning near-field Veen, Andr L. Stesmans, Marc M. Heyns, and Stefan opticalmicroscopyongraphene,”NanoLetters13,3152– De Gendt, “Charge transfer effects in graphene-cdse/zns 3156 (2013). quantum dots composites,” Proc. SPIE 8462, 84620L [33] Ying Wang, Zhaohui Li, Jun Wang, Jinghong Li, and (2012). Yuehe Lin, “Graphene and graphene oxide: biofunction- [19] Alexander V. Klekachev, Sergey N. Kuznetsov, Inge alization and applications in biotechnology,” Trends in Asselberghs, Mirco Cantoro, Jeong Hun Mun, Byung Biotechnology 29, 205 – 212 (2011). Jin Cho, Andr´e L. Stesmans, Marc M. Heyns, and Ste- [34] G Mazzamuto, A Tabani, S Pazzagli, S Rizvi, fan De Gendt, “Graphene as anode electrode for col- A Reserbat-Plantey, K Scha¨dler, G Navickaite, L Gau- loidalquantumdotsbasedlightemittingdiodes,”Applied dreau, F S Cataliotti, F Koppens, and C Toninelli, Physics Letters 103, 043124 (2013). “Single-moleculestudyforagraphene-basednano-position [20] ThFo¨rster,“Zwischenmolekulareenergiewanderungund sensor,” New Journal of Physics 16, 113007 (2014). fluoreszenz,” Annalen der physik 437, 55 (1948). [35] Shira Halivni, Amit Sitt, Ido Hadar, and Uri Banin, [21] KirillA.VelizhaninandAnatolyEfimov,“Probingplas- “Effectofnanoparticledimensionalityonfluorescenceres- mons in graphene by resonance energy transfer,” Phys. onanceenergytransferinnanoparticle-dyeconjugatedsys- Rev. B 84, 085401 (2011). tems,” ACS Nano 6, 2758–2765 (2012). [22] G. Gmez-Santos and T. Stauber, “Fluorescence quench- [36] JanJunisRindermann,GaliaPozina,BoMonemar,Lars ing in graphene: A fundamental ruler and evidence for Hultman, Hiroshi Amano, and Pavlos G. Lagoudakis, transverse plasmons,” Phys. Rev. B 84, 165438 (2011). “Dependence of resonance energy transfer on exciton [23] K.J.Tielrooij,L.Orona,A.Ferrier,M.Badioli,G.Nav- dimensionality,” Physical Review Letters 107, 236805 ickaite, S. Coop, S. Nanot, B. Kalinic, T. Cesca, L. Gau- (2011). dreau, Q. Ma, A. Centeno, A. Pesquera, A. Zurutuza, [6] Florian Godel, Emmanuelle Pichonat, Dominique Vig- H. de Riedmatten, P. Goldner, F. J. Garcia de Abajo, naud, Hicham Majjad, Dominik Metten, Yves Henry, 9 Stphane Berciaud, Jean-Francois Dayen, and David Hal- ton emission from CdSe/CdS nanocrystals measured us- ley,“EpitaxyofMgOmagnetictunnelbarriersonepitaxial ingsingle-particlespectroscopy,”PhysicalReviewLetters graphene,” Nanotechnology 24, 475708 (2013). 106, 187401 (2011). [38] Hans Kuhn, “Classical aspects of energy transfer in [52] Chunxing She, Igor Fedin, Dmitriy S. Dolzhnikov, Ar- molecularsystems,”TheJournalofChemicalPhysics53, naud Demortire, Richard D. Schaller, Matthew Pelton, 101–108 (1970). and Dmitri V. Talapin, “Low-threshold stimulated emis- [39] R.R.Chance,A.Prock, andR.Silbey,“Molecularfluo- sion using colloidal quantum wells,” Nano Letters 14, rescenceandenergytransfernearinterfaces,”Adv.Chem. 2772–2777 (2014). Phys 37, 65 (1978). [53] F Cichos, C Vonborczyskowski, and M Orrit, “Power- [40] R.S.SwathiandK.L.Sebastian,“Longrangeresonance law intermittency of single emitters,” Current Opinion in energytransferfromadyemoleculetographenehas(dis- Colloid & Interface Science 12, 272–284 (2007). tance)4 dependence,” The Journal of Chemical Physics [54] P.Spinicelli,S.Buil,X.Qu´elin,B.Mahler,B.Dubertret, 130, 086101 (2009). and J.-P. Hermier, “Bright and grey states in cdse-cds [41] D. M. Basko, V. M. Agranovich, F. Bassani, and nanocrystalsexhibitingstronglyreducedblinking,”Phys- G. C. La Rocca, “Energy transfer from a semiconductor ical Review Letters 102, 136801 (2009). quantum dot to an organic matrix,” Eur. Phys. J. B 13, [55] Anton V. Malko, Young-Shin Park, Siddharth Sampat, 653–659 (2000). Christophe Galland, Javier Vela, Yongfen Chen, Jen- [42] S. Kos, M. Achermann, V. I. Klimov, and D. L. Smith, niferA.Hollingsworth,VictorI.Klimov, andHanHtoon, “Differentregimesoffo¨rster-typeenergytransferbetween “Pump-intensity-andshell-thickness-dependentevolution an epitaxial quantum well and a proximal monolayer of of photoluminescence blinking in individual Core/Shell semiconductor nanocrystals,” Phys. Rev. B 71, 205309 CdSe/CdS nanocrystals,” Nano Letters 11, 5213–5218 (2005). (2011). [3] Mona B. Mohamed, Dino Tonti, Awos Al-Salman, Ab- [56] Micka¨elD.Tessier,Cl´ementineJavaux,IvanMaksimovic, delkrimChemseddine, andMajedChergui,“Synthesisof Vincent Loriette, and Benoit Dubertret, “Spectroscopy high quality zinc blende cdse nanocrystals,” The Journal of single CdSe nanoplatelets,” ACS Nano 6, 6751–6758 of Physical Chemistry B 109, 10533–10537 (2005). (2012). [2] Benoit Mahler, Piernicola Spinicelli, St´ephanie Buil, [57] R. S. Swathi and K. L. Sebastian, “Resonance energy Xavier Quelin, Jean-Pierre Hermier, and Benoit Du- transfer from a dye molecule to graphene,” The Journal bertret, “Towards non-blinking colloidal quantumdots,” of Chemical Physics 129, 054703 (2008). Nature Materials 7, 659–664 (2008). [58] ErminMalic,HeikoAppel,OliverT.Hofmann, andAn- [45] Benoit Mahler, Brice Nadal, Cecile Bouet, Gilles Patri- gel Rubio, “Fo¨rster-induced energy transfer in function- arche, and Benoit Dubertret, “Core/Shell colloidal semi- alized graphene,” The Journal of Physical Chemistry C conductornanoplatelets,”JournaloftheAmericanChem- 118, 9283–9289 (2014). ical Society 134, 18591–18598 (2012). [59] MarcoCalifano,AlbertoFranceschetti, andAlexZunger, [46] M. D. Tessier, B. Mahler, B. Nadal, H. Heuclin, “Temperature dependence of excitonic radiative decay in S. Pedetti, and B. Dubertret, “Spectroscopy of colloidal CdSequantumdots: Theroleofsurfaceholetraps,”Nano semiconductor Core/Shell nanoplatelets with high quan- Letters 5, 2360–2364 (2005). tum yield,” Nano Letters 13, 3321–3328 (2013). [60] R. Blachnik, J. Chu, R.R. Galazka, J. Geurts, [47] Margaret A. Hines and Philippe Guyot-Sionnest, “Syn- J. Gutowski, B. Ho¨nerlage, D. Hofmann, J. Kos- thesis and characterization of strongly luminescing zns- sut, R. L´evy, P. Michler, U. Neukirch, T. Story, cappedcdsenanocrystals,”TheJournalofPhysicalChem- D. Strauch, and A. Waag, “Semiconductors: Ii- istry 100, 468–471 (1996). vi and i-vii compounds; semimagnetic compounds,” [5] XuesongLi,WeiweiCai,JinhoAn,SeyoungKim,Junghyo in Landolt-Bo¨rnstein, New Series III/41B, edited by Nah,DongxingYang,RichardPiner,ArunaVelamakanni, U. Ro¨ssler (Springer Verlag, 1999). Inhwa Jung, Emanuel Tutuc, Sanjay K. Banerjee, Luigi [61] Jens Christian Johannsen, Søren Ulstrup, Federico Colombo, and Rodney S. Ruoff, “Large-area synthesis of Cilento, Alberto Crepaldi, Michele Zacchigna, Cephise high-quality and uniform graphene films on copper foils,” Cacho, I. C. Edmond Turcu, Emma Springate, Felix Science 324, 1312–1314 (2009). Fromm, Christian Raidel, Thomas Seyller, Fulvio Parmi- [49] W. H. Wang, W. Han, K. Pi, K. M. McCreary, F. Miao, giani,MarcoGrioni, andPhilipHofmann,“Directviewof W. Bao, C. N. Lau, and R. K. Kawakami, “Growth of hot carrier dynamics in graphene,” Physical Review Let- atomically smooth mgo films on graphene by molecular ters 111, 027403 (2013). beamepitaxy,”AppliedPhysicsLetters93,183107(2008). [62] Isabella Gierz, Jesse C. Petersen, Matteo Mitrano, [50] C. A. Leatherdale, W.-K. Woo, F. V. Mikulec, and Cephise Cacho, I. C. Edmond Turcu, Emma Springate, M. G. Bawendi, “On the absorption cross section of AlexanderSto¨hr,AxelKo¨hler,UlrichStarke, andAndrea CdSe nanocrystal quantum dots,” The Journal of Phys- Cavalleri,“Snapshotsofnon-equilibriumdiraccarrierdis- ical Chemistry B 106, 7619–7622 (2002). tributions in graphene,” Nature Materials 12, 1119–1124 [51] Y.-S. Park, A. V. Malko, J. Vela, Y. Chen, Y. Ghosh, (2013). F. Garcia-Santamaria, J. A. Hollingsworth, V. I. Klimov, and H. Htoon, “Near-unity quantum yields of biexci- 10 Supporting Information SYNTHESIS OF CdSe/ CdS NANOCRYSTALS Chemicals: 1-Octadecene(ODE,90%,Aldrich),oleylamine(OLA,70%,Fluka),oleicacid(OA,90%,Aldrich), sodium myristate (99 %, Fluka), cadmium oxide (99.99 %, Aldrich), selenium powder 100 mesh (99.99 %, Aldrich), sulfur (99.998 %, Aldrich), trioctylphosphine (TOP, 90 %, Cytec) and tetradecylphosphonic acid (TDPA, 97 %, PCI synthesis) were used as received. Precursors preparation: Cadmium myristate was prepared according to ref. [1]. The solution of cadmium oleate 0.5 M in oleic acid was synthesized by heating 6.42 g of CdO in 100 mL of oleic acid at 160 ◦C under argon until it turned colorless. The solution was then degassed under vacuum at 100 ◦C for 1 hour. Sulfur stock solution in ODE (S-ODE 0.1 M) was prepared by heating 480 mg of sulfur in 150 mL of degassed ODE at 120 ◦C until complete dissolution. TOP-Se 1 M in TOP was prepared by dissolving 15.8 g of Se powder in 200 mL TOP under magnetic stirring overnight in a glove box. Synthesis of CdSe cores: CdSe nanocrystals (NCs) were prepared by a procedure slightly modified adapted by Mahler et al. [2] from Mohamed et al. [3]. A mixture of 2 mL of Cd(oleate) (0.5 M) and 3 mL of ODE was 2 degassed under vacuum at 70 ◦C during 30 min and heated under argon flow up to 240 ◦C. A mixture of 1.5 mL of TOP-Se 1 M, 1.5 mL of oleylamine and 1 g of TDPA was heated until complete dissolution then injected and the solution was annealed for 30 seconds at 190 ◦C, and then immediately cooled down to room temperature. The solutionwaswashedupwith40mLofethanol. Thesolutionwascentrifugedat5500RPMinordertoprecipitatethe TDPA. The nanocrystals were suspended in 20 mL of toluene, washed again in 20 mL of ethanol, and dispersed in 10 mL of hexane. The nanocrystals obtained with this protocol were around 2 nm diameter, and their approximate concentration was 80 µM. Synthesis of CdS shell on the CdSe cores: For the CdS shell growth on the CdSe cores, a mixture of 3.1 mL of solution of cores dispersed in hexane, 5 mL ODE and 50 mg Cd(myr)2 was degassed under vacuum, at 70 ◦C for 30 minutes, and then put under argon flow. The temperature set value was then increased to 300 ◦C, and when the temperature reached 100 ◦C, 1 mL of OLA was injected, followed by a mixture of 8 mL SODE (0.1 M), 1.6 mL Cd(OA) (0.5 M) and 1 mL OLA at an injection rate of first 2 mL/h for 2 mL, then 16 mL/h for the rest of 2 the seringe. Once the injection was finished, a mixture of 0.5 mL OLA and 0.5 mL Cd(OA) 0.1 M diluted in OA 2 was added, and the solution was annealed for 10 minutes at 300 ◦C. The solution was then cooled down to room temperature and the nanocrystals were washed with ethanol, centrifuged and redispersed in 10 mL hexane. Luminescence TEM image s) 1 unitu 10 nm ee nsity (arb. Absorpption Occurrenc25 e nt I 10 nm 0 0 22.00 22.55 33.00 33.55 66 88 1100 1122 1144 Photon energy (eV) NC diameter (nm) FigureS1. (left)EnsembleabsorptionandphotoluminescencespectraoftheCdSe/CdSnanocrystalsusedinourmeasurements. (center) Transmission electron microscope image of the CdSe/CdS nanocrystals. (right) Histogram of the NC diameters. NC characterization: TheensembleabsorptionspectrumwasmeasuredonaCary5EUV-visiblespectrometer. Theensemblephotoluminescence(PL)spectrumwasacquiredwithanEdinburghInstrumentsFCS900spectrometer. Transmission electron microscopy (TEM) images were taken using a TEM JEOL 2010 with field emission electron gun. The results for CdSe/CdS NCs are shown in Figure S1. The NCs show strong PL at 2.14 eV, with a full width at half maximum (FWHM) of 140 meV. From the analysis of the TEM images, we find an statistically averaged diameter of (9.5±1.5) nm.
