Atomic Clocks and Coherent Population Trapping: Experiments for Undergraduate Laboratories

14 Pages·2009·0.52 MB·English

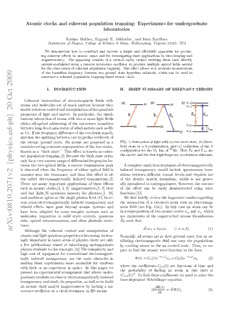
Atomic clocks and coherent population trapping: Experiments for undergraduate
laboratories
Nathan Belcher, Eugeniy E. Mikhailov, and Irina Novikova
Department of Physics, College of William & Mary, Williamsburg, Virginia 23185, USA
We demonstrate how to construct and operate a simple and affordable apparatus for produc-
ing coherent effects in atomic vapor and for investigating their applications in time-keeping and
magnetometery. The apparatus consists of a vertical cavity surface emitting diode laser directly
current-modulated using a tunable microwave oscillator to produce multiple optical fields needed
for the observation of coherent population trapping. This effect allows very accurate measurement
9 of the transition frequency between two ground state hyperfine sublevels, which can be used to
0
construct a coherent population trapping-based atomic clock.
0
2

t I. INTRODUCTION II. BRIEF SUMMARY OF RELEVANT THEORY
c
O
Coherent interactions of electromagnetic fields with
20 atoms and molecules are of much interest because they (a) (cid:231)aæ (b) (cid:231)aæ (c) FF''==21
enablecoherentcontrolandmanipulationofthequantum

] properties of light and matter. In particular, the simul- E E1 E2 E0 E+1
h taneousinteractionofatomswithtwoormorelightfields
p allows all-optical addressing of the microwave transition
- (cid:231)bæ (cid:231)bæ F=2
d betweenlong-livedspinstatesofalkalimetalssuchasRb D
e orCs. Ifthefrequencydifferenceofthetwofieldsexactly (cid:231)cæ F=1 hfs
. matches the splitting between two hyperfine sublevels of
s
c the atomic ground state, the atoms are prepared in a FIG.1: Interactionoflightwith(a)two-levelatom,(b)three-
si non-interactingcoherentsuperposition ofthe two states, level atom in a Λ-configuration, and (c) realization of the Λ
y known as a “dark state.” This effect is known as coher- configuration for the D1 line of 87Rb. Here E0 and E+1 are
h entpopulationtrapping.[2]Becausethe darkstate exists thecarrierandthefirsthigh-frequencymodulationsideband.
p
onlyforaverynarrowrangeofdifferentialfrequenciesbe-
[
tween the two optical fields, a narrow transmission peak Acompleteanalyticaltreatmentofelectromagnetically

2 is observed when the frequency of either optical field is induced transparency would include spontaneous tran-
v scanned near the resonance, and thus this effect is of- sitions between different atomic levels and requires use
1 ten called electromagnetically induced transparency.[3] of the density matrix formalism, which is not gener-
7
There are many important applications of these effects allyintroducedto undergraduates. However,the essence
0
such as atomic clocks,[4, 5, 6] magnetometers,[7, 8] slow of the effect can be easily demonstrated using wave
2
. and fast light,[9] quantum memory for photons,[1, 10] functions.[13]
0
andnonlinearoptics atthe singlephotonlevel.[11]Inre- We firstbriefly review the importantresults regarding
1
centyearselectromagneticallyinducedtransparencyand the interaction of a two-level atom with an electromag-
8
related effects have gone beyond atomic systems and netic field (see Fig. 1(a)). In this case an atom can be
0
: have been adapted for more complex systems such as inasuperpositionoftwoatomicstates ψa andψb,which
v
molecules, impurities in solid state crystals, quantum are eigenstates of the unperturbed atomic Hamiltonian
Xi dots, optical microresonators, and other photonic struc- Hˆ such that
0
tures.
ar Although the coherent control and manipulation of Hˆ0ψi =h¯ωiψi (i=a,b). (1)
atomicandlightquantumpropertiesisbecomingincreas- Normally, all atoms are in their groundstate, but an os-
ingly important in many areas of physics, there are only cillating electromagnetic field can vary the populations
a few publications aimed at introducing undergraduate by exciting atoms to the an excited state. Thus, we ex-
physicsstudentstotheconcepts.[12]Thecomplexityand pect to find the atomic wave function in the form
high cost of equipment for conventional electromagnet-
ically induced transparency are the main obstacles in Ψ(t)=Ca(t)e−iωatψa+Cb(t)e−iωbtψb, (2)
making these experiments more accessible for students
where the coefficients C (t) are functions of time and
with little or no experience in optics. In this paper we a,b
the probability of finding an atom in this state is
present an experimental arrangementthat allows under-
C (t)2. To find these coefficients we need to solve the
graduatestudentstoobserveelectromagneticallyinduced | a,b |
time-dependent Schr¨odinger equation
transparencyandstudyitsproperties,aswellastobuild
an atomic clock and/or magnetometer by locking a mi- ∂Ψ(t)
crowave oscillator on a clock resonance in Rb atoms. i¯h =HˆΨ(t), (3)
∂t
2
where Hˆ =Hˆ +Hˆ′. The interaction part of the Hamil- two nearly-resonant laser fields, forming the Λ configu-
0
tonian Hˆ′ contains information about the interaction of ration shown in Fig. 1(b). In this case the state of such
atoms with the electromagnetic field E(t) = cos(ωt). an atomic system is givenby a superposition of all three
E
When the light is nearly resonant, that is, when its (an- states:
gular)frequencyω isclosetothefrequencydifferencebe-
tween two atomic states ωab = ωa ωb, it is convenient Ψ(t)=Ca(t)e−iωatψa+Cb(t)e−iωbtψb+Cc(t)e−iωctψc.
−
to use the rotating wave approximation. This approxi- (7)
mation neglects the fast oscillating part of the solution In the following we assume that the lifetimes of the
(proportional to e±i(ω+ωab)t), which averages out at the groundstates ψb and ψc areverylong,andtheexcited
| i | i
detection stage,and keeps trackof only measurableslow state ψa spontaneously decays to the ground states at
| i
changes on a time scale proportional to 1/(ω ωab). In the average rate of γa. We also assume that each of
−
this case the only two non-zero matrix elements of the the electromagneticfields interactswith only one atomic
interaction Hamiltonian are[13] transition – the field E1(t) = 1cos(ω1t) couples the
E
states ψ and ψ , and the field E (t) = cos(ω t)
a b 2 2 2
H′ = ψ H′ ψ =h¯Ωe−iωt, (4a) couples|thie state|s ψi and ψ . ThisassumptEionmeans
ab h a| | bi | ai | ci
thatthe onlynon-zeromatrix elements ofthe three-level
and
systeminteractionHamiltonianareH′ =h¯Ω e−iω1tand
Hb′a =h¯Ωeiωt. (4b) H′ =h¯Ω e−iω2tandtheircomplexcoanbjugate1s. Ifwefol-
ac 2
lowthesamestepsasforatwo-levelsystem,wearriveat
Here Ω is proportional to the light field amplitude:
the following equations for the coefficients of the wave-
function in Eq. (7):
℘
ab
Ω= E, (5)
2h¯
iC˙ =Ω ei(ω1−ωab)tC +Ω ei(ω2−ωac)tC (8a)
a 1 b 2 c
where the parameter ℘ab ≡ hψa|−ez|ψbi is the matrix iC˙b =Ω1e−i(ω1−ωab)tCa (8b)
element of the electron dipole moment, whose value is
determined by the intrinsic properties of an atom and iC˙c =Ω2e−i(ω2−ωac)tCa. (8c)
characterizesthestrengthofinteractionwithanexternal
electromagnetic field. We have so far neglected the finite lifetime of the ex-
cited state ψ . To properly account for it we have
To find the equations describing the time evolution of a
| i
to use more sophisticated density matrix formalism.[1]
thestatecoefficientsC andC ,weneedtosubstitutethe
a b
However, the optical losses due to spontaneous emission
wavefunctioninEq.(2)intotheSchr¨odingerequation(3)
should be proportional to the population of the excited
and then use the orthogonality conditions for the wave
state C 2. Thus, if we can prevent atoms from get-
functions, ψ ψ =0, to find: a
a b | |
h | i ting excited, no energy will be dissipated, and the light
iC˙ =Ωei(ω−ωab)tC , (6a) field will propagate through atoms without any absorp-
a b
tion. Let’s examine Eq. (8a) more closely and find the
iC˙b =Ωe−i(ω−ωab)tCa. (6b) condition for which C˙ (t) = 0 at all times. This condi-
a
tioncorrespondsto casesforwhichno atoms fromeither
ThesolutionofEq.(6)iswell-known:[13]theatomicpop-
groundstateareexcitedatanytimeeveninthepresence
ulation cycles between the ground and excited states at
of the light fields, and there is no atomic population in
the frequency p(ω−ωab)2+Ω2. Such oscillations are the excited state (Ca(t) = 0), and therefore no light is
called Rabi flopping, and the parameter Ω is usually
absorbed. It is easy to see that this condition is possible
called the Rabi frequency.
only when:
Thus far we have considered only stimulated transi-
tions between two atomic states, which occur when the Ω C = Ω C ei[(ω2−ωac)−(ω1−ωab)]t. (9)
1 b 2 c
jumps between two atomic states are caused solely by −
an electromagnetic field. In this case atoms repeatedly Because the phases of laser fields are usually constant,
absorbandemit photons of the incident electromagnetic Eq. (9) requires that (ω ω ) (ω ω )=0, which
2 ac 1 ab
field, and no energy is lost in the process. This picture can be rewritten as − − −
is not completely accurate, because it does not take into
account the finite lifetime of the excited state. When ω ω =ω . (10)
2 1 bc
−
atoms are in the excited states, they can spontaneously
decay into the ground state by emitting a photon in a This condition is often called a two-photon resonance,
random direction, so that the energy carried out by this because it requires the difference of the frequencies of
photon is lost from the original light field. As a result, two light fields to match the frequency splitting of two
some fraction of resonant light is absorbed after the in- metastable states ω . For the exact two-photon reso-
bc
teraction with atoms. nance the condition (9) becomes much simpler: Ω C =
1 b
We now return to the main topic of interest for our Ω C . If we substitute the coefficients into the gen-
2 c
−
experiment – the interaction of three-level atoms with eral expression for the wave function (7) and choose the
3
proper normalization, we find that there exists a non- longerthanacharacteristicdarkstate lifetime τ. There-
interacting quantum state of the atomic system that is fore, even for small non-zero two-photon detuning, the
completely decoupled from the excited state: transparency remains if the additional phase accumu-
lated by the dark state during its lifetime is small, δ
Ω2e−iωbtψb Ω1e−iωctψc 1/τ. For larger two-photon detuning the dark state n≤o
D = − . (11)
| i pΩ21+Ω22 longerexists,andtheamountoflightabsorptionbecomes
large. Thuswecancharacterizethewidthofthecoherent
Anyatominstate D nevergetsexcitedtothe level a , populationtrapping resonance,thatis, the rangeoftwo-
and the sample vie|weid from the side stays dark due| tio photon detunings where transmission is still high. The
the lack of spontaneous emission. For that reason such exact expression of the width can be found only using a
a quantum state is often called a “dark state,” in which more complete treatment:[4]
the atomic population is “trapped” in two lower states.
1 Ω 2+ Ω 2
We can now understand what happens when an atom δ = + | 1| | 2| . (13)
CPT
τ γ
enters the interaction region. Before interacting with a
light the atomic population is equally distributed be- Forstrongerlaserfieldscoherentpopulationtrappingres-
tween two low energy states; that is, half of the atoms onance is broadened (“power broadened”), but the ulti-
areinthestate b andhalfareinthe state c . Itis very mate width is limited by the inverse lifetime of the dark
| i | i
importanttodistinguishthisstatisticalmixturefromthe state.
coherentsuperpositionoftwostates (b + c )/√2. Dur- We have discussed an idealized three-level atom, but
| i | i
ing the interaction with light the atoms are excited to no such atoms exist in nature. It is possible to real-
state a , and then spontaneously decay either into the ize a three-level Λ system in alkali metals very similar
| i
dark state, or into its orthogonalcounterpart,the bright to one we have considered. In these elements, two non-
state. The atoms in the dark state do not interact with degeneratehyperfine statesofthe groundnS levelare
1/2
the laser fields and remain in this state for as long as usedasstates ψ and ψ ,andtheelectronstatenP
b c 1/2
it exists. The atoms in the bright state interact with or nP becom| esithe e|xciited state ψ . Because spon-
3/2 a
the applied fields, so that they go through an excita- taneous radiative decay between two| hyiperfine states of
tion and spontaneous decay cycle many times before all the same ground level is strictly forbidden, an atom can
atoms end up in the dark state. After such a steady stayineitherstateuntilitinteractswithitsenvironment.
state is achieved, the atomic medium becomes transpar- It is now possible to preserve the quantum state of
ent,becausebothresonantlightfieldspropagatewithout atoms for up to several seconds, but it requires using
any optical losses. Note that the dark state is a coher- cold atoms or exotic chemical coatings. In a regular va-
ent superposition of the two atomic states b and c , por cell (a sealed glass cell filled with alkali metal vapor
| i | i
and is sensitive to their relative phase. That exchange at about room temperature) the main limitation of the
requires the two optical fields to maintain their relative ground state lifetime is the motion of atoms. Once an
phaseatalltimesinorderforatomstostay“invisible”to atomleavesthelaserbeam,itislikelytocollidewiththe
the light fields. That is why this effect is called coherent glasswallandthermalizeandloseanyinformationabout
population trapping. its previous quantum state. For a 1mm laser beam the
We now discuss what happens to light transmission if interactiontime of an atom is limited to a few µs, which
the frequency of one of the lasersis changing. If the sys- correspondsto a coherentpopulation trapping linewidth
tem is not exactly at the two-photon resonance, a small of tens or evenhundreds of kHz. Sometimes a buffer gas
two-photondetuningδ =ω2 ω1 ωbc =0causesaslow — usually a non-interacting inert gas — is added to the
− − 6
variation in the relative phase of the two ground states: vapor cell together with alkali metal. In this case alkali
atoms diffuse through the laser beam rather than mov-
Ω2e−iωbtψb eiδ·tΩ1e−iωctψc ing ballistically, thus increasing the interaction time by
D = − . (12)
| i pΩ21+Ω22 a few orders of magnitude, producing narrower coherent
population trapping resonances.
If the two-photon detuning parameter δ is small, we can
still use the dark state formalism as long as δ t<1. At
longer times such a “disturbed dark state” st·art∼s inter- III. EXPERIMENTAL SETUP
actingwiththelaserfields,causingnon-zeroatomicpop-
ulation in the excited state and associated optical losses Diode lasers are an ideal choice for working with al-
due to spontaneous emission. kali metals (K, Rb, Cs)[14] because they are affordable,
So far we have allowed atoms to remain in the dark reliable, and easy to operate, and cover the right spec-
state indefinitely. In a real system some decoherence tral range. The exact output frequency of a diode laser
mechanisms are present to disturb a quantum state by canbefine-tunedbychangingthedrivingcurrentand/or
randomizing its phase or forcing atoms to jump be- the temperature ofthe diode. In ourexperiments weuse
tween two random energy levels. Even under perfect a laser resonant with the D line of 87Rb (wavelength
1
two-photonresonanceatoms cannot be in the dark state λ = 795nm). A different Rb isotope or any other alkali
4
metal[15, 17, 18] can be used to reproduce the exper- very low power consumption. Both of these properties
iments described in the following with an appropriate make a VCSEL the laser of choice for miniature atomic
change in the operational parameters. clock applications.[4]
The level structure of the 87Rb D1 line is shown in In the following we give a detailed description of the
Fig. 1(c). Both the ground (5S ) and the excited experimentalapparatusinourlaboratory.[19]Anapprox-
1/2
(5P ) states are split due to the coupling of the elec- imate budget for the experiment is provided in Table I.
1/2
tron angular momenta and the nuclear spin, and each Laser assembly. Figure 2 shows the homemade
state is labeled with the value of the total angular mo- laser head assembly used in our experiment. A VC-
mentumF. Theobservationofcoherentpopulationtrap- SEL (ULM795-01-TN-S46FOP from U-L-M Photonics)
pingrequirestwolaserfieldstocouplebothgroundstates is placed inside a collimating tube (model LDM 3756
F = 1 and F = 2 with the same excited state (F′ = 2 from Optima Precision) and a copper holder. The re-
in our experiments). However, it is impossible to use sulting assembly is attached to a peltier thermoelec-
twoindependentdiodelasersduetotheirrelativelylarge tric cooler connected to a temperature controller (model
intrinsic frequency noise. On one hand, a dark state WTC3293-14001-Afrom Wavelength Electronics) to ac-
(12) exists only if the differential frequency of two laser tivelystabilizethediodetemperaturewithprecisionbet-
fields matches the ground state splitting with good pre- ter than 0.1◦C. The basis for the laser system is the
cision (typically better than a few kHz). On the other heat sink, which doubles as the holding block for the
hand, the electromagnetic field emitted by a laser is not system. The sensitivity of the laser frequency to tem-
truly monochromatic,but instead its frequency “jumps” perature (0.06nm/◦C for our laser) introduces a way to
randomly in a certain range, called the laser linewidth. tunethelasertotheatomicresonancefrequency,butalso
Typical linewidths of commonly used diode lasers are puts stringent requirements on the diode’s temperature
from 10–100MHz for a free-running diode laser to sev- stability. To prevent temperature fluctuations due to air
eral hundred MHz for a vertical cavity surface emitting currents,thelasermountisenclosedinasmallaluminum
diode laser (VCSEL). Even more sophisticated commer- box.
cial external-cavity diode lasers have linewidths of the
The laser diode pins are soldered to a standard SMA
order of 1MHz. As a result, the two-photon detuning
connectorandpluggedintoaBias-T(modelZFBT-6GW
of two independent lasers fluctuates in the range deter-
from Mini-Circuits), which combines a high-frequency
mined bythe laserlinewidths, andnonarrowresonances
modulation signal and a constant current, required to
can be observed.
drive the VCSEL. A typical driving current required to
To avoid this problem we obtain several electromag-
poweraVCSELisverysmall(themaximumallowedcur-
netic fields from a single laser by modulating its phase
rentis3mAinoursample). Toextendthelifetimeofthe
ϕ(t) = ǫsin(ω t). Such phase modulation is equivalent
m diode,weoperateditat1–1.5mA,resultinginanoutput
to producing a frequency comb with the frequency sepa-
optical laser power of 0.5mW, collimated to a 1mm
ration between the “teeth” equal to the modulation fre- ≈
beam. Any variations in laser current also cause fluc-
quency ω ,andthe amplitude ofeachcomponentdeter-
m tuations in the output laser frequency ( 0.9nm/mA).
mined by the phase modulation amplitude ǫ:[16] ≈
We have designed a simple, battery-operated, low-noise
current supply optimized to drive a VCSEL (Figure 3
1
E(z,t)= eikz−iωt+iϕ(t) +c.c. (14a) provides the schematics.) For the coherent population
2E
trapping and clock measurements, the output laser fre-
∞
= 21EXJn(ǫ)eikx−i(ω−nωm)t+c.c. (14b) quusienngcya DisAaVlsLoLa(cDtiivcehlryoilcocAkteodmtioc VthaepoartoLmaiscertrLaoncskitiniogn)
n=0
technique.[20, 21]
In our experiments we use phase modulation frequency Microwave modulation. Inadditiontoaconstantdriv-
ω to be close to the hyperfine splitting frequency in ing current, a high-frequency signal has to be mixed in
m
87Rb ∆ = 6.835GHz. We then use two of the result- to phase-modulate the output of the laser and produce
hfs
ing frequencycombcomponents toformaΛ system: the the frequency comb given by Eq. (14). In our experi-
zeroth(atthecarrierfrequencyω)andoneofthefirst(at mentswetestedseveralmicrowaveoscillators. Ourinitial
frequency ω ω ) modulation sidebands. It is also pos- tests used a commercial frequency synthesizer (Agilent
m
±
sible to phase modulate the laser field at half of the hy- E8257D) available in our laboratory. For all later work
perfine splitting frequency, and use two first modulation it was replaced with less expensive options: a current-
sidebands to achieve coherent population trapping.[4] A tunable crystal oscillator and an electronic phase-locked
special type of diode laser (a VCSEL)[15] allows effi- loop (PLL) with external 10MHz reference.
cient phase modulation at the desired high microwave The most affordable rf source was a Stellex Mini-YIG
frequencybydirectlymodulatingitsdrivingcurrent. For oscillatorpurchasedfromasurpluselectronicseller. This
this type of laser an active region (where the lasing oc- oscillator outputs 15dBm of power at a frequency be-
curs)issmallerthaninconventionaledge-emittingdiode tween 5.95GHz and 7.15GHz. The output frequency
lasers,providingtwomainadvantages: fastresponse(up of the oscillator can be tuned by changing the current
to10GHz modulationwasachievedforoursample), and flowingthroughtwo internalmagnetic coils: a main coil,
5
TABLEI:Welist thesourcesfortheimportant componentsoftheproposedexperimentsandcurrentprices. Thelist doesnot
include raw materials (such as aluminum or acrylic sheets or prototypingcircuit boards).
Component Source Price Quantity
Laser assembly
VCSEL laser @795nm ULM795-01-TN-S46FOP, Laser components $400 1
for different wavelengths VCSEL-780 or -850 Thorlabs $20
Collimating tube LDM-3756, OptimaPrecision $75 1
Bias-T ZX85-12G+, Mini-Circuits $100 1
Temperature controller WTC3293, Wavelength Electronics $500 1
TEC element 03111-5L31-03CP, Custom Thermoelectric $20 1
Optical isolator (optional) IO-D-780-VLP,Thorlabs $460 1
Rubidium cell enclosure
Rbcell: isotopically
∗
enriched/natural abundance Triad Technology $625/$310 1
′
Bifiler heating wire 2HN063B-13 AriIndustries $4/ft 10
Magnetic shielding
Custom 3 layer design Magnetic Shield Corporation $1300 1
alternative: lab kit $100
Microwave equipment
TunableMini-YIG Oscillator Stellex, purchased used on eBay $50 1
Linear PLL chip evaluation board LMX2487EVAL National Semiconductor $176 1
Voltage controlled oscillator CRO6835ZZ Communications $75 1
10 MHz reference oscillator 501-04609A Streamline $305 1
Outputrf amplifier Amplifier ZJL-7G Mini-Circuits $100 1
Various attenuators VAT-x Mini-Circuits VAT-x $16/each 3–4
Directional coupler 780-20-6.000 MECA Electronics $165 1
Various optics and opto-mechanics
Mirrors Thorlabs (various options) $15–$50 2–3
∗
Quarterwave-plate WPMQ05M-780 Thorlabs $230 1
∗
Photodetector Thorlabs (various options) $20–$100 1
Optical mounts& holders Thorlabs ≈$300
General lab equipment
Oscilloscope, function generator, lock-in amplifier, frequency counter, constant ±15V and ±5V and variable
power supplies, multimeters.
∗ Additional items are needed if DAVLL laser lock is used.
designed for coarse tuning of the oscillator frequency coil is responsible for fine frequency adjustments in the
(5MHz/mA) at a slow rate up to 10kHz, and an aux- narrow range of about 1MHz around the set value. Cir-
iliary fast tuning coil that allows finer frequency control cuits for the oscillator current driver and for the active
(150kHz/mA) with the 400kHz tuning bandwidth. As locking servo are shown in Figs. 4 and 5. The tuning
for the VCSEL driver, a current source used for tuning source allows tuning the oscillator frequency to a par-
musthaveminimalinternalnoise(betterthanmanycom- ticular microwavefrequency or sweeping the modulation
mercial laser current drivers), because any modulation frequency to observe changes in the optical transmission
frequency fluctuations are immediately converted into as a function of the two-photon detuning. Although all
fluctuations of the two-photon detuning. In our case the the experiments we discuss can be performed using the
tuning current required to reach the designed oscillator Stellex oscillator, it is sometimes not very convenient to
frequency (6.835GHz) is greater than 100mA. To sim- use because of its large internal frequency jitter at the
plifytheconstructionofatunablelow-noisehigh-current level of several tens of kilohertz. Also, without active
source, we used a two-stage design. The oscillator fre- frequency locking, its frequency drifts several hundreds
quency is coarsely set to the required value by manually ofkilohertzduringanhour,probablybecause ofits poor
adjusting a cw current source driving the main tuning temperature stability.
coilto approximately140mA, andanadditionaltunable
The other microwave source we used requires more
low-currentsource pluggedinto the auxiliaryfast tuning
initial investments, but provides much greater preci-
6
FIG. 2: (a) A VCSEL attached to an SMA connector. (b) The schematics of thelaser head assembly.
FIG. 3: Constant current source for a VCSEL laser. Maximum available current is 2mA.
7
sion and stability in the output frequency. It is cell, and P is the atmospheric pressure. For exam-
atm
based on the Linear PLL chip (LMX2487) evalua- ple, for the 1mm beam used in the experiment, 5Torr
tion board (LMX2487EVAL), which sets a user pro- of Ne buffer gas extends the interaction time from 2.5µs
vided voltage controlled oscillator at an arbitrary fre- to 60µs. The exact total amount and composition of
quency near 6.835GHz with sub-Hertz resolution (we a buffer gas is not critical. Any cell with 5–50Torr of
used CRO6835Z). The output signal is phased-locked to any inert gas (Ne, He, Ar, Xe) or some simple diatomic
a 10MHz reference. This feature can be used, for exam- molecules (N , CH ) is suitable for the experiments de-
2 4
ple, to lock the rf output to a stable frequency reference scribed in the next sections.
(such as a benchtop Rb frequency standard FS725 from Any longitudinal magnetic field splits the magnetic
Stanford Research Systems). Also, we can slightly vary sublevels of all hyperfine states due to the Zeeman ef-
the frequency of a voltage-controlled 10MHz oscillator fect, and thereby changes their two-photon resonance
toachievesweepingcapabilityintherfoutputfrequency. frequencies. To avoid stray fields from the laboratory
WeusedaStreamlineoscillator(501-04609A)withavolt- environment, the Rubidium cell is placed inside a set of
age tuning response of 5Hz, corresponding to a varia- three cylindricalmagnetic shields (from Magnetic Shield
±
tion in the microwave frequency of 3kHz. This tuning Corporation) to suppress any external magnetic field by
±
is sufficient to lock the rf output to the coherent pop- a factor of 103–104. Much simpler single-layer shielding
ulation trapping resonance and study its stability in an maybesufficienttoobservecoherentpopulationtrapping
atomic clock arrangement. resonances of a few tens of kHz wide. It might also be
The microwaveoutput ofeither oscillatoris connected useful to install a solenoid inside the shielding to change
to the laser through the bias-T. The power of the oscil- the longitudinal magnetic field and study its effect on
lator determines the strength of the modulation. Fig- coherent population trapping.
ure 6(a) shows the modulation comb for two values of The number of Rb atoms interacting with light (that
rf power recorded by passing the laser output through a is, the Rb vapor density inside the cell) is determined
handmade Fabry-Perotcavitywith 40GHz free spectral by the saturated vapor pressure with the solid or liquid
range. Figure 6(b) shows the ratio between the first and Rb metal droplet placed inside the cell, and can be con-
the zeroth (carrier) modulation sidebands, which grows trolledby changingthe cell’s temperature.[14] In our ex-
proportionally to the microwave power sent to the VC- periment we control the temperature of the cell by pass-
SEL. Due to the high efficiency of the high-frequency ing constant electrical current through a resistive heater
currentmodulation,wewereabletotransferalargefrac- wrappedaroundtheinnermagneticshield. Toavoidany
tionoftheopticalpowerinthefirstmodulationsideband, magneticfieldduetotheheatercurrent,weusedabifiler
achieving the first sideband/carrier ratio >1 for moder- chromium wire as a heating element. Alternatively, any
ate modulation power 15mW (12dBm). twistedloopofwirecanbeused,becausethecurrentwill
≥
Rubidium cell enclosure. The remaining important run in opposite directions along each point of the wire.
componentofthe experimentalsetupis aRubidium cell. We found that the coherent population trapping reso-
In our experiment we used a standard cylindrical glass nances have the highest contrast at some optimal range
cell (25mm diameter, 75mm length) containing isotopi- of temperatures (35–50◦C for our experiment). If the
callypure87Rb(TT-RB87/Ne-75-QfromTriadTechnol- temperature is too low, almost all the light gets through
ogy). Itiseasytoestimatethatatroomtemperature,Rb regardlessof coherentpopulation trapping conditions. If
atomsinsidethecellmovewithaveragespeedof400m/s, the temperature is too high, itis difficult to see coherent
and thus it takes only 2µs to cross the 1mm-wide laser populationtrappingresonancesduetostrongabsorption.
beam.[22] After that time an atom most likely collides
with the cell wall, and its quantum state created dur-
ing the interaction with the laser fields is destroyed. To
IV. OBSERVATION AND
amend the situation, our cell also contains 5Torr of Ne
CHARACTERIZATION OF COHERENT
buffer gas. Collisions with buffer gas atoms have a very
POPULATION TRAPPING RESONANCES
weak effect on the atomic quantum state, but rapidly
changethevelocityofRbatoms,restrictingtheirmotion
Our experimental apparatus enables a range of exper-
to slow diffusion. As a result, Rb atoms spend a much
iments onthe coherentpropertiesofatoms andtheir ap-
longer time inside the interaction region, effectively in-
plications. The first and basic one is the observation of
creasing their dark state lifetime. For example, for Rb
coherent population trapping resonances by measuring
atoms in Ne buffer gas, the diffusion time of an atom
the transmission of the laser light through the atomic
through the laser beam diameter a can be calculated
cell while scanning the laser modulation frequency over
by solving the diffusion equation. The solution is given
the two-photon resonance. The first step in this process
by[23]
is tuning the laser to the right optical frequency. Fig-
a2 P ure 8 shows the transmission of the laser light when its
Ne
τdiff = 1.15[cm2/s]P , (15) frequency is swept across all four optical transitions of
atm the 87Rb D line [the transitions are shown in Fig. 1(c)].
1
where P is the pressure of the Ne buffer gas inside the The spectralwidth of eachabsorptionline is determined
Ne
8
FIG. 4: Constant current source for a microwave oscillator tuningcoil. Maximum available current is 150mA.
9
by the decoherence rate of the optical transitions of ap- broadening of coherent population trapping resonances
proximately600MHzFWHM(dominatedbytheDoppler at higher laser powers. It is easy to verify this broad-
broadening). As a result, the two transitions from the ening experimentally by measuring the resonance width
same ground state to each of the excited states are not whilereducingthelightpowerinteractingwithatomsby
completely resolved, because their relatively small hy- placing(forexample)afewneutraldensityfiltersinfront
perfinesplitting( 800MHz)iscomparabletothewidth of the cell. The example of the resonance narrowing is
≈
of each individual transition. The transitions from each shown in Fig. 9. Note that the resonance linewidth may
of two ground states are clearly separated due to signif- notfollowEq.(13)exactly,especiallyformoderatebuffer
icantly larger ground-state hyperfine splitting (∆ = gaspressure( 10Torr),becausethedynamicsofatomic
hfs
≤
6.835GHz). diffusion becomes important. In particular, if atoms are
Theoutputopticalfrequencyofthelaserissensitiveto allowed to diffuse out of the laser beam and then re-
the rf modulation power, and the precise tuning should turnwithoutlosingtheirquantumstate,[24]thewidthof
be performed with modulation turned on. The tuning thecoherentpopulationtrappingresonancesmaybecome
processismoreconvenientiftheopticalpowerineachof much narrower than expected from the simple diffusion
thefirstmodulationsidebandsis 20–60%ofthecarrier picture provided by Eq. (15).
field, so it is easy to distinguish t≈he carrierand sideband Wecanalsoobservethatthecoherentpopulationtrap-
absorption. Then the rf oscillator frequency should be ping resonance lineshape becomes asymmetric when the
appropriately tuned – first with the coarse tuning to a optical laser frequency is detuned from the exact opti-
value close to the hyperfine splitting, and then with fine cal transition frequency.[25] This effect can be used to
adjustments such that a clear increase in light transmis- fine tune the laser frequency to the resonance position,
sion is observed near Rb resonances, as illustrated by which corresponds to the most symmetric coherent pop-
the difference in the solid lines in Fig. 8. To achieve the ulation trapping resonance. At the same time, students
maximum contrast of coherent population trapping res- may find it interesting to observe this systematic line-
onances with circularly polarized light, the carrier (that shape change,accompaniedby the reductionofthe reso-
is, the unperturbed laser frequency) should be tuned to nanceamplitudeandgrowthinbackgroundtransmission
the F = 2 F′ = 2 transition. In this case, the as fewer and fewer atoms interact with the laser fields.
→
high-frequencymodulationsidebandisresonantwiththe
F = 1 F′ = 2. This arrangement is indicated by an
→ V. EFFECT OF THE ZEEMAN STRUCTURE
arrow in Fig. 8.
ON COHERENT POPULATION TRAPPING
Once the laser opticalfrequency is parkedat the right
RESONANCES; ATOMIC MAGNETOMETER
transition, we can directly observe a coherent popula-
tion trapping resonance by slowly scanning the modula-
So far we have ignored the fact that each hyperfine
tion frequency near the two-photon transition. Figure 9
sublevel F consists of 2F +1 magnetic (Zeeman) sub-
showsexamplesofnarrow(afewkHz)peaksinthetrans-
levels characterized by m . These sublevels are degen-
mitted light power after the Rb cell when the coherent F
erate in zero magnetic field, but if a magnetic field is
population trapping condition is fulfilled. Because all
applied along the light propagation direction (for exam-
the laser light is detected, the coherent population trap-
ple,byrunningthecurrentthroughthesolenoidmounted
ping transmission peak is observed on top of an often
inside the magnetic shielding), the sublevels shift by an
large background. This background transmission con-
amount proportional to the applied magnetic field B:
sists of the off-resonant modulation sidebands, which do
not interact with atoms, as well as residual transmission
δ =m g B, (16)
of the resonant light fields, if the atomic density is not mF F F
too high. It is also easy to see that even the maximum wherem isthemagneticquantumnumberforeachsub-
F
of the coherent population trapping resonance does not level,andg isthegyromagneticratio. ForRblevelsthe
F
reach100%transmission,becausethelifetimeofthedark gyromagnetic ratios are very small (g = 0.7MHz/G
F=1
state is finite, and there is always a fraction of atoms in and g = 0.7MHz/G),[14] and a highmagnetic field
F=2
the laser beam that is absorbing light. The exact po- is required to−shift the levels far enough to resolve indi-
sition of the transmission maximum depends on many vidual optical absorption resonances from different Zee-
parameters,suchasthepowerofthelight(“lightshift”), mansublevels. Incontrast,coherentpopulationtrapping
and the amount and the temperature of the buffer gas resonances are much more sensitive to level shifts.
(“pressure shift”). For example, in the cell we used in Let’s look more carefully at what happens to a coher-
the experiments (filled with 5Torr of Ne) the position of ent population trapping resonance in the presence of a
thetwo-photonresonanceatlowlightlevelwasmeasured magnetic field. Figure 10(a) shows the relevant Rb en-
to be 6.834685GHz. ergy levels taking into account their magnetic structure.
After initial detection of coherent population trap- In this case we must consider not one, but three inde-
pingresonances,itmaybeusefultosystematicallystudy pendent Λ systems formed by the carrier and high fre-
important properties such as the dependence on the quency sideband fields between three pairs of magnetic
laser field strength. For example, Eq. (13) predicts the sublevels m =0, 1 in the ground states and magnetic
F
±
10
FIG. 5: A proportionalintegral (PI) servo controller for the oscillator frequency lock.