Table Of Content

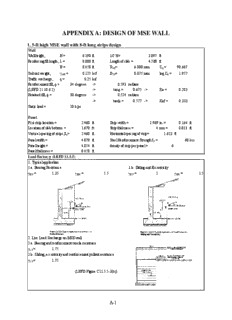
APPENDIX A: DESIGN OF MSE WALL 1. 5-ft high MSE wall with 8-ft long strips design Wall Wall height, H = 6.190 ft 1/2 H= 3.095 ft Reinforcing fill length, L = 8.000 ft Length of slab = 4.500 ft B = 8.458 ft D = 6.800 mm C = 90.667 60 u Soil unit weight,  = 0.125 kcf D = 0.075 mm log C = 1.957 soil 10 u Traffic surcharge, q = 0.25 ksf Reinforcement fill,  = 34 degrees -> 0.593 radians (LRFD 11.10.6.2) -> tan = 0.675 -> Ka = 0.283 Retained fill,  = 30 degrees -> 0.524 radians -> tan = 0.577 -> Kaf = 0.333 f Static load = 10 kips Panel First strip location = 2.460 ft Strip width = 1.969 in. = 0.164 ft Location of slab bottom = 1.670 ft Strip thickness = 4 mm = 0.013 ft Vertical spacing of strips,S = 2.460 ft Horizontal spacing of strip= 1.623 ft v Panel width = 4.870 ft Steel Reinforcement Strength f = 60 ksi y Panel height = 4.854 ft density of strip per panel = 6 Panel thickness = 0.458 ft Load Factor, (LRFD 11.5.5) 1. Typical application 1.a. Bearing Resistance 1.b. Sliding and Eccentricity  = 1.35  = 1.5  = 1  = 1.5 EV EH EV EH 2. Live Load Surcharge on MSE wall 2.a. Bearing and reinforcement tensile resistnace  = 1.75 LS 2.b. Sliding, eccentricity and reinforcement pullout resistance  = 1.75 LS (LRFD Figure C11.5.5-3(b)) A-1 Resistance Factor, (LRFD Table 11.5.6-1) Mechanically Stabilized Earth Walls Pullout resistance of tensile reinforcement, Static loading = 0.9 Combined static and impact loading = 1 Tensile Resistance of strip reinforcement, Static loading = 0.75 Combined static and impact loading = 1 1. External Stability 1.1 Static Mass Stability (LRFD Figure 11.10.5.2-1) 1.1.1 Vertical loads 1. Reinforced Soil V =  × H × L soil V1= 0.125 (kcf) × 6.19 (ft) × 8 (ft) = 6.190 kips/ft  × V1= 1.35 × V1= 8.357 kips/ft EV Moment arm of V1 = 4 ft M = 6.19 (kips/ft) × 4 (ft) = 24.760 ft-kips/ft v1  × M = 1.35 × M = 33.426 ft-kips/ft EV v1 v1 2. Traffic surcharge V2= 0.25 (ksf) × 8 (ft) = 2.000 kips/ft  × V2= 1.75 × V2= 3.500 kips/ft LS Moment arm of V2 = 4 ft M = 2 (kips/ft) × 4 (ft) = 8.000 ft-kips/ft v2  × M = 1.750 × M = 14.000 ft-kips/ft LS v2 v2 ∑V = 8.19 kips/ft ∑Mv = 32.760 ft-kips/ft ∑V = 11.86 kips/ft ∑Mv = 47.426 ft-kips/ft A-2 1.1.2 Horizontal loads 1. Retained soil F1= 1/2 × soil × H2 × Kaf F1= 1/2 × 0.125 (kcf) × 38.316 (ft2) × 0.333 = 0.798 kips/ft  × F1= 1.5 × F1= 1.197 kips/ft EH Moment arm of F1 = 6.19 /3 = 2.06 ft M = 0.798 (kips/ft) × 2.063 (ft) = 1.647 ft-kips/ft F1  × M = 1.5 × M = 2.471 ft-kips/ft EH F1 F1 2. Traffic surcharge F2= q × H × Kaf F2= 0.250 (ksf) × 6.190 (ft) × 0.333 = 0.516 kips/ft  × F2= 1.5 × F2= 0.774 kips/ft LS Moment arm of V2 = 3.095 ft M = 0.51583 (kips/ft) × 3.095 (ft) = 1.597 ft-kips/ft F2  × M = 1.5 × M = 2.395 ft-kips/ft LS F2 F2 ∑F = 1.31 kips/ft ∑MF = 3.244 ft-kips/ft ∑F = 1.97 kips/ft ∑MF = 4.865 ft-kips/ft 1.1.3 Sliding (LRFD 11.10.5.3) Sliding without Load Factor= ∑V*tan = 8.190 ×tan 30 = 3.598 ∑FH = 1.314 Sliding with Load Factor = ∑EVV*tan= 11.857 ×tan 30 = 3.473 ∑EHFH = 1.971 1.1.4 Overturning (LRFD 11.10.5.3) Overturning w/o Load Factor= ∑Mv = 32.760 = 10.100 ∑MF 3.244 Overturning w/ Load Factor= ∑EVMv = 47.426 = 9.748 ∑EHMF 4.865 1.2 Bearing Capacity at Base Eccentricity w/o Load Factor= L - ∑M - ∑M v F 2 ∑V = 8 - 32.760 - 3.244 = 0.396 2 8.190 Eccentricity w/ Load Factor = L - ∑EVMv - ∑EHMF 2 ∑EVV = 8 - 47.426 - 4.865 = 0.410 2 11.857 ≤ B = 1.410 ft OK 6 A-3  w/o Load Facto r= ∑V = 8.19 = 1.136 ksf v (L-2e) 8 - 2 × 0.39604 v w/ Load Facto r= ∑EVV = 11.86 = 1.651 ksf (L-2e) 8 - 2 × 0.41035 2. Internal Stability 2.1 Static Load 2.1.1 Compute Kr (LRFD Figure 11.10.6.2.1-3)  Kr = 1.7 × Ka = 1.7 × 0.28 = 0.48 at 0 ft EH  Kr = 1.2 × Ka = 1.2 × 0.28 = 0.34 under 20 ft EH Use interpolation at other depth 2.1.2 Fisrt strip at h1= 2.46 ft h1 = 2.46 ft kr = 0.463 1. Vertical stress 1) Reinforced Soil  =  × H V1 soil V1 = 0.125 (kcf) × 2.460 (ft) = 0.308 kips/ft2 EV × V1 = 1.35 ×  = 0.415 kips/ft2 2) Traffic surcharge  = 0.25 ksf V2 EV × V2 = 1.75 × 0.25 = 0.438 kips/ft2 a) ignoring tracffic surcharge b) including tracffic surcharge ∑v = 0.308 kips/ft2 ∑v = 0.558 kips/ft2 ∑EVv = 0.415 kips/ft2 ∑EVv = 0.853 kips/ft2 Horizontal stress,  =  ( k +  ) (LRFD Eq. 11.10.6.2.1-1) H P v r H a) ignoring tracffic surcharge  =  k = 0.308 ksf × 0.463 = 0.142 ksf h v r   =   k = 0.415 ksf × 0.463 = 0.192 ksf EV h EV v r At per strip = 4.870 (ft) × 2.460 (ft) / 3 = 3.993 ft2 Tmax = H Sv = 0.142 ksf × 3.993 ft2 = 0.57 kips per strip EV Tmax = EV H Sv = 0.192 ksf × 3.993 ft2 = 0.77 kips per strip A-4 b) including tracffic surcharge  =  k = 0.558 ksf × 0.463 = 0.258 ksf h v r   =   k = 0.853 ksf × 0.463 = 0.395 ksf EV h EV v r Tmax = H Sv = 0.258 ksf × 3.993 ft2 = 1.03 kips per strip EV Tmax = EV H Sv = 0.395 ksf × 3.993 ft2 = 1.58 kips per strip 3. Resistance in friction of one strip against soil (LRFD Equation 11.10.6.3.2-1) 1) using L for static case e P = F*  Le C b = 1.138 kips v P = 0.9 × 1.138 = 1.025 kips 2) using L for static + dynamic case P = F*  L C b = 1.483 kips v P = 0.9 × 1.483 = 1.334 kips a) F* Kr = 2.000 at 0 ft Kr = tan  = 0.675 under 20 ft f Use interpolation at other depth F* = 1.837 (LRFD Figure 11.10.6.3.2-1) b)  = 1 (LRFD Table 11.10.6.3.2-1) c)  = 0.125 (kcf) × 2.46 (ft) = 0.3075 ksf v d) L= L-H/3 = 8 - 0.3 × 6.19 = 6.143 ft e (LRFD Figure 11.10.2-1 and 11.10.10.1-2) e) C = 2 for stip (LRFD 11.10.6.3.2) f) b = 0.164 ft 4. Location of Maximum Tensile Force (LRFD Figure 11.10.10.1-2) If the height of reinforcement layer is above the H/2, the location of max. tensile force is located in 0.3H. 0.3H = 1.857 ft H/2 = 3.095 ft L = 1.857 ft max. A-5 2.1.3 Second strip at h2= 4.92 ft h1 = 4.920 ft Kr = 0.446 1. Vertical stress 1) Reinforced Soil  =  × H V1 soil V1 = 0.125 (kcf) × 4.920 (ft) = 0.615 kips/ft2 EV × V1 = 1.35 ×  = 0.830 kips/ft2 2) Traffic surcharge  = 0.25 ksf V2 EV × V2 = 1.75 × 0.25 = 0.438 kips/ft2 a) ignoring tracffic surcharge b) including tracffic surcharge ∑v = 0.615 kips/ft2 ∑v = 0.865 kips/ft2 ∑EVv = 0.830 kips/ft2 ∑EVv = 1.268 kips/ft2 Horizontal stress,  =  ( k +  ) (LRFD Eq. 11.10.6.2.1-1) H P v r H a) ignoring tracffic surcharge  =  k = 0.615 ksf × 0.446 = 0.274 ksf h v r   =   k = 0.830 ksf × 0.446 = 0.370 ksf EV h EV v r At per strip = 4.870 (ft) × 2.460 (ft) / 3 = 3.993 ft2 depth for A at the second layer = S = 2.460 ft t v Tmax = H Sv = 0.274 ksf × 3.993 ft2 = 1.095 kips per strip EV Tmax = EV H Sv = 0.370 ksf × 3.993 ft2 = 1.478 kips per strip b) including tracffic surcharge  =  k = 0.865 ksf × 0.446 = 0.386 ksf h v r   =   k = 1.268 ksf × 0.446 = 0.565 ksf EV h EV v r Tmax = H Sv = 0.386 ksf × 3.993 ft2 = 1.54 kips per strip EV Tmax = EV H Sv = 0.565 ksf × 3.993 ft2 = 2.26 kips per strip 3. Resistance in friction of one strip against soil (LRFD Equation 11.10.6.3.2-1) 1) using L for static case e P = F*  Le C b = 2.445 kips v P = 0.9 × 2.44 = 2.200 kips A-6 2) using L for static + dynamic case P = F*  L C b = 2.702 kips v P = 0.9 × 2.702 = 2.432 kips a) F* Kr = 2.000 at 0 ft Kr = tan  = 0.675 under 20 ft f Use interpolation at other depth F* = 1.674 (LRFD Figure 11.10.6.3.2-1) b)  = 1 (LRFD Table 11.10.6.3.2-1) c)  = 0.125 (kcf) × 4.920 (ft) = 0.615 ksf v d) L= 7.238 ft (LRFD Figure 11.10.2-1 and 11.10.10.1-2) e e) C = 2 for stip (LRFD 11.10.6.3.2) f) b = 0.164 ft 4. Location of Maximum Tensile Force (LRFD Figure 11.10.10.1-2) If the height of reinforcement layer is above the H/2, the location of max. tensile force is located in 0.3H. 0.3H = 1.857 ft H/2 = 3.095 ft L = 0.762 ft max. 2.1.4 Reinforcement Tensile Strength 1) 75.00 years Design Life R= f × A = f × (Strip width × E ) y steel y c = 60.00 ksi × ( 1.969 in. × 0.102 ) in. = 12.016 kips  R = 0.75 × 12.016 = 9.012 kips 2) 100.00 years Design Life R= f × A = f × (Strip width × E ) y steel y c = 60.00 ksi × ( 1.969 in. × 0.078 ) in. = 9.226 kips  R = 0.75 × 9.226 = 6.919 kips For corrosion Losses E = E - E (LRFD Eq. 11.10.6.4.2a-1) c n s Zinc Coating Lift = 16 years Loass of carbon steel = 0.012 mm/yr. after zinc deplection 1) 75.00 years Design Life E = 4.00 mm - 1.416 mm = 2.584 mm = 0.102 in. c 2) 100.00 years Design Life E = 4.00 mm - 2.016 mm = 1.984 mm = 0.078 in. c 2.1.5 Summary 1) Pullout - ignoring traffic surcharge Rein. Layer Z T T P P NO. (ft) (kips) (kips) (kips) (kips) 1 2.46 0.569 0.768 1.138 1.025 2 4.92 1.095 1.478 2.445 2.200 A-7 2) Tensile - ignoring traffic surcharge 75 year Design Life 100 year Design Life Rein. Layer Z T T R R R R NO. (ft) (kips) (kips) (kips) (kips) (kips) (kips) 1 2.46 0.569 0.768 12.016 9.012 9.226 6.919 2 4.92 1.095 1.478 2.2 Including Impact Load load Br (length of s f 45+(/2) 45+(/2) tan(45+(/2)) Cr l1 (kips) (ft) (degrees) (degrees) radian (ft) (ft) 10 4.50 34 62 1.082 1.881 0.000 8.463 2.2.1 Tensile stress 5 ft.-> ∑F = 2 kpf Rein. Layer Layer l1  A T T hmax t impact impact NO. (ft) (ft) (ksf) (ft2) (kips) (kips) bottom of sla 1.670 8.463 0.473 1 2.460 7.673 0.429 3.993 1.711 1.711 2 4.920 5.213 0.291 3.993 1.163 1.163 * Summary of Total 75 year 100 year Rein. Layer Z T T Total T R R impact NO. (ft) (kips) (kips) (kips) (kips) (kips) 1 2.46 0.569 1.711 2.280 12.016 9.226 2 4.92 1.095 1.163 2.257 12.016 9.226 A-8 2.2.2 Pullout stress 20 ft.-> ∑F = 0.5 kpf Rein. Layer Layer l1  A T T hmax t impact impact NO. (ft) (ft) (ksf) (ft2) (kips) (kips) bottom of sla 1.670 8.463 0.118 1 2.460 7.673 0.107 3.993 0.428 0.428 2 4.920 5.213 0.073 3.993 0.291 0.291 * Summary of Total Rein. Layer Z T T Total T P impact NO. (ft) (kips) (kips) (kips) (kips) 1 2.46 0.569 0.428 0.997 1.483 2 4.92 1.095 0.291 1.386 2.702 A-9 2. 5-ft high MSE wall with 16-ft long strips design Wall Wall height, H = 6.190 ft 1/2 H= 3.095 ft Reinforcing fill length, L = 16.000 ft Length of slab = 4.500 ft B = 16.458 ft D = 6.800 mm C = 90.667 60 u Soil unit weight,  = 0.125 kcf D = 0.075 mm log C = 1.957 soil 10 u Traffic surcharge, q = 0.25 ksf Reinforcement fill,  = 34 degrees -> 0.593 radians (LRFD 11.10.6.2) -> tan = 0.675 -> Ka = 0.283 Retained fill,  = 30 degrees -> 0.524 radians -> tan = 0.577 -> Kaf = 0.333 f Static load = 10 kips Panel First strip location = 2.460 ft Strip width = 1.969 in. = 0.164 ft Location of slab bottom = 1.670 ft Strip thickness = 4 mm = 0.013 ft Vertical spacing of strips,S = 2.460 ft Horizontal spacing of strip= 2.435 ft v Panel width = 4.870 ft Steel Reinforcement Strength f = 60 ksi y Panel height = 4.854 ft density of strip per panel = 4.000 Panel thickness = 0.458 ft Load Factor, (LRFD 11.5.5) 1. Typical application 1.a. Bearing Resistance 1.b. Sliding and Eccentricity  = 1.35  = 1.5  = 1  = 1.5 EV EH EV EH 2. Live Load Surcharge on MSE wall 2.a. Bearing and reinforcement tensile resistnace  = 1.75 LS 2.b. Sliding, eccentricity and reinforcement pullout resistance  = 1.75 LS (LRFD Figure C11.5.5-3(b)) A-10

Description:
Live Load Surcharge on MSE wall. 2.a. Bearing and reinforcement tensile resistnace. γLS = 1.75. 2.b. Sliding, eccentricity and reinforcement pullout
ebook img

appendix a: design of mse wall PDF

100 Pages·2010·13.1 MB·English
by  
Save to my drive
Quick download
Download

Download appendix a: design of mse wall PDF Free - Full Version

by Unknow| 2010| 100 pages| 13.1| English

Download appendix a: design of mse wall by in PDF format completely FREE. No registration required, no payment needed. Get instant access to this valuable resource on PDFdrive.to!

Free Download PDF

About appendix a: design of mse wall

Live Load Surcharge on MSE wall. 2.a. Bearing and reinforcement tensile resistnace. γLS = 1.75. 2.b. Sliding, eccentricity and reinforcement pullout

Detailed Information

Author:Unknown
Publication Year:2010
Pages:100
Language:English
File Size:13.1
Format:PDF
Price:FREE
Download Free PDF

Safe & Secure Download - No registration required

Why Choose PDFdrive for Your Free appendix a: design of mse wall Download?

  • 100% Free: No hidden fees or subscriptions required for one book every day.
  • No Registration: Immediate access is available without creating accounts for one book every day.
  • Safe and Secure: Clean downloads without malware or viruses
  • Multiple Formats: PDF, MOBI, Mpub,... optimized for all devices
  • Educational Resource: Supporting knowledge sharing and learning

Free Books Similar to appendix a: design of mse wall

Frequently Asked Questions

Is it really free to download appendix a: design of mse wall PDF?

Yes, on https://PDFdrive.to you can download appendix a: design of mse wall by completely free. We don't require any payment, subscription, or registration to access this PDF file. For 3 books every day.

How can I read appendix a: design of mse wall on my mobile device?

After downloading appendix a: design of mse wall PDF, you can open it with any PDF reader app on your phone or tablet. We recommend using Adobe Acrobat Reader, Apple Books, or Google Play Books for the best reading experience.

Is this the full version of appendix a: design of mse wall?

Yes, this is the complete PDF version of appendix a: design of mse wall by Unknow. You will be able to read the entire content as in the printed version without missing any pages.

Is it legal to download appendix a: design of mse wall PDF for free?

https://PDFdrive.to provides links to free educational resources available online. We do not store any files on our servers. Please be aware of copyright laws in your country before downloading.

The materials shared are intended for research, educational, and personal use in accordance with fair use principles.

We use cookies

We use cookies to ensure you get the best browsing experience on our website. By clicking "Accept Cookies", you agree that we can store cookies on your device in accordance with our Terms and Privacy.